Question Number 36722 by Tinkutara last updated on 04/Jun/18
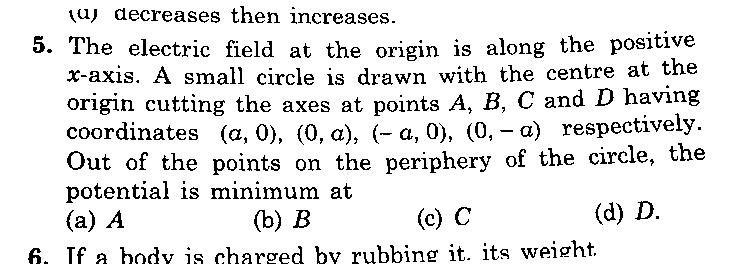
Answered by tanmay.chaudhury50@gmail.com last updated on 06/Jun/18
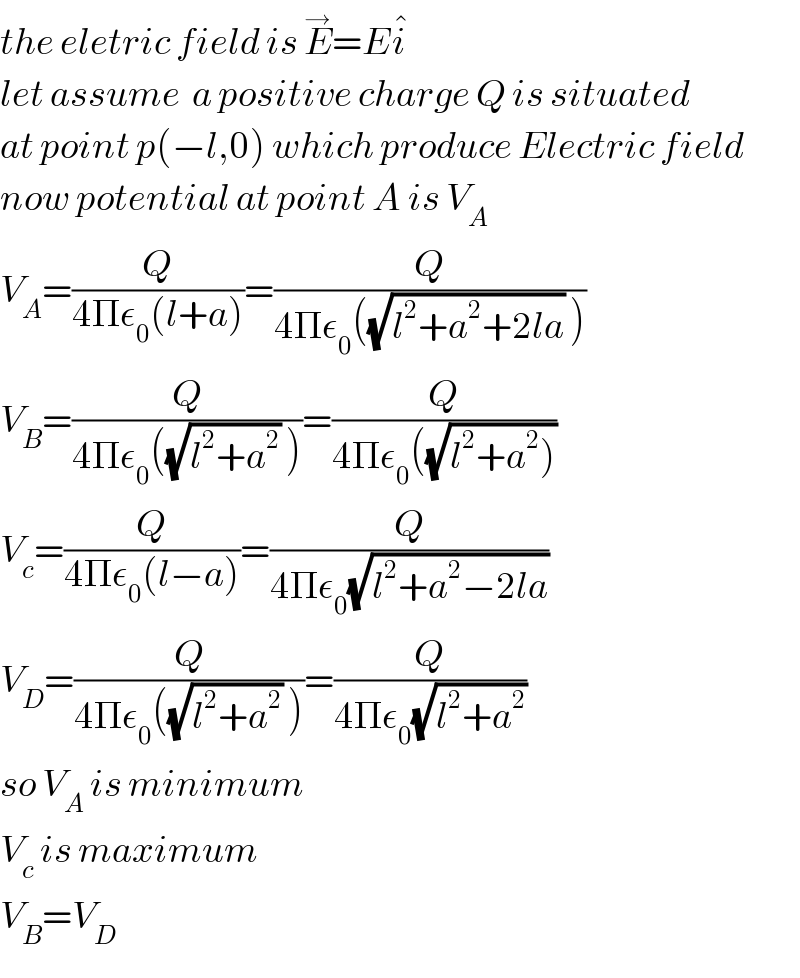
$${the}\:{eletric}\:{field}\:{is}\:\overset{\rightarrow} {{E}}={E}\hat {{i}} \\ $$$${let}\:{assume}\:\:{a}\:{positive}\:{charge}\:{Q}\:{is}\:{situated} \\ $$$${at}\:{point}\:{p}\left(−{l},\mathrm{0}\right)\:{which}\:{produce}\:{Electric}\:{field} \\ $$$${now}\:{potential}\:{at}\:{point}\:{A}\:{is}\:{V}_{{A}} \\ $$$${V}_{{A}} =\frac{{Q}}{\mathrm{4}\Pi\epsilon_{\mathrm{0}} \left({l}+{a}\right)}=\frac{{Q}}{\mathrm{4}\Pi\epsilon_{\mathrm{0}} \left(\sqrt{{l}^{\mathrm{2}} +{a}^{\mathrm{2}} +\mathrm{2}{la}}\:\right)} \\ $$$${V}_{{B}} =\frac{{Q}}{\mathrm{4}\Pi\epsilon_{\mathrm{0}} \left(\sqrt{{l}^{\mathrm{2}} +{a}^{\mathrm{2}} }\:\right)}=\frac{{Q}}{\mathrm{4}\Pi\epsilon_{\mathrm{0}} \left(\sqrt{\left.{l}^{\mathrm{2}} +{a}^{\mathrm{2}} \right)}\right.} \\ $$$${V}_{{c}} =\frac{{Q}}{\mathrm{4}\Pi\epsilon_{\mathrm{0}} \left({l}−{a}\right)}=\frac{{Q}}{\mathrm{4}\Pi\epsilon_{\mathrm{0}} \sqrt{{l}^{\mathrm{2}} +{a}^{\mathrm{2}} −\mathrm{2}{la}}} \\ $$$${V}_{{D}} =\frac{{Q}}{\mathrm{4}\Pi\epsilon_{\mathrm{0}} \left(\sqrt{{l}^{\mathrm{2}} +{a}^{\mathrm{2}} }\:\right)}=\frac{{Q}}{\mathrm{4}\Pi\epsilon_{\mathrm{0}} \sqrt{{l}^{\mathrm{2}} +{a}^{\mathrm{2}} }}\: \\ $$$${so}\:{V}_{{A}} \:{is}\:{minimum} \\ $$$${V}_{{c}} \:{is}\:{maximum} \\ $$$${V}_{{B}} ={V}_{{D}} \\ $$
Commented by Tinkutara last updated on 06/Jun/18
Thank you very much Sir! I got the answer. ��������
