Question Number 36880 by Tinkutara last updated on 06/Jun/18

Commented by ajfour last updated on 07/Jun/18
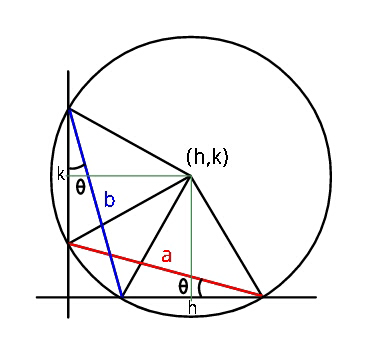
Commented by ajfour last updated on 07/Jun/18

$$\mathrm{2}{h}={a}\mathrm{cos}\:\theta+{b}\mathrm{sin}\:\theta \\ $$$$\mathrm{2}{k}={b}\mathrm{cos}\:\theta+{a}\mathrm{sin}\:\theta \\ $$$$\Rightarrow\:\mathrm{cos}\:\theta=\frac{\mathrm{2}\left({ah}−{bk}\right)}{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} } \\ $$$$\:\:\:\:\:\mathrm{sin}\:\theta=\frac{\mathrm{2}\left({bh}−{ak}\right)}{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} } \\ $$$${so}\:{locus}\:{eq}.\:{is} \\ $$$$\left({ax}−{by}\right)^{\mathrm{2}} +\left({bx}−{ay}\right)^{\mathrm{2}} =\frac{\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)^{\mathrm{2}} }{\mathrm{4}}\:. \\ $$$${doesn}'{t}\:{match}\:{with}\:{options}..?! \\ $$
Commented by Tinkutara last updated on 08/Jun/18
Sir please explain first 2 lines.
Answered by tanmay.chaudhury50@gmail.com last updated on 06/Jun/18
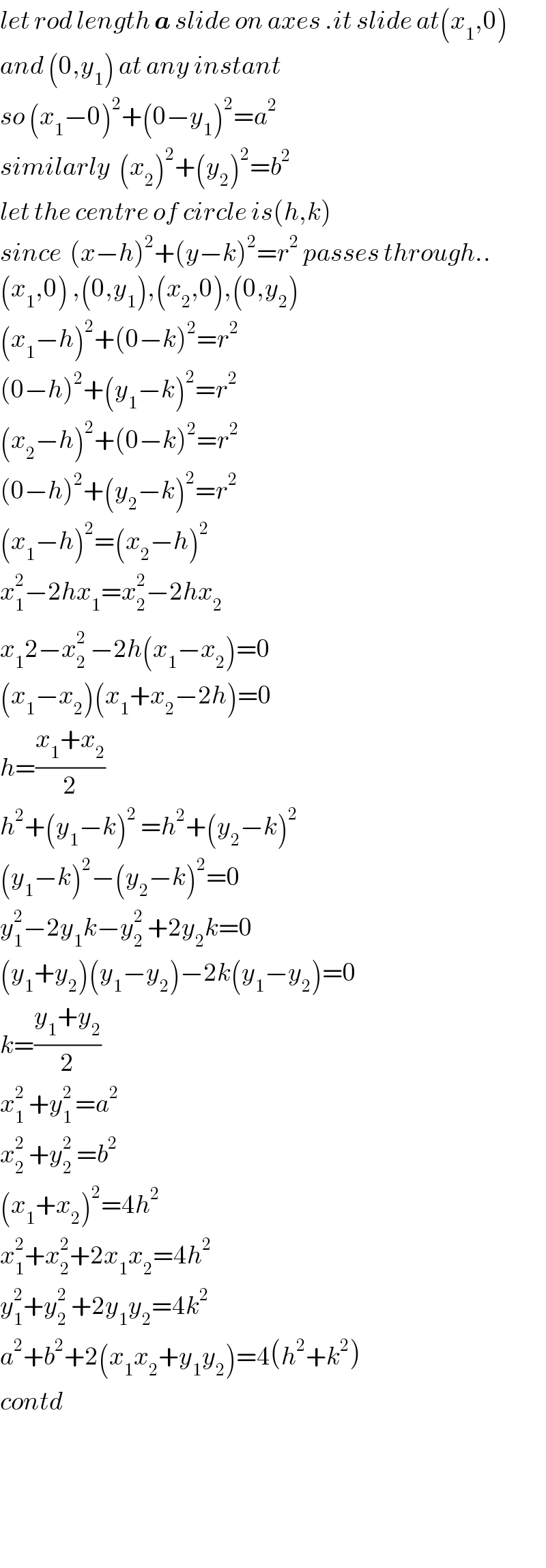
$${let}\:{rod}\:{length}\:\boldsymbol{{a}}\:{slide}\:{on}\:{axes}\:.{it}\:{slide}\:{at}\left({x}_{\mathrm{1}} ,\mathrm{0}\right)\: \\ $$$${and}\:\left(\mathrm{0},{y}_{\mathrm{1}} \right)\:{at}\:{any}\:{instant} \\ $$$${so}\:\left({x}_{\mathrm{1}} −\mathrm{0}\right)^{\mathrm{2}} +\left(\mathrm{0}−{y}_{\mathrm{1}} \right)^{\mathrm{2}} ={a}^{\mathrm{2}} \\ $$$${similarly}\:\:\left({x}_{\mathrm{2}} \right)^{\mathrm{2}} +\left({y}_{\mathrm{2}} \right)^{\mathrm{2}} ={b}^{\mathrm{2}} \\ $$$${let}\:{the}\:{centre}\:{of}\:{circle}\:{is}\left({h},{k}\right) \\ $$$${since}\:\:\left({x}−{h}\right)^{\mathrm{2}} +\left({y}−{k}\right)^{\mathrm{2}} ={r}^{\mathrm{2}} \:{passes}\:{through}..^{} \\ $$$$\left({x}_{\mathrm{1}} ,\mathrm{0}\right)\:,\left(\mathrm{0},{y}_{\mathrm{1}} \right),\left({x}_{\mathrm{2}} ,\mathrm{0}\right),\left(\mathrm{0},{y}_{\mathrm{2}} \right) \\ $$$$\left({x}_{\mathrm{1}} −{h}\right)^{\mathrm{2}} +\left(\mathrm{0}−{k}\right)^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$$\left(\mathrm{0}−{h}\right)^{\mathrm{2}} +\left({y}_{\mathrm{1}} −{k}\right)^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$$\left({x}_{\mathrm{2}} −{h}\right)^{\mathrm{2}} +\left(\mathrm{0}−{k}\right)^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$$\left(\mathrm{0}−{h}\right)^{\mathrm{2}} +\left({y}_{\mathrm{2}} −{k}\right)^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$$\left({x}_{\mathrm{1}} −{h}\right)^{\mathrm{2}} =\left({x}_{\mathrm{2}} −{h}\right)^{\mathrm{2}} \\ $$$${x}_{\mathrm{1}} ^{\mathrm{2}} −\mathrm{2}{hx}_{\mathrm{1}} ={x}_{\mathrm{2}} ^{\mathrm{2}} −\mathrm{2}{hx}_{\mathrm{2}} \\ $$$${x}_{\mathrm{1}} \mathrm{2}−{x}_{\mathrm{2}} ^{\mathrm{2}} \:−\mathrm{2}{h}\left({x}_{\mathrm{1}} −{x}_{\mathrm{2}} \overset{} {\right)}=\mathrm{0} \\ $$$$\left({x}_{\mathrm{1}} −{x}_{\mathrm{2}} \right)\left({x}_{\mathrm{1}} +{x}_{\mathrm{2}} −\mathrm{2}{h}\right)=\mathrm{0} \\ $$$${h}=\frac{{x}_{\mathrm{1}} +{x}_{\mathrm{2}} }{\mathrm{2}} \\ $$$${h}^{\mathrm{2}} +\left({y}_{\mathrm{1}} −{k}\right)^{\mathrm{2}} \:={h}^{\mathrm{2}} +\left({y}_{\mathrm{2}} −{k}\right)^{\mathrm{2}\:} \\ $$$$\left({y}_{\mathrm{1}} −{k}\right)^{\mathrm{2}} −\left({y}_{\mathrm{2}} −{k}\right)^{\mathrm{2}} =\mathrm{0} \\ $$$${y}_{\mathrm{1}} ^{\mathrm{2}} −\mathrm{2}{y}_{\mathrm{1}} {k}−{y}_{\mathrm{2}} ^{\mathrm{2}} \:+\mathrm{2}{y}_{\mathrm{2}} {k}=\mathrm{0} \\ $$$$\left({y}_{\mathrm{1}} +{y}_{\mathrm{2}} \right)\left({y}_{\mathrm{1}} −{y}_{\mathrm{2}} \right)−\mathrm{2}{k}\left({y}_{\mathrm{1}} −{y}_{\mathrm{2}} \right)=\mathrm{0} \\ $$$${k}=\frac{{y}_{\mathrm{1}} +{y}_{\mathrm{2}} }{\mathrm{2}} \\ $$$${x}_{\mathrm{1}} ^{\mathrm{2}} \:+{y}_{\mathrm{1}} ^{\mathrm{2}\:} ={a}^{\mathrm{2}} \\ $$$${x}_{\mathrm{2}} ^{\mathrm{2}} \:+{y}_{\mathrm{2}} ^{\mathrm{2}} \:={b}^{\mathrm{2}} \\ $$$$\left({x}_{\mathrm{1}} +{x}_{\mathrm{2}} \right)^{\mathrm{2}} =\mathrm{4}{h}^{\mathrm{2}} \\ $$$${x}_{\mathrm{1}} ^{\mathrm{2}} +{x}_{\mathrm{2}} ^{\mathrm{2}} +\mathrm{2}{x}_{\mathrm{1}} {x}_{\mathrm{2}} =\mathrm{4}{h}^{\mathrm{2}} \\ $$$${y}_{\mathrm{1}} ^{\mathrm{2}} +{y}_{\mathrm{2}} ^{\mathrm{2}} \:+\mathrm{2}{y}_{\mathrm{1}} {y}_{\mathrm{2}} =\mathrm{4}{k}^{\mathrm{2}} \: \\ $$$${a}^{\mathrm{2}} +{b}^{\mathrm{2}} +\mathrm{2}\left({x}_{\mathrm{1}} {x}_{\mathrm{2}} +{y}_{\mathrm{1}} {y}_{\mathrm{2}} \right)=\mathrm{4}\left({h}^{\mathrm{2}} +{k}^{\mathrm{2}} \right) \\ $$$${contd} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
