Question Number 38618 by tawa tawa last updated on 27/Jun/18

Commented by MrW3 last updated on 28/Jun/18
![[1−(1/2)(1−x)]^4 =1−4x+px^2 +qx^3 +... this can not be true. ⇒something is wrong with the question.](https://www.tinkutara.com/question/Q38649.png)
$$\left[\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}−{x}\right)\right]^{\mathrm{4}} =\mathrm{1}−\mathrm{4}{x}+{px}^{\mathrm{2}} +{qx}^{\mathrm{3}} +… \\ $$$${this}\:{can}\:{not}\:{be}\:{true}. \\ $$$$\Rightarrow{something}\:{is}\:{wrong}\:{with}\:{the}\:{question}. \\ $$
Commented by tawa tawa last updated on 28/Jun/18
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
Answered by Rio Mike last updated on 28/Jun/18
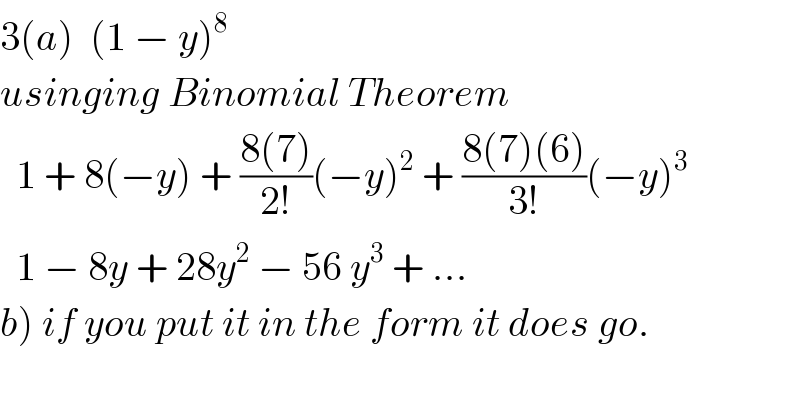
$$\mathrm{3}\left({a}\right)\:\:\left(\mathrm{1}\:−\:{y}\right)^{\mathrm{8}} \: \\ $$$${usinging}\:{Binomial}\:{Theorem} \\ $$$$\:\:\mathrm{1}\:+\:\mathrm{8}\left(−{y}\right)\:+\:\frac{\mathrm{8}\left(\mathrm{7}\right)}{\mathrm{2}!}\left(−{y}\right)^{\mathrm{2}} \:+\:\frac{\mathrm{8}\left(\mathrm{7}\right)\left(\mathrm{6}\right)}{\mathrm{3}!}\left(−{y}\right)^{\mathrm{3}} \\ $$$$\:\:\mathrm{1}\:−\:\mathrm{8}{y}\:+\:\mathrm{28}{y}^{\mathrm{2}} \:−\:\mathrm{56}\:{y}^{\mathrm{3}} \:+\:… \\ $$$$\left.{b}\right)\:{if}\:{you}\:{put}\:{it}\:{in}\:{the}\:{form}\:{it}\:{does}\:{go}. \\ $$$$ \\ $$
Commented by tawa tawa last updated on 01/Jul/18

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
