Question Number 38762 by Raj Singh last updated on 29/Jun/18

Commented by tanmay.chaudhury50@gmail.com last updated on 29/Jun/18

Commented by abdo.msup.com last updated on 29/Jun/18
![we have f(x)=arctan(cosx +sinx) =arctan((√2)cos(x−(π/4)))⇒ f^′ (x)= ((−(√2)sin(x−(π/4)))/(1+2 cos^2 (x−(π/4)))) =(√2)sin((π/4)−x) we have 0≤x≤(π/4) ⇒−(π/4)≤−x≤0 ⇒ 0≤(π/4) −x≤(π/4) ⇒(√2)sin((π/4)−x)≥0 ⇒ f^′ (x)≥0 ⇒ f is increasing on[0,(π/4)].](https://www.tinkutara.com/question/Q38797.png)
$${we}\:{have}\:{f}\left({x}\right)={arctan}\left({cosx}\:+{sinx}\right) \\ $$$$={arctan}\left(\sqrt{\mathrm{2}}{cos}\left({x}−\frac{\pi}{\mathrm{4}}\right)\right)\Rightarrow \\ $$$${f}^{'} \left({x}\right)=\:\frac{−\sqrt{\mathrm{2}}{sin}\left({x}−\frac{\pi}{\mathrm{4}}\right)}{\mathrm{1}+\mathrm{2}\:{cos}^{\mathrm{2}} \left({x}−\frac{\pi}{\mathrm{4}}\right)}\:=\sqrt{\mathrm{2}}{sin}\left(\frac{\pi}{\mathrm{4}}−{x}\right) \\ $$$${we}\:{have}\:\mathrm{0}\leqslant{x}\leqslant\frac{\pi}{\mathrm{4}}\:\Rightarrow−\frac{\pi}{\mathrm{4}}\leqslant−{x}\leqslant\mathrm{0}\:\Rightarrow \\ $$$$\mathrm{0}\leqslant\frac{\pi}{\mathrm{4}}\:−{x}\leqslant\frac{\pi}{\mathrm{4}}\:\Rightarrow\sqrt{\mathrm{2}}{sin}\left(\frac{\pi}{\mathrm{4}}−{x}\right)\geqslant\mathrm{0}\:\Rightarrow \\ $$$${f}^{'} \left({x}\right)\geqslant\mathrm{0}\:\Rightarrow\:{f}\:{is}\:{increasing}\:{on}\left[\mathrm{0},\frac{\pi}{\mathrm{4}}\right]. \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 29/Jun/18
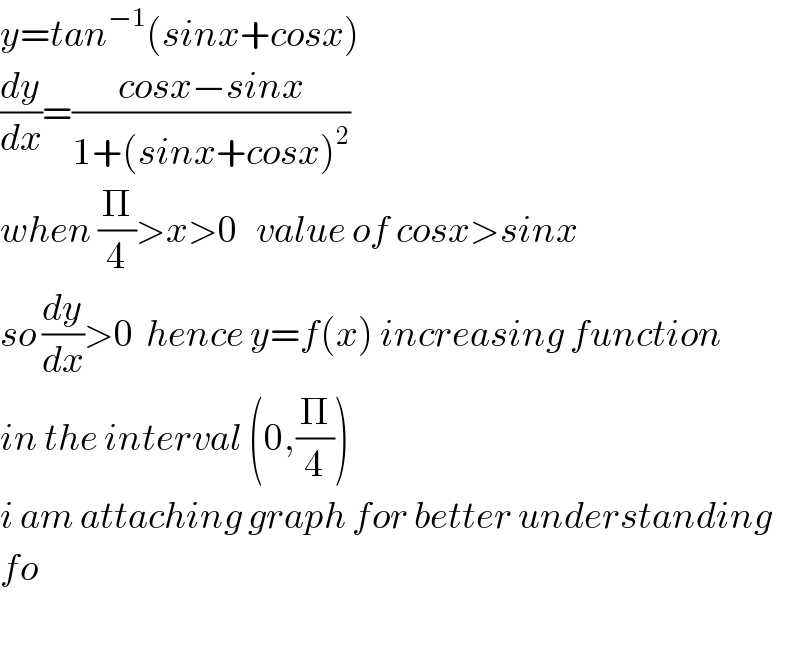
$${y}={tan}^{−\mathrm{1}} \left({sinx}+{cosx}\right) \\ $$$$\frac{{dy}}{{dx}}=\frac{{cosx}−{sinx}}{\mathrm{1}+\left({sinx}+{cosx}\right)^{\mathrm{2}} } \\ $$$${when}\:\frac{\Pi}{\mathrm{4}}>{x}>\mathrm{0}\:\:\:{value}\:{of}\:{cosx}>{sinx} \\ $$$${so}\:\frac{{dy}}{{dx}}>\mathrm{0}\:\:{hence}\:{y}={f}\left({x}\right)\:{increasing}\:{function} \\ $$$${in}\:{the}\:{interval}\:\left(\mathrm{0},\frac{\Pi}{\mathrm{4}}\right) \\ $$$${i}\:{am}\:{attaching}\:{graph}\:{for}\:{better}\:{understanding} \\ $$$${fo} \\ $$$$ \\ $$
Answered by MJS last updated on 29/Jun/18
![sin x +cos x =(√2)sin(x+(π/4)) f(x)=arctan((√2)sin(x+(π/4))) (d/dx)[arctan f(x)]=((f′(x))/(f(x)^2 +1))= =(((√2)cos(x+(π/4)))/(2sin^2 (x+(π/4))+1)) (2sin^2 (x+(π/4))+1)>0 ∀x∈R (√2)cos(x+(π/4))=0 ⇒ x=zπ−(3/4)π; z∈Z ⇒ ⇒ (√2)cos(x+(π/4))>0 ∀x∈]−(3/4)π; (π/4)[ ⇒ ⇒ f(x) is increasing for x∈]−(3/4)π; (π/4)[ ⇒ ⇒ f(x) is increasing for x∈]0; (π/4)[](https://www.tinkutara.com/question/Q38796.png)
$$\mathrm{sin}\:{x}\:+\mathrm{cos}\:{x}\:=\sqrt{\mathrm{2}}\mathrm{sin}\left({x}+\frac{\pi}{\mathrm{4}}\right) \\ $$$${f}\left({x}\right)={arctan}\left(\sqrt{\mathrm{2}}\mathrm{sin}\left({x}+\frac{\pi}{\mathrm{4}}\right)\right) \\ $$$$\frac{{d}}{{dx}}\left[\mathrm{arctan}\:{f}\left({x}\right)\right]=\frac{{f}'\left({x}\right)}{{f}\left({x}\right)^{\mathrm{2}} +\mathrm{1}}= \\ $$$$=\frac{\sqrt{\mathrm{2}}\mathrm{cos}\left({x}+\frac{\pi}{\mathrm{4}}\right)}{\mathrm{2sin}^{\mathrm{2}} \left({x}+\frac{\pi}{\mathrm{4}}\right)+\mathrm{1}} \\ $$$$ \\ $$$$\left(\mathrm{2sin}^{\mathrm{2}} \left({x}+\frac{\pi}{\mathrm{4}}\right)+\mathrm{1}\right)>\mathrm{0}\:\forall{x}\in\mathbb{R} \\ $$$$\sqrt{\mathrm{2}}\mathrm{cos}\left({x}+\frac{\pi}{\mathrm{4}}\right)=\mathrm{0}\:\Rightarrow\:{x}={z}\pi−\frac{\mathrm{3}}{\mathrm{4}}\pi;\:{z}\in\mathbb{Z}\:\Rightarrow \\ $$$$\left.\Rightarrow\:\sqrt{\mathrm{2}}\mathrm{cos}\left({x}+\frac{\pi}{\mathrm{4}}\right)>\mathrm{0}\:\forall{x}\in\right]−\frac{\mathrm{3}}{\mathrm{4}}\pi;\:\frac{\pi}{\mathrm{4}}\left[\:\Rightarrow\right. \\ $$$$\left.\Rightarrow\:{f}\left({x}\right)\:\mathrm{is}\:\mathrm{increasing}\:\mathrm{for}\:{x}\in\right]−\frac{\mathrm{3}}{\mathrm{4}}\pi;\:\frac{\pi}{\mathrm{4}}\left[\:\Rightarrow\right. \\ $$$$\left.\Rightarrow\:{f}\left({x}\right)\:\mathrm{is}\:\mathrm{increasing}\:\mathrm{for}\:{x}\in\right]\mathrm{0};\:\frac{\pi}{\mathrm{4}}\left[\right. \\ $$$$ \\ $$
