Question Number 38936 by ajfour last updated on 01/Jul/18

Commented by ajfour last updated on 01/Jul/18
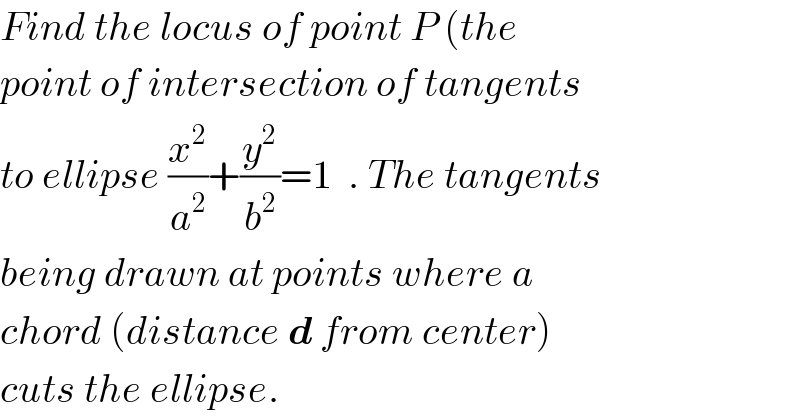
$${Find}\:{the}\:{locus}\:{of}\:{point}\:{P}\:\left({the}\right. \\ $$$${point}\:{of}\:{intersection}\:{of}\:{tangents} \\ $$$${to}\:{ellipse}\:\frac{{x}^{\mathrm{2}} }{{a}^{\mathrm{2}} }+\frac{{y}^{\mathrm{2}} }{{b}^{\mathrm{2}} }=\mathrm{1}\:\:.\:{The}\:{tangents} \\ $$$${being}\:{drawn}\:{at}\:{points}\:{where}\:{a} \\ $$$${chord}\:\left({distance}\:\boldsymbol{{d}}\:{from}\:{center}\right) \\ $$$${cuts}\:{the}\:{ellipse}. \\ $$
Answered by ajfour last updated on 01/Jul/18
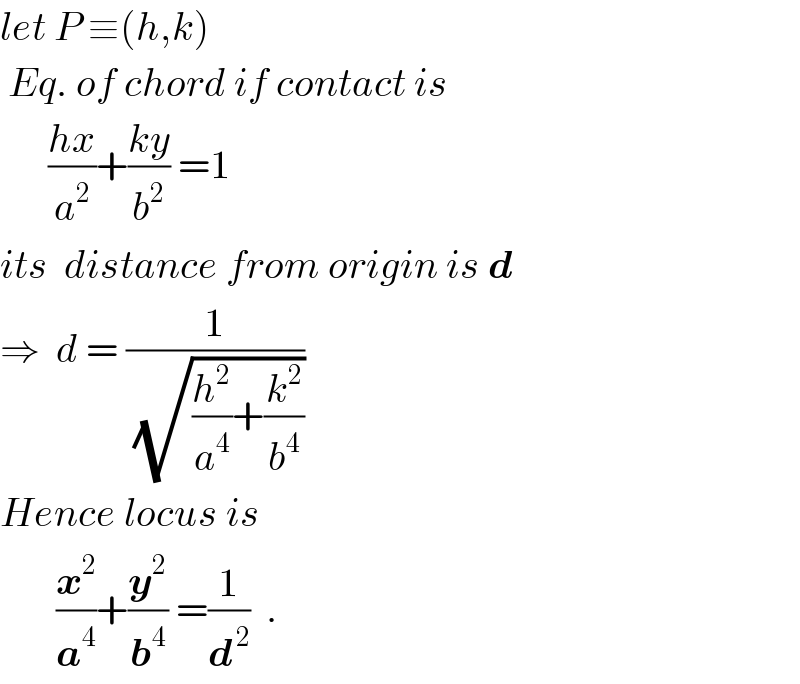
$${let}\:{P}\:\equiv\left({h},{k}\right) \\ $$$$\:{Eq}.\:{of}\:{chord}\:{if}\:{contact}\:{is} \\ $$$$\:\:\:\:\:\:\frac{{hx}}{{a}^{\mathrm{2}} }+\frac{{ky}}{{b}^{\mathrm{2}} }\:=\mathrm{1} \\ $$$${its}\:\:{distance}\:{from}\:{origin}\:{is}\:\boldsymbol{{d}} \\ $$$$\Rightarrow\:\:{d}\:=\:\frac{\mathrm{1}}{\:\sqrt{\frac{{h}^{\mathrm{2}} }{{a}^{\mathrm{4}} }+\frac{{k}^{\mathrm{2}} }{{b}^{\mathrm{4}} }}} \\ $$$${Hence}\:{locus}\:{is} \\ $$$$\:\:\:\:\:\:\:\frac{\boldsymbol{{x}}^{\mathrm{2}} }{\boldsymbol{{a}}^{\mathrm{4}} }+\frac{\boldsymbol{{y}}^{\mathrm{2}} }{\boldsymbol{{b}}^{\mathrm{4}} }\:=\frac{\mathrm{1}}{\boldsymbol{{d}}^{\:\mathrm{2}} }\:\:. \\ $$
