Question-38939

Question Number 38939 by rahul 19 last updated on 01/Jul/18

Commented by rahul 19 last updated on 01/Jul/18

Pls solve this problem by Nodal Method. Kirchhoff′s law is not allowed !

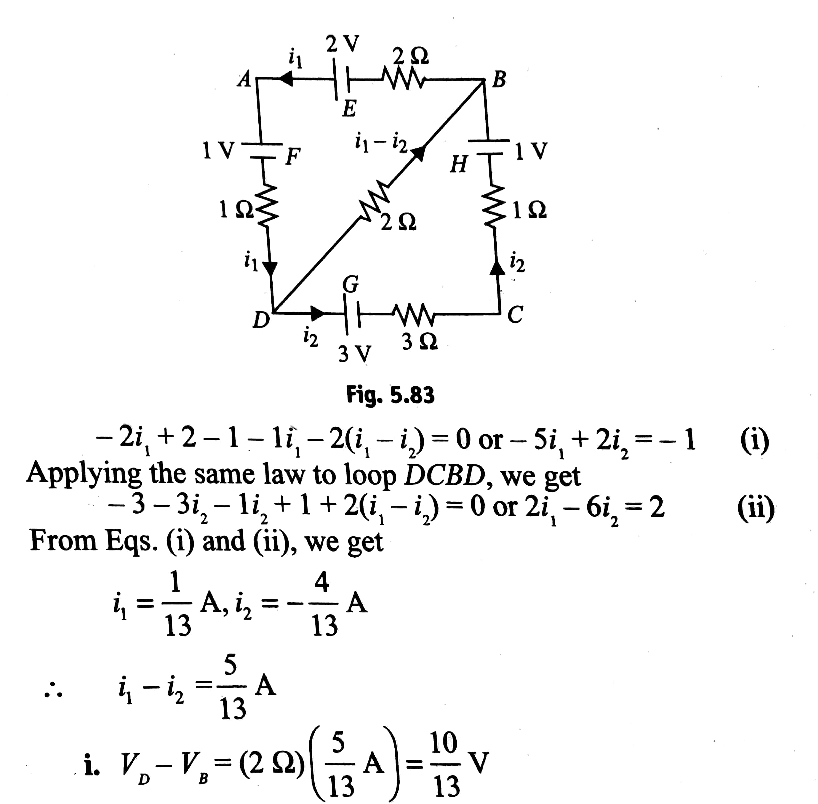
$$\mathrm{Pls}\:\mathrm{solve}\:\mathrm{this}\:\mathrm{problem}\:\mathrm{by}\:\mathrm{Nodal}\:\mathrm{Method}. \\ $$$$\mathrm{Kirchhoff}'\mathrm{s}\:\mathrm{law}\:\mathrm{is}\:\mathrm{not}\:\mathrm{allowed}\:! \\ $$

Commented by rahul 19 last updated on 01/Jul/18

Answered by tanmay.chaudhury50@gmail.com last updated on 01/Jul/18

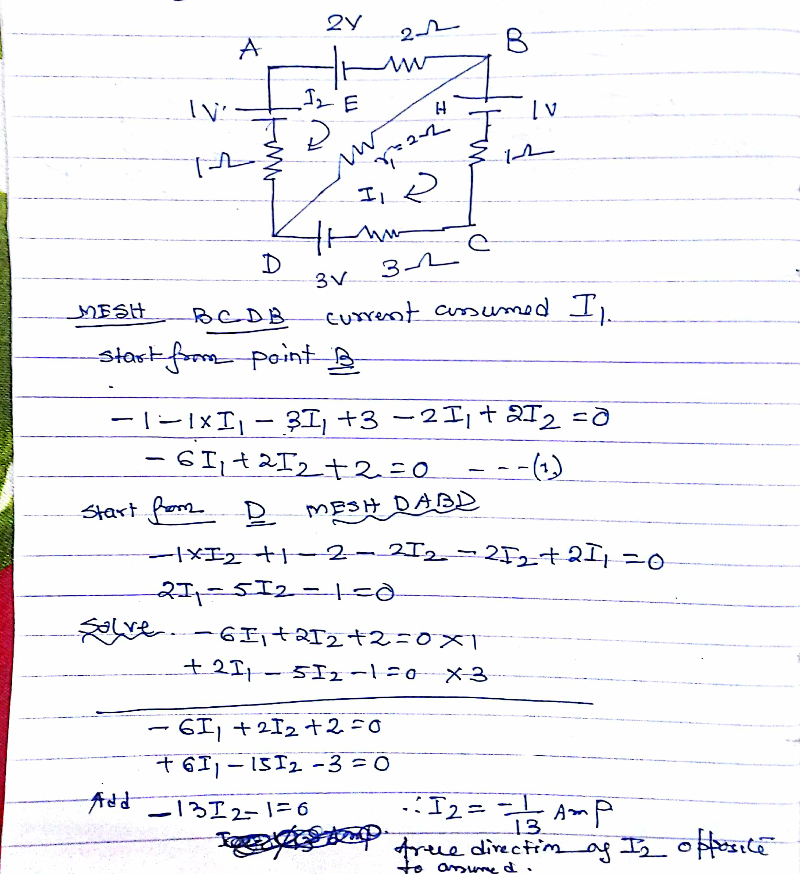
Commented by tanmay.chaudhury50@gmail.com last updated on 01/Jul/18

Commented by tanmay.chaudhury50@gmail.com last updated on 01/Jul/18

$${pls}\:{check}… \\ $$

Commented by rahul 19 last updated on 01/Jul/18

thank you sir ��

Commented by rahul 19 last updated on 01/Jul/18

pls check book sol. , is it correct ?

Commented by tanmay.chaudhury50@gmail.com last updated on 01/Jul/18

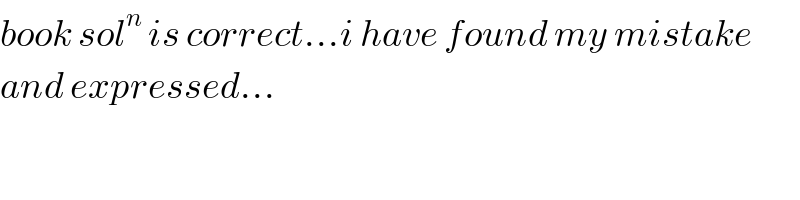
$${book}\:{sol}^{{n}} \:{is}\:{correct}…{i}\:{have}\:{found}\:{my}\:{mistake} \\ $$$${and}\:{expressed}… \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 01/Jul/18

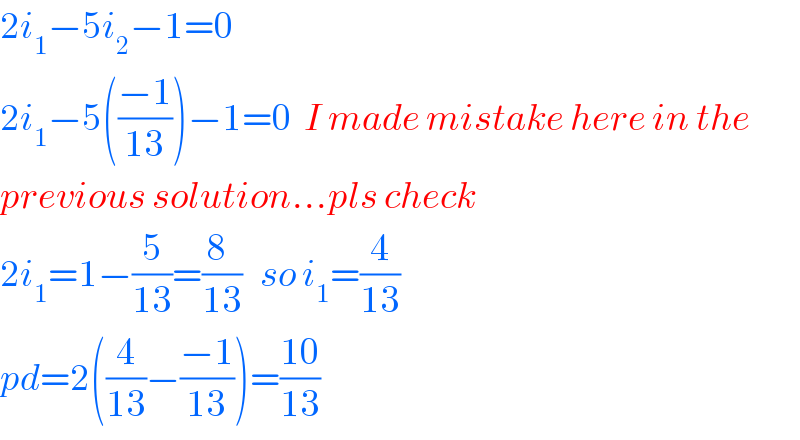
2i_1 −5i_2 −1=0 2i_1 −5(((−1)/(13)))−1=0 I made mistake here in the previous solution...pls check 2i_1 =1−(5/(13))=((8 )/(13)) so i_1 =(4/(13)) pd=2((4/(13))−((−1)/(13)))=((10)/(13))

$$\mathrm{2}{i}_{\mathrm{1}} −\mathrm{5}{i}_{\mathrm{2}} −\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{2}{i}_{\mathrm{1}} −\mathrm{5}\left(\frac{−\mathrm{1}}{\mathrm{13}}\right)−\mathrm{1}=\mathrm{0}\:\:{I}\:{made}\:{mistake}\:{here}\:{in}\:{the} \\ $$$${previous}\:{solution}…{pls}\:{check} \\ $$$$\mathrm{2}{i}_{\mathrm{1}} =\mathrm{1}−\frac{\mathrm{5}}{\mathrm{13}}=\frac{\mathrm{8}\:\:}{\mathrm{13}}\:\:\:{so}\:{i}_{\mathrm{1}} =\frac{\mathrm{4}}{\mathrm{13}} \\ $$$${pd}=\mathrm{2}\left(\frac{\mathrm{4}}{\mathrm{13}}−\frac{−\mathrm{1}}{\mathrm{13}}\right)=\frac{\mathrm{10}}{\mathrm{13}} \\ $$

Answered by ajfour last updated on 01/Jul/18

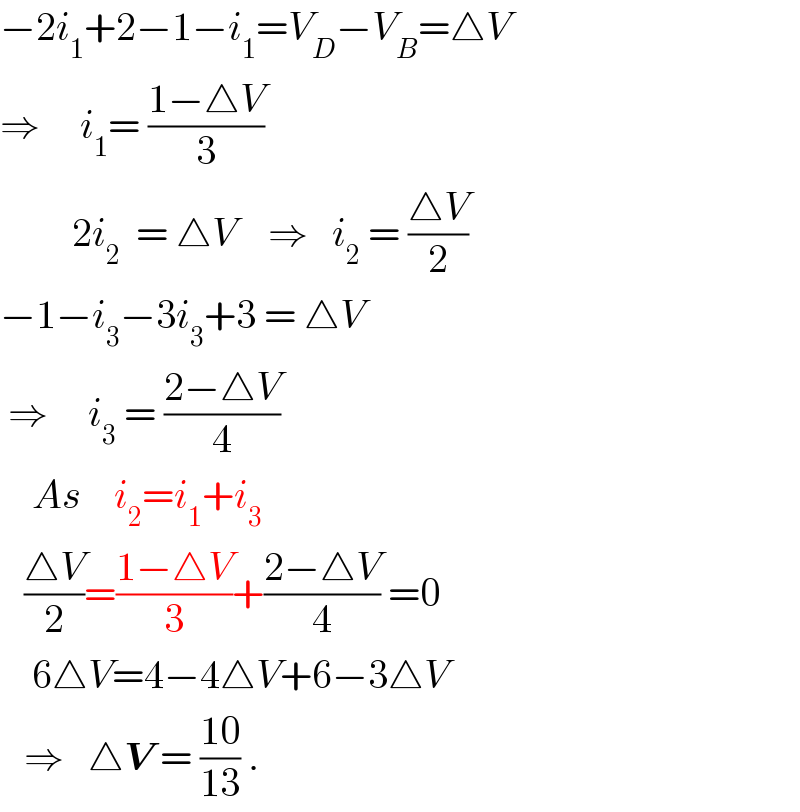
−2i_1 +2−1−i_1 =V_D −V_B =△V ⇒ i_1 = ((1−△V)/3) 2i_2 = △V ⇒ i_2 = ((△V)/2) −1−i_3 −3i_3 +3 = △V ⇒ i_3 = ((2−△V)/4) As i_2 =i_1 +i_3 ((△V)/2)=((1−△V)/3)+((2−△V)/4) =0 6△V=4−4△V+6−3△V ⇒ △V = ((10)/(13)) .

$$−\mathrm{2}{i}_{\mathrm{1}} +\mathrm{2}−\mathrm{1}−{i}_{\mathrm{1}} ={V}_{{D}} −{V}_{{B}} =\bigtriangleup{V} \\ $$$$\Rightarrow\:\:\:\:\:{i}_{\mathrm{1}} =\:\frac{\mathrm{1}−\bigtriangleup{V}}{\mathrm{3}} \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{2}{i}_{\mathrm{2}} \:\:=\:\bigtriangleup{V}\:\:\:\:\Rightarrow\:\:\:{i}_{\mathrm{2}} \:=\:\frac{\bigtriangleup{V}}{\mathrm{2}} \\ $$$$−\mathrm{1}−{i}_{\mathrm{3}} −\mathrm{3}{i}_{\mathrm{3}} +\mathrm{3}\:=\:\bigtriangleup{V} \\ $$$$\:\Rightarrow\:\:\:\:\:{i}_{\mathrm{3}} \:=\:\frac{\mathrm{2}−\bigtriangleup{V}}{\mathrm{4}} \\ $$$$\:\:\:\:{As}\:\:\:\:{i}_{\mathrm{2}} ={i}_{\mathrm{1}} +{i}_{\mathrm{3}} \:\: \\ $$$$\:\:\:\frac{\bigtriangleup{V}}{\mathrm{2}}=\frac{\mathrm{1}−\bigtriangleup{V}}{\mathrm{3}}+\frac{\mathrm{2}−\bigtriangleup{V}}{\mathrm{4}}\:=\mathrm{0} \\ $$$$\:\:\:\:\mathrm{6}\bigtriangleup{V}=\mathrm{4}−\mathrm{4}\bigtriangleup{V}+\mathrm{6}−\mathrm{3}\bigtriangleup{V} \\ $$$$\:\:\:\Rightarrow\:\:\:\bigtriangleup\boldsymbol{{V}}\:=\:\frac{\mathrm{10}}{\mathrm{13}}\:. \\ $$

Commented by rahul 19 last updated on 01/Jul/18

Thank You sir ��

Facebook Tweet Pin

Leave a Reply Cancel reply