Question Number 39300 by behi83417@gmail.com last updated on 05/Jul/18

Answered by MrW3 last updated on 05/Jul/18

$${a}\:{square}\:{can}\:{inscribe}\:{every}\:{triangle}. \\ $$$${so}\:{the}\:{question}\:{is} \\ $$$${if}\:{it}\:{is}\:{possible}\:{to}\:{build}\:{a}\:{triangle}\: \\ $$$${whose}\:{sides}\:{are}\:{three}\:{consecutive}\:{odd} \\ $$$$\left({or}\:{even}\right)\:{numbers}. \\ $$$${a}={n} \\ $$$${b}={n}+\mathrm{2} \\ $$$${c}={n}+\mathrm{4} \\ $$$${for}\:{this}\:{to}\:{be}\:{possible},\:{we}\:{must}\:{have} \\ $$$${a}+{b}>{c}\Rightarrow\mathrm{2}{n}+\mathrm{2}>{n}+\mathrm{4}\Rightarrow{n}>\mathrm{2} \\ $$$${b}+{c}>{a}\Rightarrow\mathrm{2}{n}+\mathrm{6}>{n}\Rightarrow{n}>−\mathrm{6} \\ $$$${c}+{a}>{b}\Rightarrow\mathrm{2}{n}+\mathrm{4}>{n}+\mathrm{2}\Rightarrow{n}>−\mathrm{2} \\ $$$${that}\:{means}\:{n}\geqslant\mathrm{3}. \\ $$$${so}\:{the}\:{answer}\:{is}\:{yes}. \\ $$$$ \\ $$$${if}\:{additionally}\:{the}\:{triangle}\:{should}\:{only} \\ $$$${have}\:{acute}\:{angles},\:{we}\:{must}\:{have} \\ $$$$\left({n}+\mathrm{4}\right)^{\mathrm{2}} \leqslant{n}^{\mathrm{2}} +\left({n}+\mathrm{2}\right)^{\mathrm{2}} \\ $$$${n}^{\mathrm{2}} +\mathrm{8}{n}+\mathrm{16}\leqslant{n}^{\mathrm{2}} +{n}^{\mathrm{2}} +\mathrm{4}{n}+\mathrm{4} \\ $$$${n}^{\mathrm{2}} −\mathrm{4}{n}−\mathrm{12}\geqslant\mathrm{0} \\ $$$$\left({n}+\mathrm{2}\right)\left({n}−\mathrm{6}\right)\geqslant\mathrm{0} \\ $$$${n}\geqslant\mathrm{6} \\ $$
Commented by behi83417@gmail.com last updated on 05/Jul/18
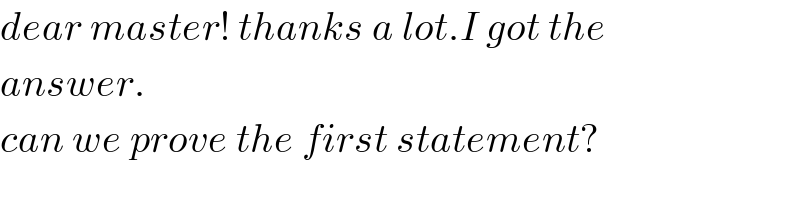
$${dear}\:{master}!\:{thanks}\:{a}\:{lot}.{I}\:{got}\:{the} \\ $$$${answer}. \\ $$$${can}\:{we}\:{prove}\:{the}\:{first}\:{statement}? \\ $$
Commented by MrW3 last updated on 05/Jul/18

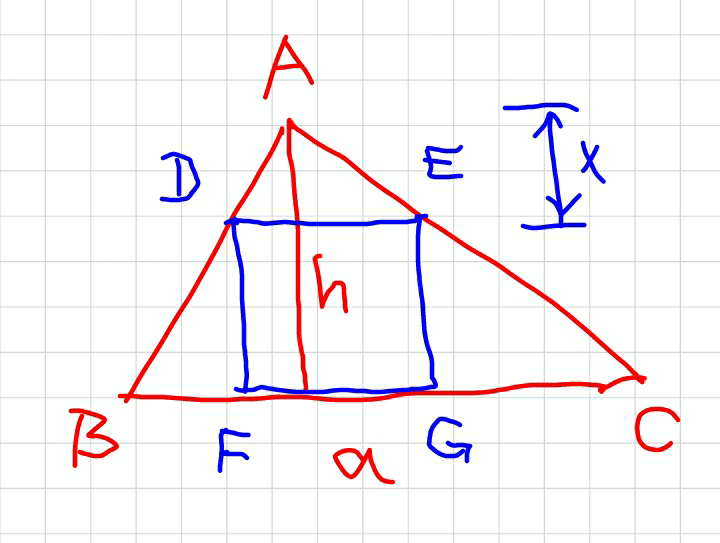
$${Statement}: \\ $$$${In}\:{every}\:{triangle}\:{it}\:{is}\:{possible}\:{to} \\ $$$${find}\:{an}\:{inscribing}\:{square}.\: \\ $$$${Proof}: \\ $$$${In}\:{every}\:{triangle}\:{there}\:{are}\:{at}\:{least} \\ $$$${two}\:{acute}\:{angles}.\:{Assume}\:{the}\:{side} \\ $$$${length}\:{between}\:{these}\:{two}\:{acute}\:{angles} \\ $$$${is}\:{a},\:{and}\:{corresponding}\:{altitude}\:{is}\:{h}. \\ $$$${DE}=\frac{{x}}{{h}}×{a} \\ $$$${DF}={h}−{x} \\ $$$${DE}={DF} \\ $$$$\frac{{x}}{{h}}{a}={h}−{x} \\ $$$$\Rightarrow{x}=\frac{{h}}{{h}+{a}}×{h} \\ $$$$\mathrm{0}<{x}<{h} \\ $$$${i}.{e}.\:{there}\:{is}\:{a}\:{x}=\frac{{h}}{{h}+{a}}×{a}\:{with}\:{which} \\ $$$${DEGF}\:{is}\:{a}\:{square}. \\ $$$${Since}\:\angle{B}\:{and}\:\angle{C}\:{are}\:{acute}, \\ $$$$\Rightarrow{F}\:{and}\:{G}\:{lie}\:{on}\:{the}\:{side}\:{BC}, \\ $$$${i}.{e}.\:{DEGF}\:{is}\:{an}\:{inscribing}\:{square}. \\ $$
Commented by MrW3 last updated on 05/Jul/18
Commented by behi83417@gmail.com last updated on 05/Jul/18
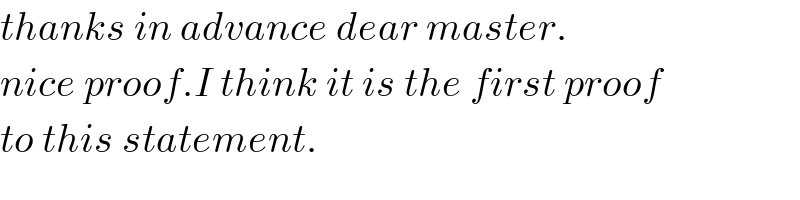
$${thanks}\:{in}\:{advance}\:{dear}\:{master}. \\ $$$${nice}\:{proof}.{I}\:{think}\:{it}\:{is}\:{the}\:{first}\:{proof} \\ $$$${to}\:{this}\:{statement}. \\ $$
