Question Number 39382 by rahul 19 last updated on 05/Jul/18

Answered by ajfour last updated on 06/Jul/18
![∫_α ^( β) f(x)g′(x)dx =[f(x)g(x)]_α ^β −∫_α ^( β) f ′(x)g(x)dx and since f(α)=f(β); g(α)=g(β) So ∫_α ^( β) f(x)g′(x)dx = −∫_α ^( β) f ′(x)g(x)dx option (b) and if A=−B A=(1/2)(A−B) Hence option (c) even. (b) and (c).](https://www.tinkutara.com/question/Q39487.png)
$$\int_{\alpha} ^{\:\:\beta} {f}\left({x}\right){g}'\left({x}\right){dx}\:=\left[{f}\left({x}\right){g}\left({x}\right)\right]_{\alpha} ^{\beta} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−\int_{\alpha} ^{\:\:\beta} {f}\:'\left({x}\right){g}\left({x}\right){dx} \\ $$$${and}\:{since}\:{f}\left(\alpha\right)={f}\left(\beta\right);\:{g}\left(\alpha\right)={g}\left(\beta\right) \\ $$$${So} \\ $$$$\int_{\alpha} ^{\:\:\beta} {f}\left({x}\right){g}'\left({x}\right){dx}\:=\:−\int_{\alpha} ^{\:\:\beta} {f}\:'\left({x}\right){g}\left({x}\right){dx} \\ $$$$\:\:{option}\:\left({b}\right) \\ $$$${and}\:\:\:{if}\:\:{A}=−{B} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{A}=\frac{\mathrm{1}}{\mathrm{2}}\left({A}−{B}\right) \\ $$$$\:\:\:{Hence}\:\:{option}\:\left({c}\right)\:{even}. \\ $$$$\left({b}\right)\:{and}\:\left({c}\right). \\ $$
Commented by rahul 19 last updated on 06/Jul/18
Why f(alpha)= f(beta)? ��
Commented by ajfour last updated on 06/Jul/18
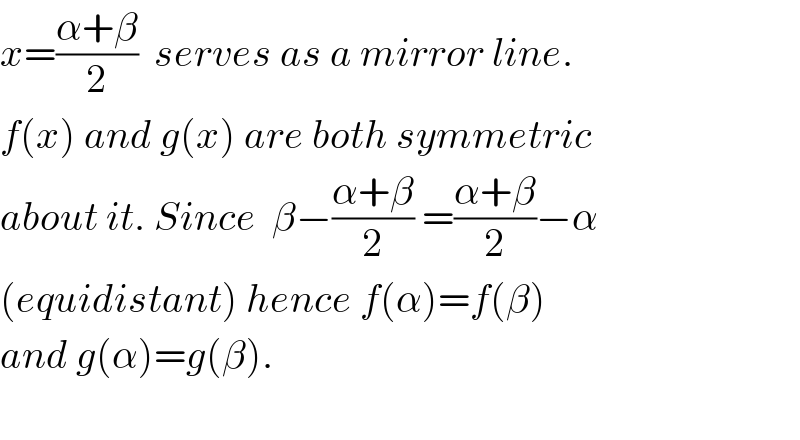
$${x}=\frac{\alpha+\beta}{\mathrm{2}}\:\:{serves}\:{as}\:{a}\:{mirror}\:{line}. \\ $$$${f}\left({x}\right)\:{and}\:{g}\left({x}\right)\:{are}\:{both}\:{symmetric} \\ $$$${about}\:{it}.\:{Since}\:\:\beta−\frac{\alpha+\beta}{\mathrm{2}}\:=\frac{\alpha+\beta}{\mathrm{2}}−\alpha \\ $$$$\left({equidistant}\right)\:{hence}\:{f}\left(\alpha\right)={f}\left(\beta\right) \\ $$$${and}\:{g}\left(\alpha\right)={g}\left(\beta\right). \\ $$$$ \\ $$
