Question Number 40375 by behi83417@gmail.com last updated on 21/Jul/18

Answered by MJS last updated on 21/Jul/18
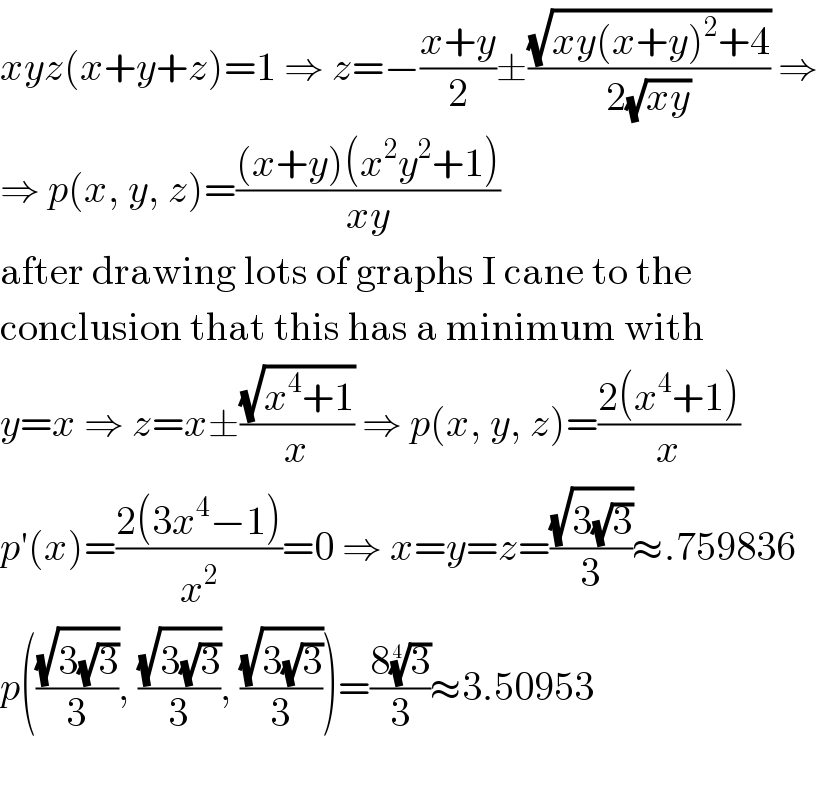
$${xyz}\left({x}+{y}+{z}\right)=\mathrm{1}\:\Rightarrow\:{z}=−\frac{{x}+{y}}{\mathrm{2}}\pm\frac{\sqrt{{xy}\left({x}+{y}\right)^{\mathrm{2}} +\mathrm{4}}}{\mathrm{2}\sqrt{{xy}}}\:\Rightarrow \\ $$$$\Rightarrow\:{p}\left({x},\:{y},\:{z}\right)=\frac{\left({x}+{y}\right)\left({x}^{\mathrm{2}} {y}^{\mathrm{2}} +\mathrm{1}\right)}{{xy}} \\ $$$$\mathrm{after}\:\mathrm{drawing}\:\mathrm{lots}\:\mathrm{of}\:\mathrm{graphs}\:\mathrm{I}\:\mathrm{cane}\:\mathrm{to}\:\mathrm{the} \\ $$$$\mathrm{conclusion}\:\mathrm{that}\:\mathrm{this}\:\mathrm{has}\:\mathrm{a}\:\mathrm{minimum}\:\mathrm{with} \\ $$$${y}={x}\:\Rightarrow\:{z}={x}\pm\frac{\sqrt{{x}^{\mathrm{4}} +\mathrm{1}}}{{x}}\:\Rightarrow\:{p}\left({x},\:{y},\:{z}\right)=\frac{\mathrm{2}\left({x}^{\mathrm{4}} +\mathrm{1}\right)}{{x}} \\ $$$${p}'\left({x}\right)=\frac{\mathrm{2}\left(\mathrm{3}{x}^{\mathrm{4}} −\mathrm{1}\right)}{{x}^{\mathrm{2}} }=\mathrm{0}\:\Rightarrow\:{x}={y}={z}=\frac{\sqrt{\mathrm{3}\sqrt{\mathrm{3}}}}{\mathrm{3}}\approx.\mathrm{759836} \\ $$$${p}\left(\frac{\sqrt{\mathrm{3}\sqrt{\mathrm{3}}}}{\mathrm{3}},\:\frac{\sqrt{\mathrm{3}\sqrt{\mathrm{3}}}}{\mathrm{3}},\:\frac{\sqrt{\mathrm{3}\sqrt{\mathrm{3}}}}{\mathrm{3}}\right)=\frac{\mathrm{8}\sqrt[{\mathrm{4}}]{\mathrm{3}}}{\mathrm{3}}\approx\mathrm{3}.\mathrm{50953} \\ $$$$ \\ $$
Commented by behi83417@gmail.com last updated on 21/Jul/18

$${thank}\:{you}\:{so}\:{much}\:{dear}. \\ $$
