Question Number 41530 by physics last updated on 09/Aug/18

Answered by tanmay.chaudhury50@gmail.com last updated on 09/Aug/18
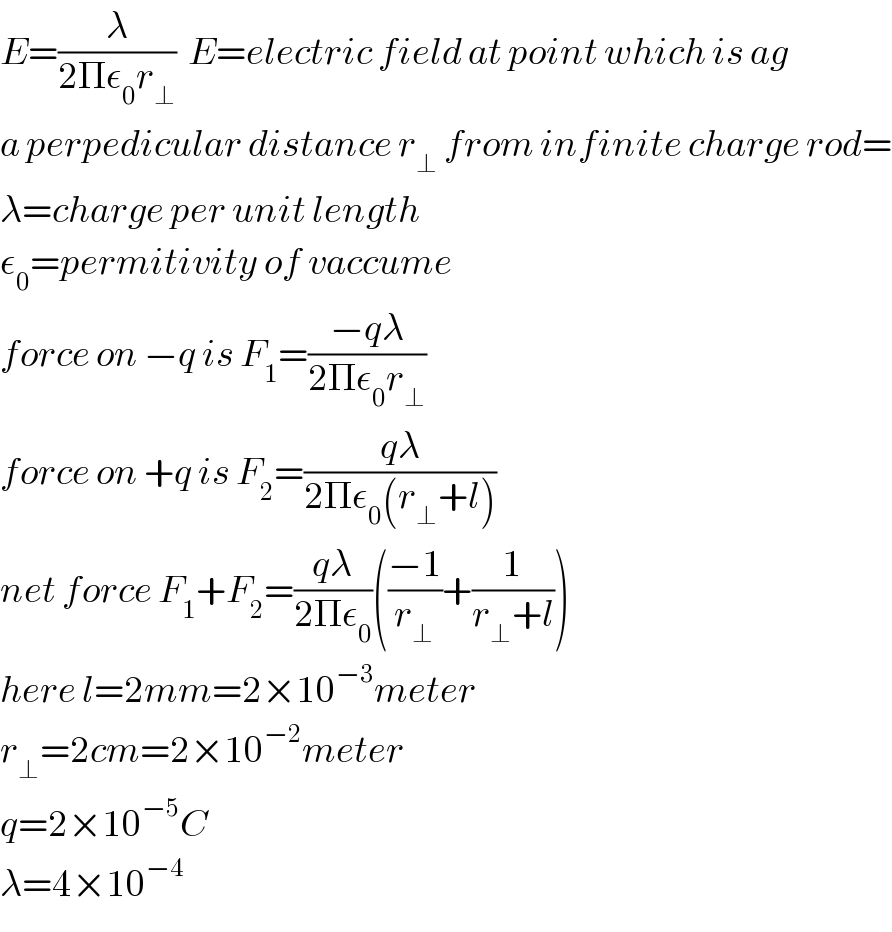
$${E}=\frac{\lambda}{\mathrm{2}\Pi\epsilon_{\mathrm{0}} {r}_{\bot} }\:\:{E}={electric}\:{field}\:{at}\:{point}\:{which}\:{is}\:{ag} \\ $$$${a}\:{perpedicular}\:{distance}\:{r}_{\bot} \:{from}\:{infinite}\:{charge}\:{rod}= \\ $$$$\lambda={charge}\:{per}\:{unit}\:{length} \\ $$$$\epsilon_{\mathrm{0}} ={permitivity}\:{of}\:{vaccume} \\ $$$${force}\:{on}\:−{q}\:{is}\:{F}_{\mathrm{1}} =\frac{−{q}\lambda}{\mathrm{2}\Pi\epsilon_{\mathrm{0}} {r}_{\bot} } \\ $$$${force}\:{on}\:+{q}\:{is}\:{F}_{\mathrm{2}} =\frac{{q}\lambda}{\mathrm{2}\Pi\epsilon_{\mathrm{0}} \left({r}_{\bot} +{l}\right)} \\ $$$${net}\:{force}\:{F}_{\mathrm{1}} +{F}_{\mathrm{2}} =\frac{{q}\lambda}{\mathrm{2}\Pi\epsilon_{\mathrm{0}} }\left(\frac{−\mathrm{1}}{{r}_{\bot} }+\frac{\mathrm{1}}{{r}_{\bot} +{l}}\right) \\ $$$${here}\:{l}=\mathrm{2}{mm}=\mathrm{2}×\mathrm{10}^{−\mathrm{3}} {meter} \\ $$$${r}_{\bot} =\mathrm{2}{cm}=\mathrm{2}×\mathrm{10}^{−\mathrm{2}} {meter} \\ $$$${q}=\mathrm{2}×\mathrm{10}^{−\mathrm{5}} {C} \\ $$$$\lambda=\mathrm{4}×\mathrm{10}^{−\mathrm{4}} \\ $$
