Menu Close

Question-43263

Tinku Tara 0 Comments
Pin




Question Number 43263 by MrW3 last updated on 09/Sep/18
Answered by MJS last updated on 09/Sep/18
AB is a straight line on the mantle in the plain C= ((0),(0) ) angle ACB =(r/s)360°=((20)/(60))360°=120° circle A x^2 +y^2 =60^2 circle B x^2 +y^2 =50^2 A= (((−60)),(0) ) B= (((25)),((25(√3))) ) AB: y=((5(√3))/(17))x+((300(√3))/(17)) the turning point is T= ((t),((((5(√3))/(17))t+((300(√3))/(17)))) ) with mimimal ∣CT∣ ((364t^2 +9000t+270000)/(189)) ⇒ min (d/dt)=((728)/(189))t+((9000)/(189))=0 ⇒ t=−((1125)/(91)) T= (((−((1125)/(91)))),(((1275(√3))/(91))) ) ∣AB∣=10(√(91))≈95.39 ∣AT∣=((510(√(91)))/(91))≈53.46 ∣TB∣=((400(√(91)))/(91))≈41.93
$${AB}\:\mathrm{is}\:\mathrm{a}\:\mathrm{straight}\:\mathrm{line}\:\mathrm{on}\:\mathrm{the}\:\mathrm{mantle} \\ $$$$\mathrm{in}\:\mathrm{the}\:\mathrm{plain} \\ $$$${C}=\begin{pmatrix}{\mathrm{0}}\\{\mathrm{0}}\end{pmatrix} \\ $$$$\mathrm{angle}\:{ACB}\:=\frac{{r}}{{s}}\mathrm{360}°=\frac{\mathrm{20}}{\mathrm{60}}\mathrm{360}°=\mathrm{120}° \\ $$$$\mathrm{circle}\:\mathrm{A} \\ $$$${x}^{\mathrm{2}} +{y}^{\mathrm{2}} =\mathrm{60}^{\mathrm{2}} \\ $$$$\mathrm{circle}\:{B} \\ $$$${x}^{\mathrm{2}} +{y}^{\mathrm{2}} =\mathrm{50}^{\mathrm{2}} \\ $$$$ \\ $$$${A}=\begin{pmatrix}{−\mathrm{60}}\\{\mathrm{0}}\end{pmatrix}\:\:\:\:\:{B}=\begin{pmatrix}{\mathrm{25}}\\{\mathrm{25}\sqrt{\mathrm{3}}}\end{pmatrix} \\ $$$${AB}:\:{y}=\frac{\mathrm{5}\sqrt{\mathrm{3}}}{\mathrm{17}}{x}+\frac{\mathrm{300}\sqrt{\mathrm{3}}}{\mathrm{17}} \\ $$$$\mathrm{the}\:\mathrm{turning}\:\mathrm{point}\:\mathrm{is}\:{T}=\begin{pmatrix}{{t}}\\{\frac{\mathrm{5}\sqrt{\mathrm{3}}}{\mathrm{17}}{t}+\frac{\mathrm{300}\sqrt{\mathrm{3}}}{\mathrm{17}}}\end{pmatrix}\:\mathrm{with} \\ $$$$\mathrm{mimimal}\:\mid{CT}\mid \\ $$$$\frac{\mathrm{364}{t}^{\mathrm{2}} +\mathrm{9000}{t}+\mathrm{270000}}{\mathrm{189}}\:\Rightarrow\:\mathrm{min} \\ $$$$\frac{{d}}{{dt}}=\frac{\mathrm{728}}{\mathrm{189}}{t}+\frac{\mathrm{9000}}{\mathrm{189}}=\mathrm{0}\:\Rightarrow\:{t}=−\frac{\mathrm{1125}}{\mathrm{91}} \\ $$$${T}=\begin{pmatrix}{−\frac{\mathrm{1125}}{\mathrm{91}}}\\{\frac{\mathrm{1275}\sqrt{\mathrm{3}}}{\mathrm{91}}}\end{pmatrix} \\ $$$$\mid{AB}\mid=\mathrm{10}\sqrt{\mathrm{91}}\approx\mathrm{95}.\mathrm{39} \\ $$$$\mid{AT}\mid=\frac{\mathrm{510}\sqrt{\mathrm{91}}}{\mathrm{91}}\approx\mathrm{53}.\mathrm{46} \\ $$$$\mid{TB}\mid=\frac{\mathrm{400}\sqrt{\mathrm{91}}}{\mathrm{91}}\approx\mathrm{41}.\mathrm{93} \\ $$$$ \\ $$
Commented by MrW3 last updated on 09/Sep/18
thank you sir! answer is correct.
$${thank}\:{you}\:{sir}!\:{answer}\:{is}\:{correct}. \\ $$
Answered by MrW3 last updated on 09/Sep/18
Commented by MrW3 last updated on 09/Sep/18
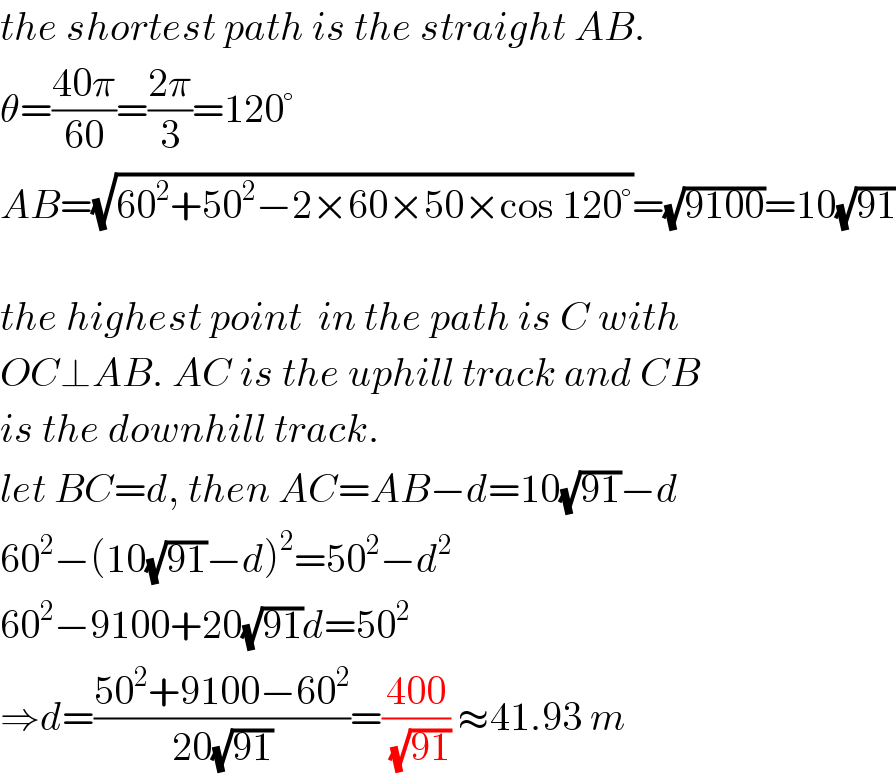
the shortest path is the straight AB. θ=((40π)/(60))=((2π)/3)=120° AB=(√(60^2 +50^2 −2×60×50×cos 120°))=(√(9100))=10(√(91)) the highest point in the path is C with OC⊥AB. AC is the uphill track and CB is the downhill track. let BC=d, then AC=AB−d=10(√(91))−d 60^2 −(10(√(91))−d)^2 =50^2 −d^2 60^2 −9100+20(√(91))d=50^2 ⇒d=((50^2 +9100−60^2 )/(20(√(91))))=((400)/( (√(91)))) ≈41.93 m
$${the}\:{shortest}\:{path}\:{is}\:{the}\:{straight}\:{AB}. \\ $$$$\theta=\frac{\mathrm{40}\pi}{\mathrm{60}}=\frac{\mathrm{2}\pi}{\mathrm{3}}=\mathrm{120}° \\ $$$${AB}=\sqrt{\mathrm{60}^{\mathrm{2}} +\mathrm{50}^{\mathrm{2}} −\mathrm{2}×\mathrm{60}×\mathrm{50}×\mathrm{cos}\:\mathrm{120}°}=\sqrt{\mathrm{9100}}=\mathrm{10}\sqrt{\mathrm{91}} \\ $$$$ \\ $$$${the}\:{highest}\:{point}\:\:{in}\:{the}\:{path}\:{is}\:{C}\:{with} \\ $$$${OC}\bot{AB}.\:{AC}\:{is}\:{the}\:{uphill}\:{track}\:{and}\:{CB} \\ $$$${is}\:{the}\:{downhill}\:{track}. \\ $$$${let}\:{BC}={d},\:{then}\:{AC}={AB}−{d}=\mathrm{10}\sqrt{\mathrm{91}}−{d} \\ $$$$\mathrm{60}^{\mathrm{2}} −\left(\mathrm{10}\sqrt{\mathrm{91}}−{d}\right)^{\mathrm{2}} =\mathrm{50}^{\mathrm{2}} −{d}^{\mathrm{2}} \\ $$$$\mathrm{60}^{\mathrm{2}} −\mathrm{9100}+\mathrm{20}\sqrt{\mathrm{91}}{d}=\mathrm{50}^{\mathrm{2}} \\ $$$$\Rightarrow{d}=\frac{\mathrm{50}^{\mathrm{2}} +\mathrm{9100}−\mathrm{60}^{\mathrm{2}} }{\mathrm{20}\sqrt{\mathrm{91}}}=\frac{\mathrm{400}}{\:\sqrt{\mathrm{91}}}\:\approx\mathrm{41}.\mathrm{93}\:{m} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *