Question Number 43384 by peter frank last updated on 10/Sep/18

Answered by tanmay.chaudhury50@gmail.com last updated on 10/Sep/18

$${cos}\mathrm{30}^{{o}} =\frac{\frac{{a}}{\mathrm{2}}}{{R}} \\ $$$$\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}=\frac{{a}}{\mathrm{2}{R}} \\ $$$${a}={R}\sqrt{\mathrm{3}}\: \\ $$$${a}=\mathrm{5}\sqrt{\mathrm{3}}\: \\ $$$${area}\:{triangle}=\frac{\sqrt{\mathrm{3}}\:}{\mathrm{4}}×{a}^{\mathrm{2}} =\frac{\sqrt{\mathrm{3}}\:}{\mathrm{4}}×\mathrm{75} \\ $$$${perimetdr}\:{triangle}=\mathrm{3}{a}=\mathrm{3}×\mathrm{5}\sqrt{\mathrm{3}}\:=\mathrm{15}\sqrt{\mathrm{3}}\: \\ $$$${area}\:{of}\:{circle}\:\Pi{R}^{\mathrm{2}} \\ $$$$=\Pi×\mathrm{5}^{\mathrm{2}} =\mathrm{25}\Pi \\ $$$${area}\:{betwedn}\:{circle}\:{and}\:{triangle} \\ $$$$=\mathrm{25}\Pi−\frac{\mathrm{75}\sqrt{\mathrm{3}}}{\mathrm{4}} \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 10/Sep/18

Commented by peter frank last updated on 10/Sep/18

$$ \\ $$$$ \\ $$$$ \\ $$$${cos}\mathrm{30}?\:{where}\:{do}\:{u}\:{get} \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 10/Sep/18
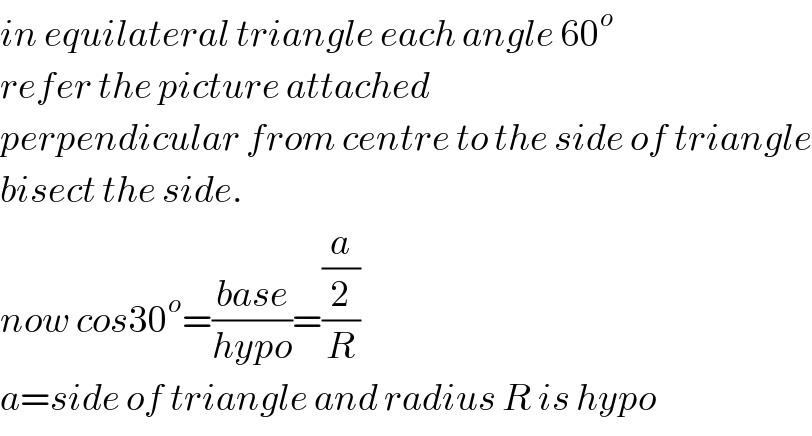
$${in}\:{equilateral}\:{triangle}\:{each}\:{angle}\:\mathrm{60}^{{o}} \\ $$$${refer}\:{the}\:{picture}\:{attached} \\ $$$${perpendicular}\:{from}\:{centre}\:{to}\:{the}\:{side}\:{of}\:{triangle} \\ $$$${bisect}\:{the}\:{side}. \\ $$$${now}\:{cos}\mathrm{30}^{{o}} =\frac{{base}}{{hypo}}=\frac{\frac{{a}}{\mathrm{2}}}{{R}} \\ $$$${a}={side}\:{of}\:{triangle}\:{and}\:{radius}\:{R}\:{is}\:{hypo} \\ $$
Commented by peter frank last updated on 10/Sep/18

$$ \\ $$$${thanks} \\ $$
