Question Number 44441 by Tawa1 last updated on 29/Sep/18

Answered by tanmay.chaudhury50@gmail.com last updated on 29/Sep/18
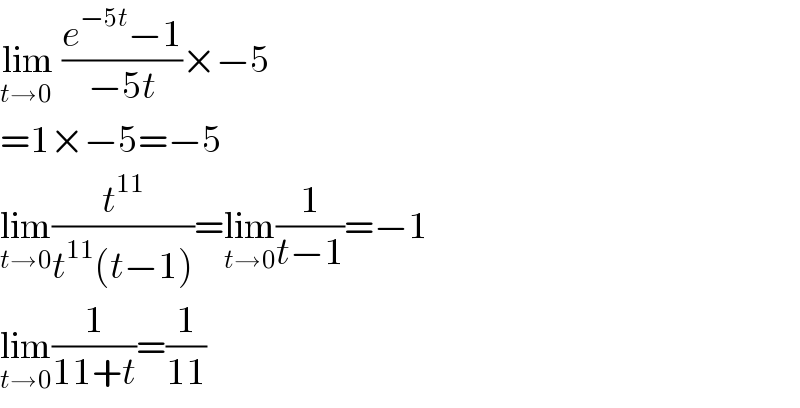
$$\underset{{t}\rightarrow\mathrm{0}\:} {\mathrm{lim}}\:\frac{{e}^{−\mathrm{5}{t}} −\mathrm{1}}{−\mathrm{5}{t}}×−\mathrm{5} \\ $$$$=\mathrm{1}×−\mathrm{5}=−\mathrm{5} \\ $$$$\underset{{t}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{t}^{\mathrm{11}} }{{t}^{\mathrm{11}} \left({t}−\mathrm{1}\right)}=\underset{{t}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}}{{t}−\mathrm{1}}=−\mathrm{1} \\ $$$$\underset{{t}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}}{\mathrm{11}+{t}}=\frac{\mathrm{1}}{\mathrm{11}} \\ $$
Commented by Tawa1 last updated on 29/Sep/18

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
