Menu Close

Question-45730

Tinku Tara 0 Comments
Pin




Question Number 45730 by ajfour last updated on 16/Oct/18
Commented by ajfour last updated on 16/Oct/18
If base BC of △ABC has slipped to the base edges of box and its sides AB and AC touch (rests against) poimts P and Q respectively of the two upper edges of the box, find A(x,y,z) in terms of sides a,b, c of △ and dimensions l, w, h of the open box.
$${If}\:{base}\:{BC}\:{of}\:\bigtriangleup{ABC}\:{has}\:{slipped}\: \\ $$$${to}\:{the}\:{base}\:{edges}\:{of}\:{box}\:{and}\:{its} \\ $$$${sides}\:{AB}\:{and}\:{AC}\:{touch}\:\left({rests}\right. \\ $$$$\left.{against}\right)\:{poimts}\:{P}\:{and}\:{Q} \\ $$$${respectively}\:{of}\:{the}\:{two}\:{upper} \\ $$$${edges}\:{of}\:{the}\:{box},\:{find}\:{A}\left({x},{y},{z}\right) \\ $$$${in}\:{terms}\:{of}\:{sides}\:\boldsymbol{{a}},\boldsymbol{{b}},\:\boldsymbol{{c}}\:\:{of}\:\bigtriangleup\:{and} \\ $$$${dimensions}\:\boldsymbol{{l}},\:\boldsymbol{{w}},\:\boldsymbol{{h}}\:{of}\:{the}\:{open}\:{box}. \\ $$
Commented by ajfour last updated on 17/Oct/18
MrW Sir please solve this.. what a Question !
$${MrW}\:{Sir}\:\:{please}\:{solve}\:{this}.. \\ $$$$\:\:{what}\:{a}\:{Question}\:! \\ $$
Commented by MrW3 last updated on 17/Oct/18
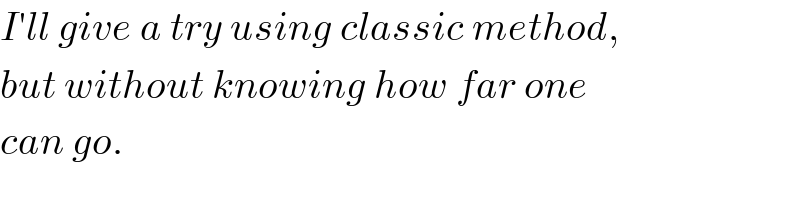
I′ll give a try using classic method, but without knowing how far one can go.
$${I}'{ll}\:{give}\:{a}\:{try}\:{using}\:{classic}\:{method}, \\ $$$${but}\:{without}\:{knowing}\:{how}\:{far}\:{one} \\ $$$${can}\:{go}. \\ $$
Answered by ajfour last updated on 17/Oct/18
let y_P = p , x_Q =q r_A ^� = xi^� +yj^� +zk^� = x_B i^� +λ[(l−x_B )i^� +p j^� +hk^� ] = y_C j^� +μ[qi^� +(w−y_C )j^� +hk^� ] ⇒ λ=μ = ε (say) q=l+(((1−ε)/ε))x_B ....(i) p=w+(((1−ε)/ε))y_C ....(ii) (l−x_B )^2 +p^2 +h^2 = c^2 /ε^2 ....(iii) q^2 +(w−y_C )^2 +h^2 = b^2 /ε^2 ...(iv) x_B ^2 +y_C ^2 =a^2 ....(v) let ((1−ε)/ε)= f ⇒ ε = (1/(1+f)) using (ii) in (iii) (l−x_B )^2 +(w+fy_C )^2 +h^2 =(1+f)^2 c^2 similarly using (i) in (iv) (l+fx_B )^2 +(w−y_C )^2 +h^2 =(1+f)^2 b^2 let l^2 +w^2 +h^2 = s^2 ⇒ −2lx_B +x_B ^2 +2fwy_C +f^2 y_C ^2 = (1+f)^2 c^2 −s^2 ...(I) −2wy_C +y_C ^2 +2flx_B +f^2 x_B ^2 = (1+f)^2 b^2 −s^2 ..(II) adding the two −2(1−f)(lx_B +wy_C )+(1+f^2 )a^2 = (1+f)^2 (b^2 +c^2 )−2s^2 let lx_B +wy_C =(a(√(l^2 +w^2 )) )sin φ sin φ = (((1+f)^2 (b^2 +c^2 )−(1+f^2 )a^2 −2s^2 )/(2(f−1)a(√(l^2 +w^2 )))) and x_B ^2 +y_C ^2 = a^2 ; ⇒ x_B =acos θ , y_C = asin θ ⇒ a(√(l^2 +w^2 )) sin(θ+tan^(−1) (l/w))= (a(√(l^2 +w^2 )) )sin φ ⇒ θ = φ−β ; β = tan^(−1) (l/w) hence x_B = acos (φ−β) y_C = asin (φ−β) since φ(f) function of f we can now use either of eqs. (I) and (II) to obtain f ; then φ, x_B , y_B , p, q, and finally r_A = xi^� +yj^� +zk^� ; A(x,y,z) .
$${let}\:{y}_{{P}} =\:{p}\:,\:\:{x}_{{Q}} ={q} \\ $$$$\bar {{r}}_{{A}} =\:{x}\hat {{i}}+{y}\hat {{j}}+{z}\hat {{k}}\: \\ $$$$\:\:\:\:\:\:=\:{x}_{{B}} \hat {{i}}+\lambda\left[\left(\boldsymbol{{l}}−{x}_{{B}} \right)\hat {{i}}+{p}\:\hat {{j}}+{h}\hat {{k}}\right] \\ $$$$\:\:\:\:\:\:=\:{y}_{{C}} \:\hat {{j}}+\mu\left[{q}\hat {{i}}+\left({w}−{y}_{{C}} \right)\hat {{j}}+{h}\hat {{k}}\right]\:\:\: \\ $$$$\Rightarrow\:\:\lambda=\mu\:=\:\epsilon\:\left({say}\right) \\ $$$$\:\:\:\:\:\:\:\:{q}={l}+\left(\frac{\mathrm{1}−\epsilon}{\epsilon}\right){x}_{{B}} \:\:\:….\left({i}\right) \\ $$$$\:\:\:\:\:\:\:\:{p}={w}+\left(\frac{\mathrm{1}−\epsilon}{\epsilon}\right){y}_{{C}} \:\:\:\:\:\:\:….\left({ii}\right) \\ $$$$\:\:\:\left({l}−{x}_{{B}} \right)^{\mathrm{2}} +{p}^{\mathrm{2}} +{h}^{\mathrm{2}} =\:{c}^{\mathrm{2}} /\epsilon^{\mathrm{2}} \:\:\:\:\:\:….\left({iii}\right) \\ $$$$\:\:\:{q}^{\mathrm{2}} +\left({w}−{y}_{{C}} \right)^{\mathrm{2}} +{h}^{\mathrm{2}} =\:{b}^{\mathrm{2}} /\epsilon^{\mathrm{2}} \:\:\:\:\:\:\:…\left({iv}\right) \\ $$$$\:\:\:\:{x}_{{B}} ^{\mathrm{2}} +{y}_{{C}} ^{\mathrm{2}} \:={a}^{\mathrm{2}} \:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:….\left({v}\right) \\ $$$${let}\:\:\frac{\mathrm{1}−\epsilon}{\epsilon}=\:{f}\:\:\:\:\:\Rightarrow\:\:\epsilon\:=\:\frac{\mathrm{1}}{\mathrm{1}+{f}} \\ $$$$\:\:{using}\:\left({ii}\right)\:{in}\:\left({iii}\right) \\ $$$$\:\left({l}−{x}_{{B}} \right)^{\mathrm{2}} +\left({w}+{fy}_{{C}} \right)^{\mathrm{2}} +{h}^{\mathrm{2}} =\left(\mathrm{1}+{f}\right)^{\mathrm{2}} {c}^{\mathrm{2}} \\ $$$$\:\:{similarly}\:{using}\:\left({i}\right)\:{in}\:\left({iv}\right) \\ $$$$\:\left({l}+{fx}_{{B}} \right)^{\mathrm{2}} +\left({w}−{y}_{{C}} \right)^{\mathrm{2}} +{h}^{\mathrm{2}} =\left(\mathrm{1}+{f}\right)^{\mathrm{2}} {b}^{\mathrm{2}} \\ $$$$\:{let}\:\:\:\:{l}^{\mathrm{2}} +{w}^{\mathrm{2}} +{h}^{\mathrm{2}} \:=\:{s}^{\mathrm{2}} \\ $$$$\:\Rightarrow\:\:−\mathrm{2}{lx}_{{B}} +{x}_{{B}} ^{\mathrm{2}} +\mathrm{2}{fwy}_{{C}} +{f}^{\mathrm{2}} {y}_{{C}} ^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\left(\mathrm{1}+{f}\right)^{\mathrm{2}} {c}^{\mathrm{2}} −{s}^{\mathrm{2}} \:\:\:…\left({I}\right) \\ $$$$\:\:\:\:\:−\mathrm{2}{wy}_{{C}} +{y}_{{C}} ^{\mathrm{2}} +\mathrm{2}{flx}_{{B}} +{f}^{\mathrm{2}} {x}_{{B}} ^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\left(\mathrm{1}+{f}\right)^{\mathrm{2}} {b}^{\mathrm{2}} −{s}^{\mathrm{2}} \:\:\:\:..\left({II}\right) \\ $$$${adding}\:{the}\:{two} \\ $$$$\:−\mathrm{2}\left(\mathrm{1}−{f}\right)\left({lx}_{{B}} +{wy}_{{C}} \right)+\left(\mathrm{1}+{f}^{\mathrm{2}} \right){a}^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\left(\mathrm{1}+{f}\right)^{\mathrm{2}} \left({b}^{\mathrm{2}} +{c}^{\mathrm{2}} \right)−\mathrm{2}{s}^{\mathrm{2}} \\ $$$$\:\:{let}\:\:\:{lx}_{{B}} +{wy}_{{C}} \:=\left({a}\sqrt{{l}^{\mathrm{2}} +{w}^{\mathrm{2}} }\:\right)\mathrm{sin}\:\phi \\ $$$$\:\mathrm{sin}\:\phi\:=\:\frac{\left(\mathrm{1}+{f}\right)^{\mathrm{2}} \left({b}^{\mathrm{2}} +{c}^{\mathrm{2}} \right)−\left(\mathrm{1}+{f}^{\mathrm{2}} \right){a}^{\mathrm{2}} −\mathrm{2}{s}^{\mathrm{2}} }{\mathrm{2}\left({f}−\mathrm{1}\right){a}\sqrt{{l}^{\mathrm{2}} +{w}^{\mathrm{2}} }} \\ $$$${and}\:\:\:\:{x}_{{B}} ^{\mathrm{2}} +{y}_{{C}} ^{\mathrm{2}} =\:{a}^{\mathrm{2}} ;\: \\ $$$$\Rightarrow\:\:\:\:\:{x}_{{B}} ={a}\mathrm{cos}\:\theta\:,\:{y}_{{C}} =\:{a}\mathrm{sin}\:\theta \\ $$$$\Rightarrow\:\:{a}\sqrt{{l}^{\mathrm{2}} +{w}^{\mathrm{2}} }\:\mathrm{sin}\left(\theta+\mathrm{tan}^{−\mathrm{1}} \frac{{l}}{{w}}\right)=\:\left({a}\sqrt{{l}^{\mathrm{2}} +{w}^{\mathrm{2}} }\:\right)\mathrm{sin}\:\phi \\ $$$$\Rightarrow\:\:\:\:\theta\:=\:\phi−\beta\:\:;\:\:\beta\:=\:\mathrm{tan}^{−\mathrm{1}} \frac{{l}}{{w}} \\ $$$${hence}\:\:\boldsymbol{{x}}_{\boldsymbol{{B}}} =\:{a}\mathrm{cos}\:\left(\phi−\beta\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{{y}}_{\boldsymbol{{C}}} \:=\:{a}\mathrm{sin}\:\left(\phi−\beta\right) \\ $$$$\:{since}\:\:\phi\left({f}\right)\:\:{function}\:{of}\:{f} \\ $$$${we}\:{can}\:{now}\:{use}\:{either}\:{of}\: \\ $$$$\:{eqs}.\:\left({I}\right)\:{and}\:\left({II}\right)\:{to}\:{obtain}\:\boldsymbol{{f}}\:; \\ $$$${then}\:\phi,\:{x}_{{B}} \:,\:{y}_{{B}} \:,\:{p},\:{q},\:{and}\:{finally} \\ $$$$\:\:{r}_{{A}} =\:{x}\hat {{i}}+{y}\hat {{j}}+{z}\hat {{k}}\:\:;\:\:{A}\left({x},{y},{z}\right)\:. \\ $$
Commented by MrW3 last updated on 17/Oct/18
very powerful sir!
$${very}\:{powerful}\:{sir}! \\ $$
Answered by MrW3 last updated on 17/Oct/18
Commented by MrW3 last updated on 17/Oct/18
Commented by MrW3 last updated on 17/Oct/18
Commented by MrW3 last updated on 17/Oct/18
the position of triangle ABC is given by parameters θ and φ. the altitude of ΔABC over BC is d: Area ΔABC=(1/2)ad=(√(s(s−a)(s−b)(s−c))) ⇒d=(2/a)(√(s(s−a)(s−b)(s−c))) with s=((a+b+c)/2) z_A =d sin φ=b sin φ_1 =c sin φ_2 ⇒sin φ_1 =(d/b) sin φ ⇒sin φ_2 =(d/c) sin φ MR=(h/(sin φ)) PQ=((MR)/d)a=((ah)/(d sin φ)) BP′=(h/(tan φ_2 ))=((h(√(1−(d^2 /c^2 )sin^2 φ)))/((d/c)sin φ))=(h/(sin φ))(√((c^2 /d^2 )−sin^2 φ)) CQ′=(h/(tan φ_1 ))=((h(√(1−(d^2 /b^2 )sin^2 φ)))/((d/b)sin φ))=(h/(sin φ))(√((b^2 /d^2 )−sin^2 φ)) BP′^2 =(l−a cos θ)^2 +(w−PQ sin θ)^2 =(h^2 /(sin^2 φ))((c^2 /d^2 )−sin^2 φ) ⇒(l−a cos θ)^2 +(w−((ah)/(d sin φ)) sin θ)^2 =h^2 ((c^2 /d^2 ) sin^2 φ−1) ⇒l^2 −2al cos θ+a^2 cos^2 θ+w^2 −((2ahw)/(d sin φ)) sin θ+((a^2 h^2 sin^2 θ)/(d^2 sin^2 φ))=((h^2 c^2 )/d^2 ) sin^2 φ−h^2 ⇒2al cos θ−a^2 cos^2 θ+((2ahw sin θ)/(d sin φ)) −((a^2 h^2 sin^2 θ)/(d^2 sin^2 φ))+((h^2 c^2 sin^2 φ)/d^2 )−(l^2 +w^2 +h^2 )=0 ⇒2ad^2 l cos θ sin^2 φ−a^2 d^2 cos^2 θ sin^2 φ+2adhw sin θ sin φ −a^2 h^2 sin^2 θ+c^2 h^2 sin^4 φ−d^2 (l^2 +w^2 +h^2 ) sin^2 φ=0 ...(i) CQ′^2 =(w−a sin θ)^2 +(l−PQ cos θ)^2 =(h^2 /(sin^2 φ))((b^2 /d^2 )−sin^2 φ) ⇒(w−a sin θ)^2 +(l−((ah)/(d sin φ)) cos θ)^2 =(h^2 /(sin^2 φ))((b^2 /d^2 )−sin^2 φ) ⇒w^2 −2aw sin θ+a^2 sin^2 θ+l^2 −((2ahl)/(d sin φ)) cos θ+((a^2 h^2 cos^2 θ)/(d^2 sin^2 φ))=((h^2 b^2 )/d^2 ) sin^2 φ−h^2 ⇒2aw sin θ−a^2 sin^2 θ+((2ahl)/(d sin φ)) cos θ−((a^2 h^2 cos^2 θ)/(d^2 sin^2 φ))+((h^2 b^2 )/d^2 ) sin^2 φ=l^2 +w^2 +h^2 ⇒2ad^2 w sin θ sin^2 φ−a^2 d^2 sin^2 θ sin^2 φ+2adhl cos θ sin φ−a^2 h^2 cos^2 θ+b^2 h^2 sin^4 φ−d^2 (l^2 +w^2 +h^2 ) sin^2 φ=0 ...(ii) .... .... two unknowns θ and φ in two eqn.
$${the}\:{position}\:{of}\:{triangle}\:{ABC}\:{is}\:{given} \\ $$$${by}\:{parameters}\:\theta\:{and}\:\phi. \\ $$$$ \\ $$$${the}\:{altitude}\:{of}\:\Delta{ABC}\:{over}\:{BC}\:{is}\:{d}: \\ $$$${Area}\:\Delta{ABC}=\frac{\mathrm{1}}{\mathrm{2}}{ad}=\sqrt{{s}\left({s}−{a}\right)\left({s}−{b}\right)\left({s}−{c}\right)} \\ $$$$\Rightarrow{d}=\frac{\mathrm{2}}{{a}}\sqrt{{s}\left({s}−{a}\right)\left({s}−{b}\right)\left({s}−{c}\right)}\:{with}\:{s}=\frac{{a}+{b}+{c}}{\mathrm{2}} \\ $$$${z}_{{A}} ={d}\:\mathrm{sin}\:\phi={b}\:\mathrm{sin}\:\phi_{\mathrm{1}} ={c}\:\mathrm{sin}\:\phi_{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{sin}\:\phi_{\mathrm{1}} =\frac{{d}}{{b}}\:\mathrm{sin}\:\phi \\ $$$$\Rightarrow\mathrm{sin}\:\phi_{\mathrm{2}} =\frac{{d}}{{c}}\:\mathrm{sin}\:\phi \\ $$$${MR}=\frac{{h}}{\mathrm{sin}\:\phi} \\ $$$${PQ}=\frac{{MR}}{{d}}{a}=\frac{{ah}}{{d}\:\mathrm{sin}\:\phi} \\ $$$${BP}'=\frac{{h}}{\mathrm{tan}\:\phi_{\mathrm{2}} }=\frac{{h}\sqrt{\mathrm{1}−\frac{{d}^{\mathrm{2}} }{{c}^{\mathrm{2}} }\mathrm{sin}^{\mathrm{2}} \:\phi}}{\frac{{d}}{{c}}\mathrm{sin}\:\phi}=\frac{{h}}{\mathrm{sin}\:\phi}\sqrt{\frac{{c}^{\mathrm{2}} }{{d}^{\mathrm{2}} }−\mathrm{sin}^{\mathrm{2}} \:\phi} \\ $$$${CQ}'=\frac{{h}}{\mathrm{tan}\:\phi_{\mathrm{1}} }=\frac{{h}\sqrt{\mathrm{1}−\frac{{d}^{\mathrm{2}} }{{b}^{\mathrm{2}} }\mathrm{sin}^{\mathrm{2}} \:\phi}}{\frac{{d}}{{b}}\mathrm{sin}\:\phi}=\frac{{h}}{\mathrm{sin}\:\phi}\sqrt{\frac{{b}^{\mathrm{2}} }{{d}^{\mathrm{2}} }−\mathrm{sin}^{\mathrm{2}} \:\phi} \\ $$$${BP}'^{\mathrm{2}} =\left({l}−{a}\:\mathrm{cos}\:\theta\right)^{\mathrm{2}} +\left({w}−{PQ}\:\mathrm{sin}\:\theta\right)^{\mathrm{2}} =\frac{{h}^{\mathrm{2}} }{\mathrm{sin}^{\mathrm{2}} \:\phi}\left(\frac{{c}^{\mathrm{2}} }{{d}^{\mathrm{2}} }−\mathrm{sin}^{\mathrm{2}} \:\phi\right) \\ $$$$\Rightarrow\left({l}−{a}\:\mathrm{cos}\:\theta\right)^{\mathrm{2}} +\left({w}−\frac{{ah}}{{d}\:\mathrm{sin}\:\phi}\:\mathrm{sin}\:\theta\right)^{\mathrm{2}} ={h}^{\mathrm{2}} \left(\frac{{c}^{\mathrm{2}} }{{d}^{\mathrm{2}} }\:\mathrm{sin}^{\mathrm{2}} \:\phi−\mathrm{1}\right) \\ $$$$\Rightarrow{l}^{\mathrm{2}} −\mathrm{2}{al}\:\mathrm{cos}\:\theta+{a}^{\mathrm{2}} \:\mathrm{cos}^{\mathrm{2}} \:\theta+{w}^{\mathrm{2}} −\frac{\mathrm{2}{ahw}}{{d}\:\mathrm{sin}\:\phi}\:\mathrm{sin}\:\theta+\frac{{a}^{\mathrm{2}} {h}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\theta}{{d}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\phi}=\frac{{h}^{\mathrm{2}} {c}^{\mathrm{2}} }{{d}^{\mathrm{2}} }\:\mathrm{sin}^{\mathrm{2}} \:\phi−{h}^{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{2}{al}\:\mathrm{cos}\:\theta−{a}^{\mathrm{2}} \:\mathrm{cos}^{\mathrm{2}} \:\theta+\frac{\mathrm{2}{ahw}\:\mathrm{sin}\:\theta}{{d}\:\mathrm{sin}\:\phi}\:−\frac{{a}^{\mathrm{2}} {h}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\theta}{{d}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\phi}+\frac{{h}^{\mathrm{2}} {c}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\phi}{{d}^{\mathrm{2}} }−\left({l}^{\mathrm{2}} +{w}^{\mathrm{2}} +{h}^{\mathrm{2}} \right)=\mathrm{0} \\ $$$$\Rightarrow\mathrm{2}{ad}^{\mathrm{2}} {l}\:\mathrm{cos}\:\theta\:\mathrm{sin}^{\mathrm{2}} \:\phi−{a}^{\mathrm{2}} {d}^{\mathrm{2}} \:\mathrm{cos}^{\mathrm{2}} \:\theta\:\mathrm{sin}^{\mathrm{2}} \:\phi+\mathrm{2}{adhw}\:\mathrm{sin}\:\theta\:\mathrm{sin}\:\phi\:−{a}^{\mathrm{2}} {h}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\theta+{c}^{\mathrm{2}} {h}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{4}} \:\phi−{d}^{\mathrm{2}} \left({l}^{\mathrm{2}} +{w}^{\mathrm{2}} +{h}^{\mathrm{2}} \right)\:\mathrm{sin}^{\mathrm{2}} \:\phi=\mathrm{0}\:\:\:\:…\left({i}\right) \\ $$$$ \\ $$$${CQ}'^{\mathrm{2}} =\left({w}−{a}\:\mathrm{sin}\:\theta\right)^{\mathrm{2}} +\left({l}−{PQ}\:\mathrm{cos}\:\theta\right)^{\mathrm{2}} =\frac{{h}^{\mathrm{2}} }{\mathrm{sin}^{\mathrm{2}} \:\phi}\left(\frac{{b}^{\mathrm{2}} }{{d}^{\mathrm{2}} }−\mathrm{sin}^{\mathrm{2}} \:\phi\right) \\ $$$$\Rightarrow\left({w}−{a}\:\mathrm{sin}\:\theta\right)^{\mathrm{2}} +\left({l}−\frac{{ah}}{{d}\:\mathrm{sin}\:\phi}\:\mathrm{cos}\:\theta\right)^{\mathrm{2}} =\frac{{h}^{\mathrm{2}} }{\mathrm{sin}^{\mathrm{2}} \:\phi}\left(\frac{{b}^{\mathrm{2}} }{{d}^{\mathrm{2}} }−\mathrm{sin}^{\mathrm{2}} \:\phi\right) \\ $$$$\Rightarrow{w}^{\mathrm{2}} −\mathrm{2}{aw}\:\mathrm{sin}\:\theta+{a}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\theta+{l}^{\mathrm{2}} −\frac{\mathrm{2}{ahl}}{{d}\:\mathrm{sin}\:\phi}\:\mathrm{cos}\:\theta+\frac{{a}^{\mathrm{2}} {h}^{\mathrm{2}} \:\mathrm{cos}^{\mathrm{2}} \:\theta}{{d}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\phi}=\frac{{h}^{\mathrm{2}} {b}^{\mathrm{2}} }{{d}^{\mathrm{2}} }\:\mathrm{sin}^{\mathrm{2}} \:\phi−{h}^{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{2}{aw}\:\mathrm{sin}\:\theta−{a}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\theta+\frac{\mathrm{2}{ahl}}{{d}\:\mathrm{sin}\:\phi}\:\mathrm{cos}\:\theta−\frac{{a}^{\mathrm{2}} {h}^{\mathrm{2}} \:\mathrm{cos}^{\mathrm{2}} \:\theta}{{d}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\phi}+\frac{{h}^{\mathrm{2}} {b}^{\mathrm{2}} }{{d}^{\mathrm{2}} }\:\mathrm{sin}^{\mathrm{2}} \:\phi={l}^{\mathrm{2}} +{w}^{\mathrm{2}} +{h}^{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{2}{ad}^{\mathrm{2}} {w}\:\mathrm{sin}\:\theta\:\mathrm{sin}^{\mathrm{2}} \:\phi−{a}^{\mathrm{2}} {d}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\theta\:\mathrm{sin}^{\mathrm{2}} \:\phi+\mathrm{2}{adhl}\:\mathrm{cos}\:\theta\:\mathrm{sin}\:\phi−{a}^{\mathrm{2}} {h}^{\mathrm{2}} \:\mathrm{cos}^{\mathrm{2}} \:\theta+{b}^{\mathrm{2}} {h}^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{4}} \:\phi−{d}^{\mathrm{2}} \left({l}^{\mathrm{2}} +{w}^{\mathrm{2}} +{h}^{\mathrm{2}} \right)\:\mathrm{sin}^{\mathrm{2}} \:\phi=\mathrm{0}\:\:\:\:\:…\left({ii}\right) \\ $$$$…. \\ $$$$….\:{two}\:{unknowns}\:\theta\:{and}\:\phi\:{in}\:{two}\:{eqn}. \\ $$
Commented by MrW3 last updated on 17/Oct/18
Commented by ajfour last updated on 17/Oct/18
i have corrected my solution only now.. thank you Sir, for yours.. Excellent diagrams, let me have some time comprehending your solution.
$${i}\:{have}\:{corrected}\:{my}\:{solution}\:{only}\:{now}.. \\ $$$${thank}\:{you}\:{Sir},\:{for}\:{yours}.. \\ $$$${Excellent}\:{diagrams},\:{let}\:{me}\:{have} \\ $$$${some}\:{time}\:{comprehending}\:{your} \\ $$$${solution}. \\ $$
Commented by MrW3 last updated on 17/Oct/18
let p=tan (θ/2), q=sin φ ⇒2ad^2 l (((1−p^2 )/(1+p^2 )))q^2 −a^2 d^2 (((1−p^2 )/(1+p^2 )))^2 q^2 +2adhw(((2p)/(1+p^2 )))q−a^2 h^2 (((2p)/(1+p^2 )))^2 +c^2 h^2 q^4 −d^2 (l^2 +w^2 +h^2 )q^2 =0 ⇒(1/((l^2 +w^2 +h^2 )))[2l−a+(2l+a)p^2 ](1−p^2 )q^2 +((4ahw)/(d(l^2 +w^2 +h^2 )))p(1+p^2 )q−((4a^2 h^2 )/(d^2 (l^2 +w^2 +h^2 )))p^2 +[((c^2 h^2 )/(d^2 (l^2 +w^2 +h^2 )))q^2 −1](1+p^2 )^2 q^2 =0 ...(i) ⇒4ad^2 wp(1+p^2 )^2 q^2 −4a^2 d^2 p^2 q^2 +2adhl(1−p^4 )q−a^2 h^2 (1−p^2 )^2 +b^2 h^2 (1+p^2 )^2 q^4 −d^2 (l^2 +w^2 +h^2 )(1+p^2 )q^2 =0 ⇒((4a)/((l^2 +w^2 +h^2 )))[w(1+p^2 )^2 −ap]pq^2 +((ah)/(d^2 (l^2 +w^2 +h^2 )))[2dl(1+p^2 )q−ah(1−p^2 )](1−p^2 )+[((b^2 h^2 )/(d^2 (l^2 +w^2 +h^2 )))q^2 −1](1+p^2 )^2 q^2 =0 ....(ii)
$${let}\:{p}=\mathrm{tan}\:\frac{\theta}{\mathrm{2}},\:{q}=\mathrm{sin}\:\phi \\ $$$$\Rightarrow\mathrm{2}{ad}^{\mathrm{2}} {l}\:\left(\frac{\mathrm{1}−{p}^{\mathrm{2}} }{\mathrm{1}+{p}^{\mathrm{2}} }\right){q}^{\mathrm{2}} −{a}^{\mathrm{2}} {d}^{\mathrm{2}} \left(\frac{\mathrm{1}−{p}^{\mathrm{2}} }{\mathrm{1}+{p}^{\mathrm{2}} }\right)^{\mathrm{2}} {q}^{\mathrm{2}} +\mathrm{2}{adhw}\left(\frac{\mathrm{2}{p}}{\mathrm{1}+{p}^{\mathrm{2}} }\right){q}−{a}^{\mathrm{2}} {h}^{\mathrm{2}} \left(\frac{\mathrm{2}{p}}{\mathrm{1}+{p}^{\mathrm{2}} }\right)^{\mathrm{2}} +{c}^{\mathrm{2}} {h}^{\mathrm{2}} {q}^{\mathrm{4}} −{d}^{\mathrm{2}} \left({l}^{\mathrm{2}} +{w}^{\mathrm{2}} +{h}^{\mathrm{2}} \right){q}^{\mathrm{2}} =\mathrm{0}\: \\ $$$$\Rightarrow\frac{\mathrm{1}}{\left({l}^{\mathrm{2}} +{w}^{\mathrm{2}} +{h}^{\mathrm{2}} \right)}\left[\mathrm{2}{l}−{a}+\left(\mathrm{2}{l}+{a}\right){p}^{\mathrm{2}} \right]\left(\mathrm{1}−{p}^{\mathrm{2}} \right){q}^{\mathrm{2}} +\frac{\mathrm{4}{ahw}}{{d}\left({l}^{\mathrm{2}} +{w}^{\mathrm{2}} +{h}^{\mathrm{2}} \right)}{p}\left(\mathrm{1}+{p}^{\mathrm{2}} \right){q}−\frac{\mathrm{4}{a}^{\mathrm{2}} {h}^{\mathrm{2}} }{{d}^{\mathrm{2}} \left({l}^{\mathrm{2}} +{w}^{\mathrm{2}} +{h}^{\mathrm{2}} \right)}{p}^{\mathrm{2}} +\left[\frac{{c}^{\mathrm{2}} {h}^{\mathrm{2}} }{{d}^{\mathrm{2}} \left({l}^{\mathrm{2}} +{w}^{\mathrm{2}} +{h}^{\mathrm{2}} \right)}{q}^{\mathrm{2}} −\mathrm{1}\right]\left(\mathrm{1}+{p}^{\mathrm{2}} \right)^{\mathrm{2}} {q}^{\mathrm{2}} =\mathrm{0}\:\:\:\:…\left({i}\right) \\ $$$$ \\ $$$$\Rightarrow\mathrm{4}{ad}^{\mathrm{2}} {wp}\left(\mathrm{1}+{p}^{\mathrm{2}} \right)^{\mathrm{2}} {q}^{\mathrm{2}} −\mathrm{4}{a}^{\mathrm{2}} {d}^{\mathrm{2}} {p}^{\mathrm{2}} {q}^{\mathrm{2}} +\mathrm{2}{adhl}\left(\mathrm{1}−{p}^{\mathrm{4}} \right){q}−{a}^{\mathrm{2}} {h}^{\mathrm{2}} \left(\mathrm{1}−{p}^{\mathrm{2}} \right)^{\mathrm{2}} +{b}^{\mathrm{2}} {h}^{\mathrm{2}} \left(\mathrm{1}+{p}^{\mathrm{2}} \right)^{\mathrm{2}} {q}^{\mathrm{4}} −{d}^{\mathrm{2}} \left({l}^{\mathrm{2}} +{w}^{\mathrm{2}} +{h}^{\mathrm{2}} \right)\left(\mathrm{1}+{p}^{\mathrm{2}} \right){q}^{\mathrm{2}} =\mathrm{0} \\ $$$$\Rightarrow\frac{\mathrm{4}{a}}{\left({l}^{\mathrm{2}} +{w}^{\mathrm{2}} +{h}^{\mathrm{2}} \right)}\left[{w}\left(\mathrm{1}+{p}^{\mathrm{2}} \right)^{\mathrm{2}} −{ap}\right]{pq}^{\mathrm{2}} +\frac{{ah}}{{d}^{\mathrm{2}} \left({l}^{\mathrm{2}} +{w}^{\mathrm{2}} +{h}^{\mathrm{2}} \right)}\left[\mathrm{2}{dl}\left(\mathrm{1}+{p}^{\mathrm{2}} \right){q}−{ah}\left(\mathrm{1}−{p}^{\mathrm{2}} \right)\right]\left(\mathrm{1}−{p}^{\mathrm{2}} \right)+\left[\frac{{b}^{\mathrm{2}} {h}^{\mathrm{2}} }{{d}^{\mathrm{2}} \left({l}^{\mathrm{2}} +{w}^{\mathrm{2}} +{h}^{\mathrm{2}} \right)}{q}^{\mathrm{2}} −\mathrm{1}\right]\left(\mathrm{1}+{p}^{\mathrm{2}} \right)^{\mathrm{2}} {q}^{\mathrm{2}} =\mathrm{0}\:\:\:\:\:….\left({ii}\right) \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *