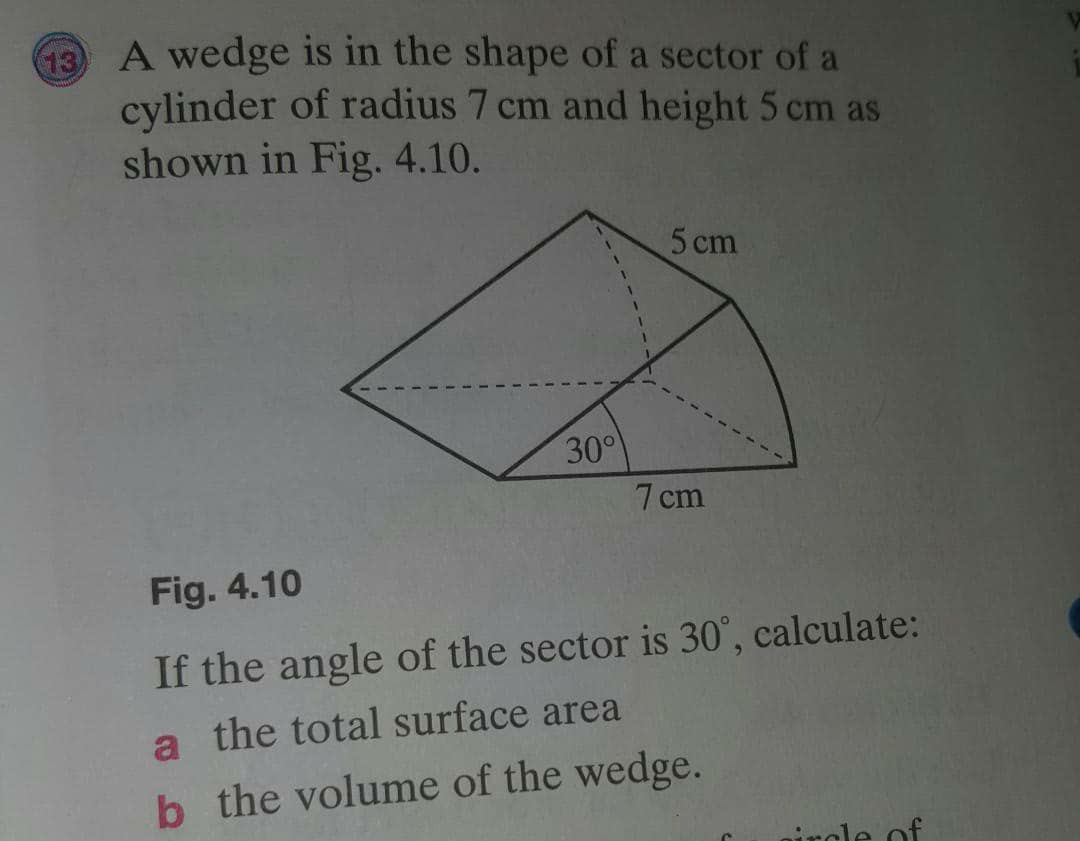
Question Number 45753 by Tawa1 last updated on 16/Oct/18
Answered by tanmay.chaudhury50@gmail.com last updated on 16/Oct/18
![for cylnder volume =πR^2 H when base is circle of area πR^2 but here volume is sector aresfor 30^o ×height requiref volume is sector area=((πR^2 )/(2π))×(π/6) [(π/6)=30^(o]) volume =((πR^2 )/(2π))×(π/6)×H=((πR^2 H)/(12))=((3.14×7^2 ×5)/(12)) totsl surface area=((πR^2 )/(12))+((πR^2 )/(12))+2×{5×7}+ ((2πRH)/(2π))×(π/6) note...total surcace area= two sectlor area of30^o +two rectangle area+ one curve area =(((πR^2 )/(12))×2)+(5×7×2)+((2πRH)/(2π))×(π/6)](https://www.tinkutara.com/question/Q45754.png)
$${for}\:{cylnder}\:{volume}\:=\pi{R}^{\mathrm{2}} {H}\:\:{when}\:{base}\:{is}\:{circle} \\ $$$${of}\:{area}\:\pi{R}^{\mathrm{2}} \\ $$$${but}\:{here}\:{volume}\:{is}\:{sector}\:{aresfor}\:\mathrm{30}^{{o}} ×{height} \\ $$$${requiref}\:{volume}\:{is} \\ $$$${sector}\:{area}=\frac{\pi{R}^{\mathrm{2}} }{\mathrm{2}\pi}×\frac{\pi}{\mathrm{6}}\:\:\:\left[\frac{\pi}{\mathrm{6}}=\mathrm{30}^{\left.{o}\right]} \right. \\ $$$${volume}\:=\frac{\pi{R}^{\mathrm{2}} }{\mathrm{2}\pi}×\frac{\pi}{\mathrm{6}}×{H}=\frac{\pi{R}^{\mathrm{2}} {H}}{\mathrm{12}}=\frac{\mathrm{3}.\mathrm{14}×\mathrm{7}^{\mathrm{2}} ×\mathrm{5}}{\mathrm{12}} \\ $$$${totsl}\:{surface}\:{area}=\frac{\pi{R}^{\mathrm{2}} }{\mathrm{12}}+\frac{\pi{R}^{\mathrm{2}} }{\mathrm{12}}+\mathrm{2}×\left\{\mathrm{5}×\mathrm{7}\right\}+ \\ $$$$ \\ $$$$\:\:\frac{\mathrm{2}\pi{RH}}{\mathrm{2}\pi}×\frac{\pi}{\mathrm{6}} \\ $$$${note}…{total}\:{surcace}\:\:{area}= \\ $$$${two}\:{sectlor}\:{area}\:{of}\mathrm{30}^{{o}} +{two}\:{rectangle}\:{area}+ \\ $$$${one}\:{curve}\:{area} \\ $$$$=\left(\frac{\pi{R}^{\mathrm{2}} }{\mathrm{12}}×\mathrm{2}\right)+\left(\mathrm{5}×\mathrm{7}×\mathrm{2}\right)+\frac{\mathrm{2}\pi{RH}}{\mathrm{2}\pi}×\frac{\pi}{\mathrm{6}} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by Tawa1 last updated on 16/Oct/18
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
