Question-46453

Question Number 46453 by MrW3 last updated on 26/Oct/18

Commented by MrW3 last updated on 27/Oct/18

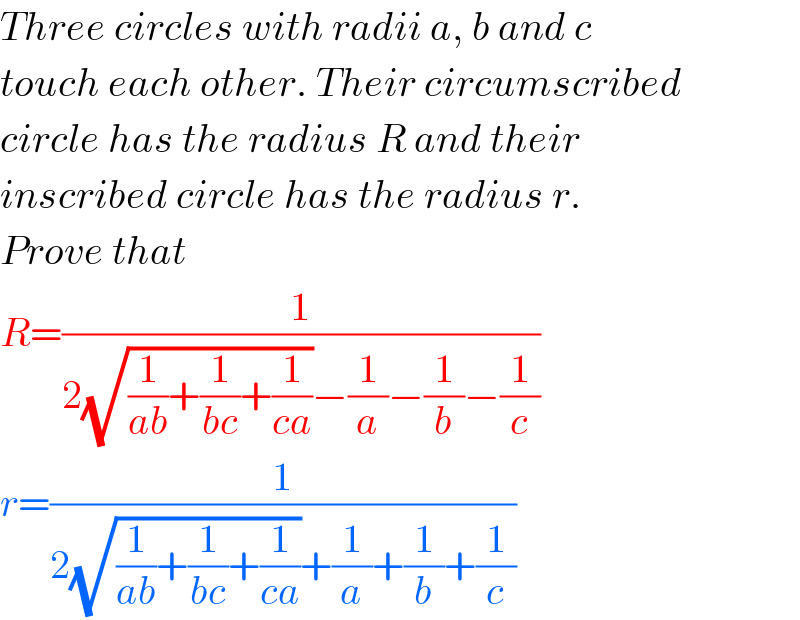
$${Three}\:{circles}\:{with}\:{radii}\:{a},\:{b}\:{and}\:{c} \\ $$$${touch}\:{each}\:{other}.\:{Their}\:{circumscribed} \\ $$$${circle}\:{has}\:{the}\:{radius}\:{R}\:{and}\:{their} \\ $$$${inscribed}\:{circle}\:{has}\:{the}\:{radius}\:{r}. \\ $$$${Prove}\:{that} \\ $$$${R}=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\frac{\mathrm{1}}{{ab}}+\frac{\mathrm{1}}{{bc}}+\frac{\mathrm{1}}{{ca}}}−\frac{\mathrm{1}}{{a}}−\frac{\mathrm{1}}{{b}}−\frac{\mathrm{1}}{{c}}} \\ $$$${r}=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\frac{\mathrm{1}}{{ab}}+\frac{\mathrm{1}}{{bc}}+\frac{\mathrm{1}}{{ca}}}+\frac{\mathrm{1}}{{a}}+\frac{\mathrm{1}}{{b}}+\frac{\mathrm{1}}{{c}}} \\ $$

Commented by behi83417@gmail.com last updated on 26/Oct/18

$${vety}\:{beautiful}\:{question}! \\ $$

Commented by MJS last updated on 26/Oct/18

this is related to a former question, couldn′t find it right now. the inscribed circle always exists but the circumcircle sometimes degenerates to a common tangent or even a circle touching the 3 given from outside (imagine 2 big circles and the 3^(rd) one small enough to fit in the “gap” between them)

$$\mathrm{this}\:\mathrm{is}\:\mathrm{related}\:\mathrm{to}\:\mathrm{a}\:\mathrm{former}\:\mathrm{question},\:\mathrm{couldn}'\mathrm{t} \\ $$$$\mathrm{find}\:\mathrm{it}\:\mathrm{right}\:\mathrm{now}. \\ $$$$\mathrm{the}\:\mathrm{inscribed}\:\mathrm{circle}\:\mathrm{always}\:\mathrm{exists}\:\mathrm{but}\:\mathrm{the} \\ $$$$\mathrm{circumcircle}\:\mathrm{sometimes}\:\mathrm{degenerates}\:\mathrm{to}\:\mathrm{a} \\ $$$$\mathrm{common}\:\mathrm{tangent}\:\mathrm{or}\:\mathrm{even}\:\mathrm{a}\:\mathrm{circle}\:\mathrm{touching} \\ $$$$\mathrm{the}\:\mathrm{3}\:\mathrm{given}\:\mathrm{from}\:\mathrm{outside}\:\left(\mathrm{imagine}\:\mathrm{2}\:\mathrm{big}\:\mathrm{circles}\right. \\ $$$$\mathrm{and}\:\mathrm{the}\:\mathrm{3}^{\mathrm{rd}} \:\mathrm{one}\:\mathrm{small}\:\mathrm{enough}\:\mathrm{to}\:\mathrm{fit}\:\mathrm{in}\:\mathrm{the} \\ $$$$\left.“\mathrm{gap}''\:\mathrm{between}\:\mathrm{them}\right) \\ $$

Commented by MJS last updated on 26/Oct/18

I once downloaded my solution as an image, here it is (sorry can′t change it so it doesn′t fit exactly here but it might help)

$$\mathrm{I}\:\mathrm{once}\:\mathrm{downloaded}\:\mathrm{my}\:\mathrm{solution}\:\mathrm{as}\:\mathrm{an}\:\mathrm{image},\: \\ $$$$\mathrm{here}\:\mathrm{it}\:\mathrm{is}\:\left(\mathrm{sorry}\:\mathrm{can}'\mathrm{t}\:\mathrm{change}\:\mathrm{it}\:\mathrm{so}\:\mathrm{it}\:\mathrm{doesn}'\mathrm{t}\right. \\ $$$$\left.\mathrm{fit}\:\mathrm{exactly}\:\mathrm{here}\:\mathrm{but}\:\mathrm{it}\:\mathrm{might}\:\mathrm{help}\right) \\ $$

Commented by MJS last updated on 26/Oct/18

Commented by MrW3 last updated on 26/Oct/18

This question is indeed derived from the question #37209 from ajfour sir. The given formulas for R and r are simple, but not easy to prove. Can we find an easy way for the proof?

$${This}\:{question}\:{is}\:{indeed}\:{derived}\:{from} \\ $$$${the}\:{question}\:#\mathrm{37209}\:{from}\:{ajfour}\:{sir}. \\ $$$${The}\:{given}\:{formulas}\:{for}\:{R}\:{and}\:{r}\:{are} \\ $$$${simple},\:{but}\:{not}\:{easy}\:{to}\:{prove}.\:{Can}\:{we} \\ $$$${find}\:{an}\:{easy}\:{way}\:{for}\:{the}\:{proof}? \\ $$

Answered by ajfour last updated on 27/Oct/18

Σ(√(R[R−(a+b)]ab))=(√((a+b+c)abc)) cant solve for R !

$$\Sigma\sqrt{{R}\left[{R}−\left({a}+{b}\right)\right]{ab}}=\sqrt{\left({a}+{b}+{c}\right){abc}} \\ $$$${cant}\:{solve}\:{for}\:{R}\:! \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply