Question Number 47179 by peter frank last updated on 05/Nov/18

Answered by tanmay.chaudhury50@gmail.com last updated on 06/Nov/18
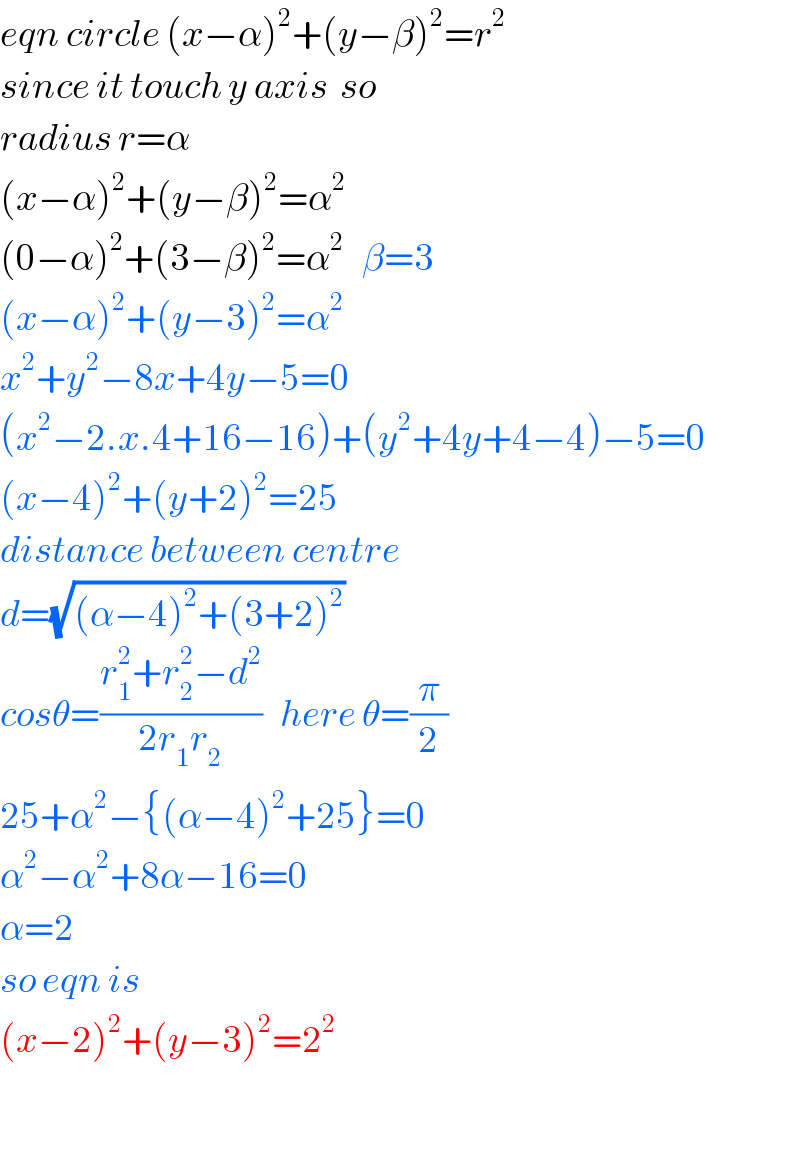
$${eqn}\:{circle}\:\left({x}−\alpha\right)^{\mathrm{2}} +\left({y}−\beta\right)^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$${since}\:{it}\:{touch}\:{y}\:{axis}\:\:{so} \\ $$$${radius}\:{r}=\alpha \\ $$$$\left({x}−\alpha\right)^{\mathrm{2}} +\left({y}−\beta\right)^{\mathrm{2}} =\alpha^{\mathrm{2}} \\ $$$$\left(\mathrm{0}−\alpha\right)^{\mathrm{2}} +\left(\mathrm{3}−\beta\right)^{\mathrm{2}} =\alpha^{\mathrm{2}} \:\:\:\beta=\mathrm{3} \\ $$$$\left({x}−\alpha\right)^{\mathrm{2}} +\left({y}−\mathrm{3}\right)^{\mathrm{2}} =\alpha^{\mathrm{2}} \\ $$$${x}^{\mathrm{2}} +{y}^{\mathrm{2}} −\mathrm{8}{x}+\mathrm{4}{y}−\mathrm{5}=\mathrm{0} \\ $$$$\left({x}^{\mathrm{2}} −\mathrm{2}.{x}.\mathrm{4}+\mathrm{16}−\mathrm{16}\right)+\left({y}^{\mathrm{2}} +\mathrm{4}{y}+\mathrm{4}−\mathrm{4}\right)−\mathrm{5}=\mathrm{0} \\ $$$$\left({x}−\mathrm{4}\right)^{\mathrm{2}} +\left({y}+\mathrm{2}\right)^{\mathrm{2}} =\mathrm{25} \\ $$$${distance}\:{between}\:{centre} \\ $$$${d}=\sqrt{\left(\alpha−\mathrm{4}\right)^{\mathrm{2}} +\left(\mathrm{3}+\mathrm{2}\right)^{\mathrm{2}} }\: \\ $$$${cos}\theta=\frac{{r}_{\mathrm{1}} ^{\mathrm{2}} +{r}_{\mathrm{2}} ^{\mathrm{2}} −{d}^{\mathrm{2}} }{\mathrm{2}{r}_{\mathrm{1}} {r}_{\mathrm{2}} }\:\:\:{here}\:\theta=\frac{\pi}{\mathrm{2}} \\ $$$$\mathrm{25}+\alpha^{\mathrm{2}} −\left\{\left(\alpha−\mathrm{4}\right)^{\mathrm{2}} +\mathrm{25}\right\}=\mathrm{0} \\ $$$$\alpha^{\mathrm{2}} −\alpha^{\mathrm{2}} +\mathrm{8}\alpha−\mathrm{16}=\mathrm{0} \\ $$$$\alpha=\mathrm{2} \\ $$$${so}\:{eqn}\:{is} \\ $$$$\left({x}−\mathrm{2}\right)^{\mathrm{2}} +\left({y}−\mathrm{3}\right)^{\mathrm{2}} =\mathrm{2}^{\mathrm{2}} \\ $$$$ \\ $$
Commented by peter frank last updated on 06/Nov/18
$$\mathrm{pls}\:\mathrm{sir}\:\mathrm{help}\:\mathrm{Qn}\:\mathrm{47185} \\ $$
