Question Number 47401 by Aditya789 last updated on 09/Nov/18

Commented by maxmathsup by imad last updated on 10/Nov/18
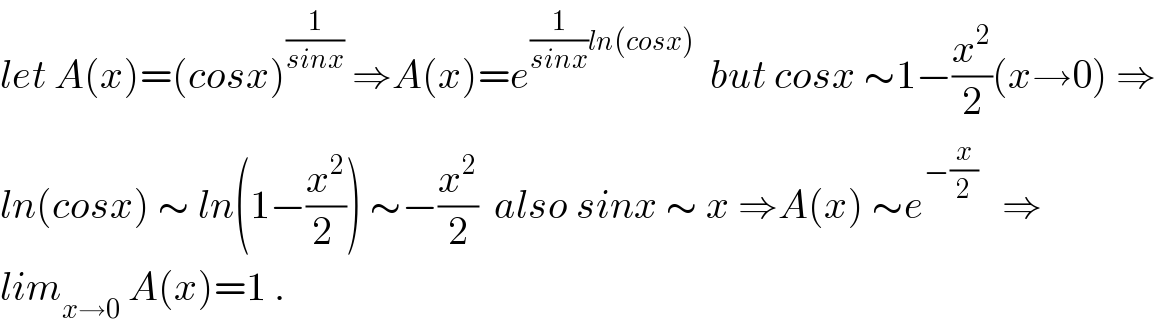
$${let}\:{A}\left({x}\right)=\left({cosx}\right)^{\frac{\mathrm{1}}{{sinx}}} \:\Rightarrow{A}\left({x}\right)={e}^{\frac{\mathrm{1}}{{sinx}}{ln}\left({cosx}\right)} \:\:{but}\:{cosx}\:\sim\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\left({x}\rightarrow\mathrm{0}\right)\:\Rightarrow \\ $$$${ln}\left({cosx}\right)\:\sim\:{ln}\left(\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{2}\:}\right)\:\sim−\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\:\:{also}\:{sinx}\:\sim\:{x}\:\Rightarrow{A}\left({x}\right)\:\sim{e}^{−\frac{{x}}{\mathrm{2}}} \:\:\:\Rightarrow \\ $$$${lim}_{{x}\rightarrow\mathrm{0}} \:{A}\left({x}\right)=\mathrm{1}\:. \\ $$
Answered by ajfour last updated on 09/Nov/18
![= lim_(x→0) [1−(1−cos x)]^(1/sin x) =lim_(x→0) {[1−2sin^2 (x/2)]^(−1/2sin^2 (x/2)) }^(−((2sin^2 (x/2))/(2sin (x/2)cos (x/2)))) = lim_(x→0) e^(−tan (x/2)) = 1 .](https://www.tinkutara.com/question/Q47405.png)
$$=\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left[\mathrm{1}−\left(\mathrm{1}−\mathrm{cos}\:{x}\right)\right]^{\mathrm{1}/\mathrm{sin}\:{x}} \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left\{\left[\mathrm{1}−\mathrm{2sin}\:^{\mathrm{2}} \frac{{x}}{\mathrm{2}}\right]^{−\mathrm{1}/\mathrm{2sin}\:^{\mathrm{2}} \left({x}/\mathrm{2}\right)} \right\}^{−\frac{\mathrm{2sin}\:^{\mathrm{2}} \left({x}/\mathrm{2}\right)}{\mathrm{2sin}\:\left({x}/\mathrm{2}\right)\mathrm{cos}\:\left({x}/\mathrm{2}\right)}} \\ $$$$=\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:{e}^{−\mathrm{tan}\:\left({x}/\mathrm{2}\right)} \:=\:\mathrm{1}\:. \\ $$
Answered by rahul 19 last updated on 11/Nov/18
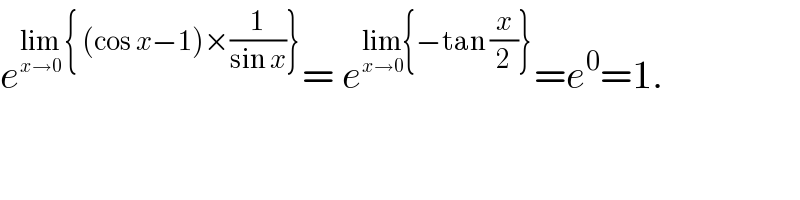
$${e}^{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\left\{\:\left(\mathrm{cos}\:{x}−\mathrm{1}\right)×\frac{\mathrm{1}}{\mathrm{sin}\:{x}}\right\}} =\:{e}^{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left\{−\mathrm{tan}\:\frac{{x}}{\mathrm{2}}\right\}} ={e}^{\mathrm{0}} =\mathrm{1}. \\ $$
