Question Number 47824 by ajfour last updated on 15/Nov/18

Commented by ajfour last updated on 15/Nov/18

Commented by ajfour last updated on 15/Nov/18
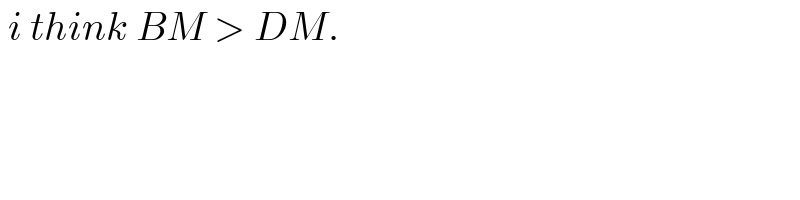
Answered by ajfour last updated on 16/Nov/18
![S(0,−d,H) ; let E be center of sphere: E(0,0,h+R)≡(0,0,z_0 ) let a ray grazing the sphere hit the shadow-curve boundary at (p,q). Eq. of such a ray: r^� =−dj^� +Hk^� +λ(pi^� +(q+d)j^� −Hk^� ) ⊥ distance of v^� from a line r^� =a^� +λb^� , we need to obtain this first. _________________________ let foot of ⊥ from v^� to line be r^� = a^� +λ_0 b^� ⇒ (a^� +λ_0 b^� −v^� ).b^� = 0 ⇒ λ_0 =(((v^� −a^� ).b^� )/(b^� .b^� )) hence ⊥ distance is d = ∣a^� +λ_0 b^� −v^� ∣ = ∣a^� −v^� +((((v^� −a^� ).b^� )/(b^� .b^� )))b^� ∣ ________________________ Now ⊥ distance of ray from center of sphere E is R. So ∣−dj^� +Hk^� +(([dj^� +(h+R−H)k^� ].[pi^� +(q+d)j^� −Hk^� ](pi^� +(q+d)j^� −Hk^� ])/(p^2 +(q+d)^2 +H^2 ))−(h+R)k^� ∣=R ∣−dj^� +Hk^� +(([d(y+d)−H(h+R−H)](xi^� +(y+d)j^� −Hk^� ])/(x^2 +(y+d)^2 +H^2 ))−(h+R)k^� ∣=R _________________________.](https://www.tinkutara.com/question/Q47833.png)
Commented by ajfour last updated on 15/Nov/18

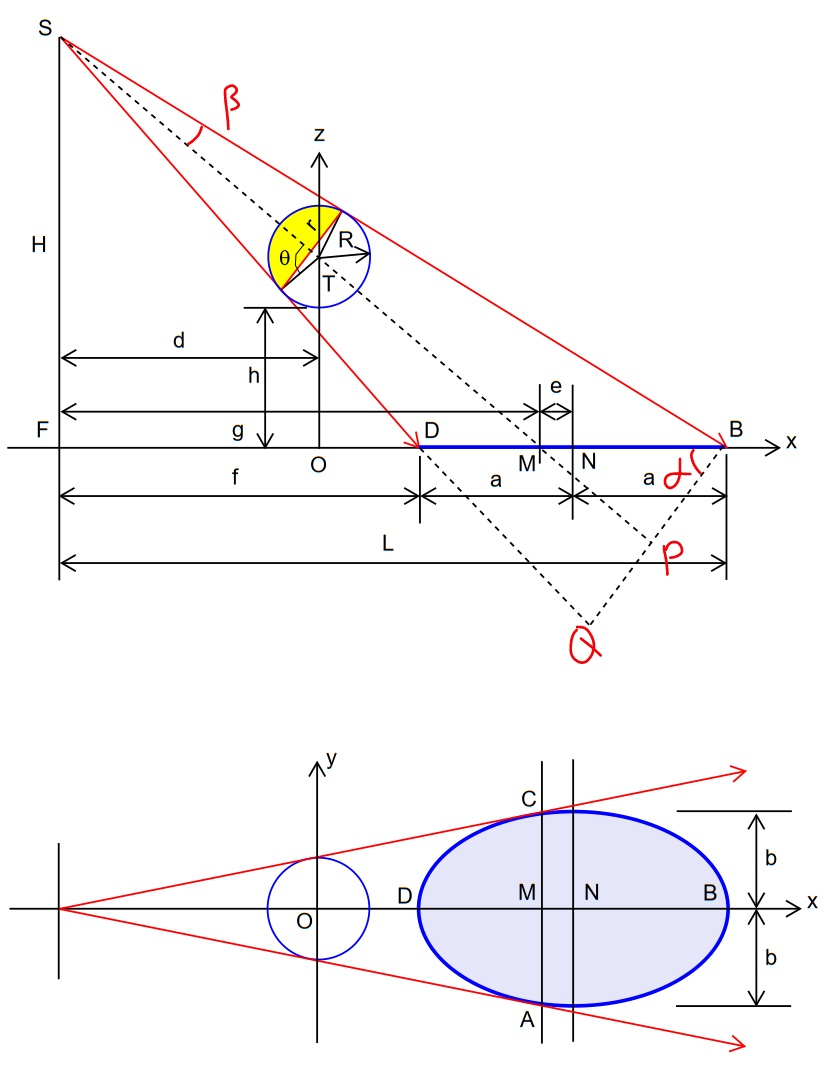
Answered by mr W last updated on 15/Nov/18
Commented by Tawa1 last updated on 16/Nov/18
Commented by mr W last updated on 16/Nov/18

Commented by ajfour last updated on 16/Nov/18
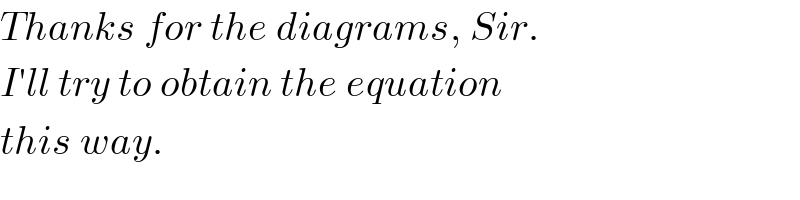
Commented by ajfour last updated on 16/Nov/18

Commented by ajfour last updated on 16/Nov/18
Commented by Tawa1 last updated on 16/Nov/18
Commented by mr W last updated on 16/Nov/18

Commented by mr W last updated on 16/Nov/18

Commented by mr W last updated on 16/Nov/18
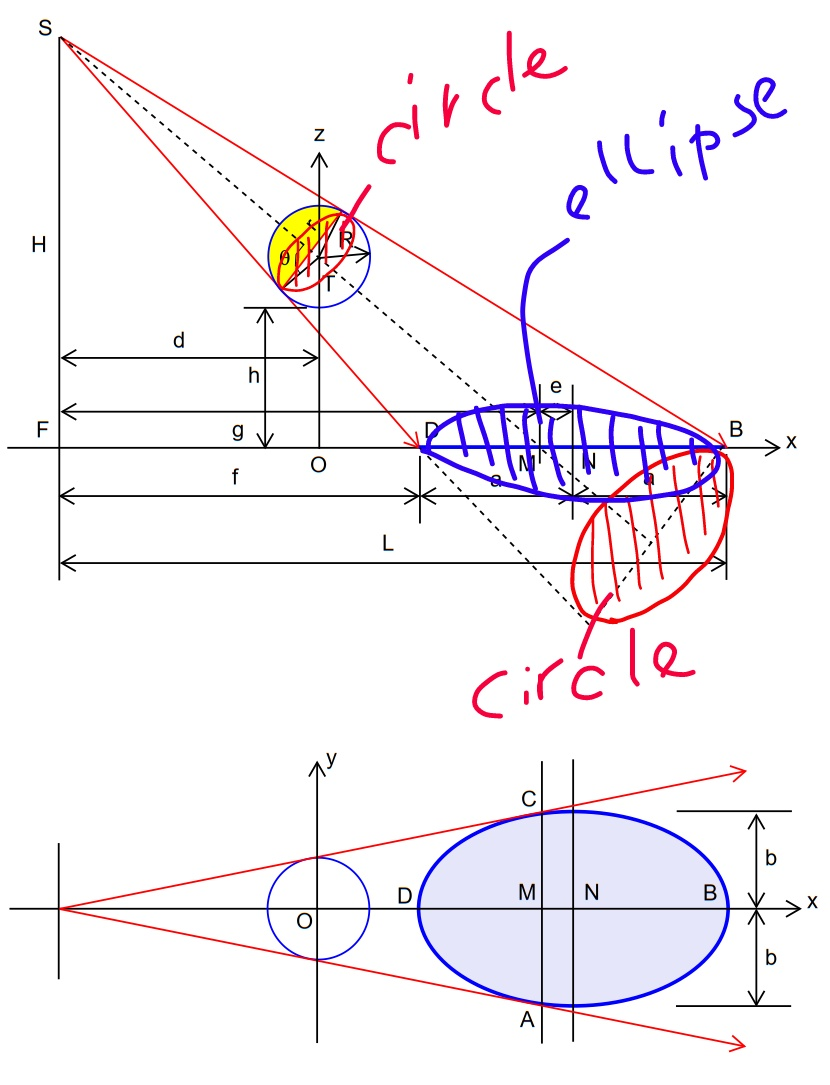
Commented by Tawa1 last updated on 16/Nov/18
Commented by mr W last updated on 16/Nov/18
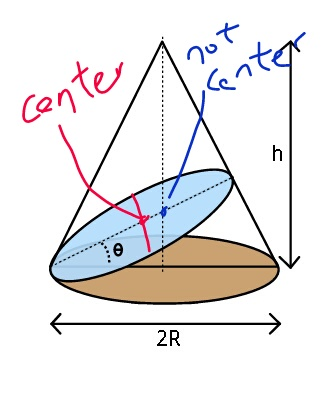
Commented by ajfour last updated on 16/Nov/18

