Question Number 48250 by ajfour last updated on 21/Nov/18
Commented by ajfour last updated on 21/Nov/18

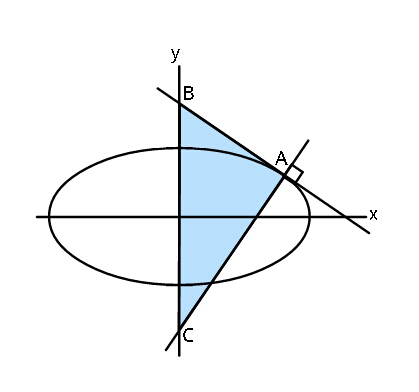
$${Find}\:{minimum}\:{area}\:{of}\:\bigtriangleup{ABC} \\ $$$${in}\:{terms}\:{of}\:{ellipse}\:{parameters} \\ $$$${a}\:{and}\:{b}. \\ $$
Commented by MJS last updated on 21/Nov/18

$$\mathrm{the}\:\mathrm{minimum}\:\mathrm{area}\:\mathrm{is}\:\mathrm{zero}\:\mathrm{when}\:{A}=\begin{pmatrix}{\mathrm{0}}\\{{b}}\end{pmatrix} \\ $$$$\mathrm{using}\:\mathrm{my}\:\mathrm{beloved}\:\mathrm{coordinate}\:\mathrm{method}\:\mathrm{this} \\ $$$$\mathrm{leads}\:\mathrm{to}\:\mathrm{a}\:\mathrm{polynome}\:\mathrm{of}\:\mathrm{degree}\:\mathrm{3}\:\mathrm{which}\:\mathrm{has} \\ $$$$\mathrm{got}\:\mathrm{1}\:\mathrm{real}\:\mathrm{solution}\:\mathrm{for}\:{b}<\frac{{a}}{\mathrm{3}},\:\mathrm{2}\:\mathrm{real}\:\mathrm{solutions} \\ $$$$\mathrm{for}\:{b}=\frac{{a}}{\mathrm{3}}\:\mathrm{and}\:\mathrm{3}\:\mathrm{real}\:\mathrm{solutions}\:\mathrm{for}\:{b}>\frac{{a}}{\mathrm{3}}. \\ $$$${b}<{a}\:\mathrm{for}\:\mathrm{all}\:\mathrm{cases}.\:\mathrm{will}\:\mathrm{post}\:\mathrm{later} \\ $$
Commented by ajfour last updated on 21/Nov/18
$${very}\:{interesting}\:{sir}.. \\ $$
Answered by ajfour last updated on 21/Nov/18
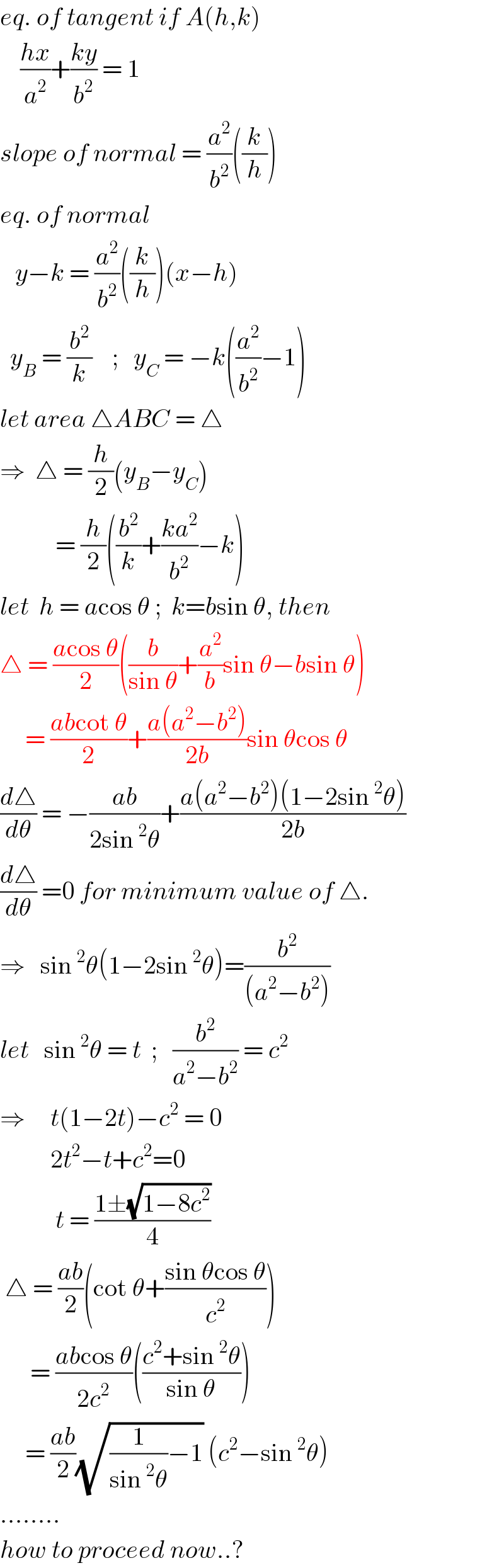
$${eq}.\:{of}\:{tangent}\:{if}\:{A}\left({h},{k}\right) \\ $$$$\:\:\:\:\frac{{hx}}{{a}^{\mathrm{2}} }+\frac{{ky}}{{b}^{\mathrm{2}} }\:=\:\mathrm{1} \\ $$$${slope}\:{of}\:{normal}\:=\:\frac{{a}^{\mathrm{2}} }{{b}^{\mathrm{2}} }\left(\frac{{k}}{{h}}\right) \\ $$$${eq}.\:{of}\:{normal} \\ $$$$\:\:\:{y}−{k}\:=\:\frac{{a}^{\mathrm{2}} }{{b}^{\mathrm{2}} }\left(\frac{{k}}{{h}}\right)\left({x}−{h}\right) \\ $$$$\:\:{y}_{{B}} \:=\:\frac{{b}^{\mathrm{2}} }{{k}}\:\:\:\:;\:\:\:{y}_{{C}} \:=\:−{k}\left(\frac{{a}^{\mathrm{2}} }{{b}^{\mathrm{2}} }−\mathrm{1}\right) \\ $$$${let}\:{area}\:\bigtriangleup{ABC}\:=\:\bigtriangleup \\ $$$$\Rightarrow\:\:\bigtriangleup\:=\:\frac{{h}}{\mathrm{2}}\left({y}_{{B}} −{y}_{{C}} \right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:=\:\frac{{h}}{\mathrm{2}}\left(\frac{{b}^{\mathrm{2}} }{{k}}+\frac{{ka}^{\mathrm{2}} }{{b}^{\mathrm{2}} }−{k}\right) \\ $$$${let}\:\:{h}\:=\:{a}\mathrm{cos}\:\theta\:;\:\:{k}={b}\mathrm{sin}\:\theta,\:{then} \\ $$$$\bigtriangleup\:=\:\frac{{a}\mathrm{cos}\:\theta}{\mathrm{2}}\left(\frac{{b}}{\mathrm{sin}\:\theta}+\frac{{a}^{\mathrm{2}} }{{b}}\mathrm{sin}\:\theta−{b}\mathrm{sin}\:\theta\right) \\ $$$$\:\:\:\:\:=\:\frac{{ab}\mathrm{cot}\:\theta}{\mathrm{2}}+\frac{{a}\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)}{\mathrm{2}{b}}\mathrm{sin}\:\theta\mathrm{cos}\:\theta \\ $$$$\frac{{d}\bigtriangleup}{{d}\theta}\:=\:−\frac{{ab}}{\mathrm{2sin}\:^{\mathrm{2}} \theta}+\frac{{a}\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)\left(\mathrm{1}−\mathrm{2sin}\:^{\mathrm{2}} \theta\right)}{\mathrm{2}{b}} \\ $$$$\frac{{d}\bigtriangleup}{{d}\theta}\:=\mathrm{0}\:{for}\:{minimum}\:{value}\:{of}\:\bigtriangleup. \\ $$$$\Rightarrow\:\:\:\mathrm{sin}\:^{\mathrm{2}} \theta\left(\mathrm{1}−\mathrm{2sin}\:^{\mathrm{2}} \theta\right)=\frac{{b}^{\mathrm{2}} }{\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)} \\ $$$${let}\:\:\:\mathrm{sin}\:^{\mathrm{2}} \theta\:=\:{t}\:\:;\:\:\:\frac{{b}^{\mathrm{2}} }{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }\:=\:{c}^{\mathrm{2}} \\ $$$$\Rightarrow\:\:\:\:\:{t}\left(\mathrm{1}−\mathrm{2}{t}\right)−{c}^{\mathrm{2}} \:=\:\mathrm{0} \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{2}{t}^{\mathrm{2}} −{t}+{c}^{\mathrm{2}} =\mathrm{0} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:{t}\:=\:\frac{\mathrm{1}\pm\sqrt{\mathrm{1}−\mathrm{8}{c}^{\mathrm{2}} }}{\mathrm{4}} \\ $$$$\:\bigtriangleup\:=\:\frac{{ab}}{\mathrm{2}}\left(\mathrm{cot}\:\theta+\frac{\mathrm{sin}\:\theta\mathrm{cos}\:\theta}{{c}^{\mathrm{2}} }\right) \\ $$$$\:\:\:\:\:\:=\:\frac{{ab}\mathrm{cos}\:\theta}{\mathrm{2}{c}^{\mathrm{2}} }\left(\frac{{c}^{\mathrm{2}} +\mathrm{sin}\:^{\mathrm{2}} \theta}{\mathrm{sin}\:\theta}\right) \\ $$$$\:\:\:\:\:=\:\frac{{ab}}{\mathrm{2}}\sqrt{\frac{\mathrm{1}}{\mathrm{sin}\:^{\mathrm{2}} \theta}−\mathrm{1}}\:\left({c}^{\mathrm{2}} −\mathrm{sin}\:^{\mathrm{2}} \theta\right) \\ $$$$…….. \\ $$$${how}\:{to}\:{proceed}\:{now}..? \\ $$
Answered by MJS last updated on 21/Nov/18
![I corrected my method. no 3^(rd) degree polynome A= ((p),(((b/a)(√(a^2 −p^2 )))) ) B= ((0),(((ab)/( (√(a^2 −p^2 ))))) ) C= ((0),((−(((a^2 −b^2 )(√(a^2 −p^2 )))/(ab)))) ) ∣BC∣^2 =(((a^4 −p^2 (a^2 −b^2 ))^2 )/(a^2 b^2 (a^2 −p^2 ))) ∣AC∣^2 =((a^4 −p^2 (a^2 −b^2 ))/b^2 ) ∣AB∣^2 =((p^2 (a^4 −p^2 (a^2 −b^2 )))/(a^2 (a^2 −p^2 ))) Heron′s product gives ((2p(a^4 −p^2 (a^2 −b^2 )))/(ab(√(a^2 −p^2 )))) [and this is zero with p=0] its derivate is ((4(a^2 −b^2 )p^4 −6a^2 (a^2 −b^2 )p^2 +2a^6 )/(ab(√((a^2 −p^2 )^3 )))) zeros are (p>0) p_1 =(a/2)(√(3−((√(a^2 −9b^2 ))/( (√(a^2 −b^2 )))))) p_2 =(a/2)(√(3+((√(a^2 −9b^2 ))/( (√(a^2 −b^2 )))))) p_1 , p_2 are the local extremes with b<(a/3) with b=(a/3) ⇒ p_1 =p_2 and there′s just a horizontal tangent. with b>(a/3) the area is constantly rising with p towards a the area is zero at p=0 and +∞ at p=a](https://www.tinkutara.com/question/Q48287.png)
$$\mathrm{I}\:\mathrm{corrected}\:\mathrm{my}\:\mathrm{method}.\:\mathrm{no}\:\mathrm{3}^{\mathrm{rd}} \:\mathrm{degree}\:\mathrm{polynome} \\ $$$${A}=\begin{pmatrix}{{p}}\\{\frac{{b}}{{a}}\sqrt{{a}^{\mathrm{2}} −{p}^{\mathrm{2}} }}\end{pmatrix}\:\:{B}=\begin{pmatrix}{\mathrm{0}}\\{\frac{{ab}}{\:\sqrt{{a}^{\mathrm{2}} −{p}^{\mathrm{2}} }}}\end{pmatrix}\:\:{C}=\begin{pmatrix}{\mathrm{0}}\\{−\frac{\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)\sqrt{{a}^{\mathrm{2}} −{p}^{\mathrm{2}} }}{{ab}}}\end{pmatrix} \\ $$$$\mid{BC}\mid^{\mathrm{2}} =\frac{\left({a}^{\mathrm{4}} −{p}^{\mathrm{2}} \left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)\right)^{\mathrm{2}} }{{a}^{\mathrm{2}} {b}^{\mathrm{2}} \left({a}^{\mathrm{2}} −{p}^{\mathrm{2}} \right)}\:\:\mid{AC}\mid^{\mathrm{2}} =\frac{{a}^{\mathrm{4}} −{p}^{\mathrm{2}} \left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)}{{b}^{\mathrm{2}} }\:\:\mid{AB}\mid^{\mathrm{2}} =\frac{{p}^{\mathrm{2}} \left({a}^{\mathrm{4}} −{p}^{\mathrm{2}} \left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)\right)}{{a}^{\mathrm{2}} \left({a}^{\mathrm{2}} −{p}^{\mathrm{2}} \right)} \\ $$$$\mathrm{Heron}'\mathrm{s}\:\mathrm{product}\:\mathrm{gives} \\ $$$$\frac{\mathrm{2}{p}\left({a}^{\mathrm{4}} −{p}^{\mathrm{2}} \left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)\right)}{{ab}\sqrt{{a}^{\mathrm{2}} −{p}^{\mathrm{2}} }}\:\:\:\:\:\left[\mathrm{and}\:\mathrm{this}\:\mathrm{is}\:\mathrm{zero}\:\mathrm{with}\:{p}=\mathrm{0}\right] \\ $$$$\mathrm{its}\:\mathrm{derivate}\:\mathrm{is} \\ $$$$\frac{\mathrm{4}\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right){p}^{\mathrm{4}} −\mathrm{6}{a}^{\mathrm{2}} \left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right){p}^{\mathrm{2}} +\mathrm{2}{a}^{\mathrm{6}} }{{ab}\sqrt{\left({a}^{\mathrm{2}} −{p}^{\mathrm{2}} \right)^{\mathrm{3}} }} \\ $$$$\mathrm{zeros}\:\mathrm{are}\:\left({p}>\mathrm{0}\right) \\ $$$${p}_{\mathrm{1}} =\frac{{a}}{\mathrm{2}}\sqrt{\mathrm{3}−\frac{\sqrt{{a}^{\mathrm{2}} −\mathrm{9}{b}^{\mathrm{2}} }}{\:\sqrt{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }}}\:\:{p}_{\mathrm{2}} =\frac{{a}}{\mathrm{2}}\sqrt{\mathrm{3}+\frac{\sqrt{{a}^{\mathrm{2}} −\mathrm{9}{b}^{\mathrm{2}} }}{\:\sqrt{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }}} \\ $$$${p}_{\mathrm{1}} ,\:{p}_{\mathrm{2}} \:\mathrm{are}\:\mathrm{the}\:\mathrm{local}\:\mathrm{extremes}\:\mathrm{with}\:{b}<\frac{{a}}{\mathrm{3}} \\ $$$$\mathrm{with}\:{b}=\frac{{a}}{\mathrm{3}}\:\Rightarrow\:{p}_{\mathrm{1}} ={p}_{\mathrm{2}} \:\mathrm{and}\:\mathrm{there}'\mathrm{s}\:\mathrm{just}\:\mathrm{a}\:\mathrm{horizontal} \\ $$$$\mathrm{tangent}.\:\mathrm{with}\:{b}>\frac{{a}}{\mathrm{3}}\:\mathrm{the}\:\mathrm{area}\:\mathrm{is}\:\mathrm{constantly} \\ $$$$\mathrm{rising}\:\mathrm{with}\:{p}\:\mathrm{towards}\:{a} \\ $$$$\mathrm{the}\:\mathrm{area}\:\mathrm{is}\:\mathrm{zero}\:\mathrm{at}\:{p}=\mathrm{0}\:\mathrm{and}\:+\infty\:\mathrm{at}\:{p}={a} \\ $$
Commented by ajfour last updated on 21/Nov/18

$${thanks}\:{for}\:{the}\:{correct}\:{vision},\:{Sir}. \\ $$
