Question Number 48368 by behi83417@gmail.com last updated on 22/Nov/18
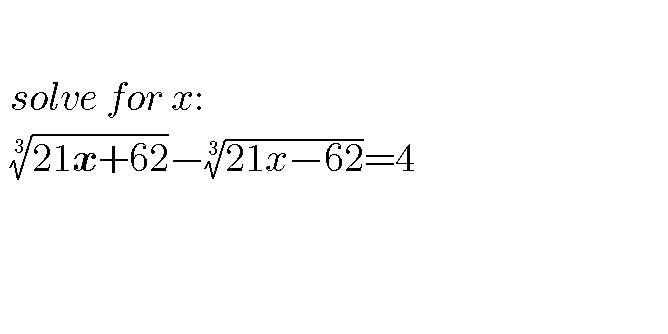
Answered by MJS last updated on 22/Nov/18
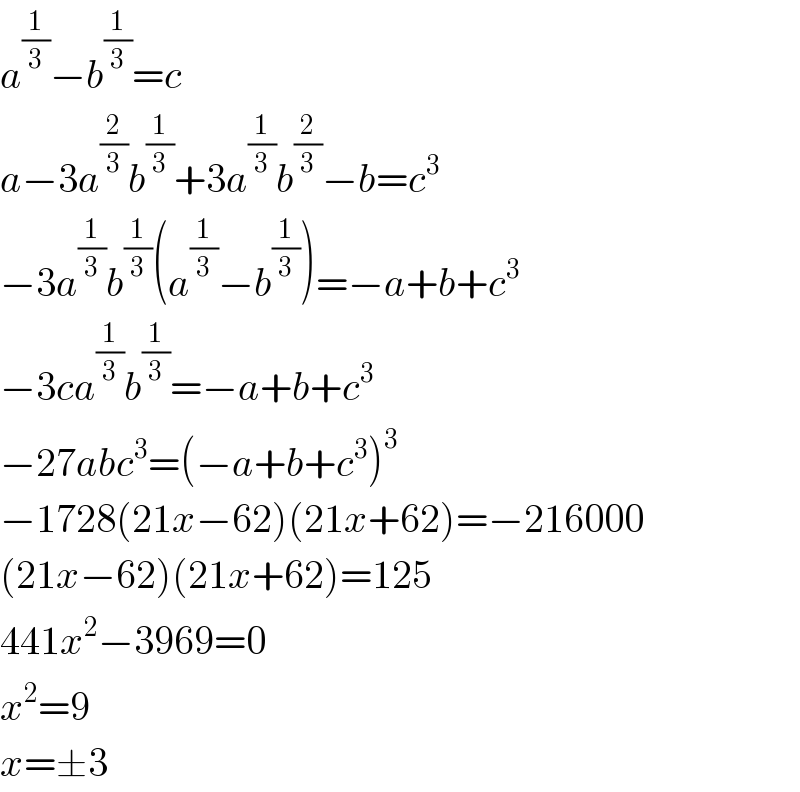
$${a}^{\frac{\mathrm{1}}{\mathrm{3}}} −{b}^{\frac{\mathrm{1}}{\mathrm{3}}} ={c} \\ $$$${a}−\mathrm{3}{a}^{\frac{\mathrm{2}}{\mathrm{3}}} {b}^{\frac{\mathrm{1}}{\mathrm{3}}} +\mathrm{3}{a}^{\frac{\mathrm{1}}{\mathrm{3}}} {b}^{\frac{\mathrm{2}}{\mathrm{3}}} −{b}={c}^{\mathrm{3}} \\ $$$$−\mathrm{3}{a}^{\frac{\mathrm{1}}{\mathrm{3}}} {b}^{\frac{\mathrm{1}}{\mathrm{3}}} \left({a}^{\frac{\mathrm{1}}{\mathrm{3}}} −{b}^{\frac{\mathrm{1}}{\mathrm{3}}} \right)=−{a}+{b}+{c}^{\mathrm{3}} \\ $$$$−\mathrm{3}{ca}^{\frac{\mathrm{1}}{\mathrm{3}}} {b}^{\frac{\mathrm{1}}{\mathrm{3}}} =−{a}+{b}+{c}^{\mathrm{3}} \\ $$$$−\mathrm{27}{abc}^{\mathrm{3}} =\left(−{a}+{b}+{c}^{\mathrm{3}} \right)^{\mathrm{3}} \\ $$$$−\mathrm{1728}\left(\mathrm{21}{x}−\mathrm{62}\right)\left(\mathrm{21}{x}+\mathrm{62}\right)=−\mathrm{216000} \\ $$$$\left(\mathrm{21}{x}−\mathrm{62}\right)\left(\mathrm{21}{x}+\mathrm{62}\right)=\mathrm{125} \\ $$$$\mathrm{441}{x}^{\mathrm{2}} −\mathrm{3969}=\mathrm{0} \\ $$$${x}^{\mathrm{2}} =\mathrm{9} \\ $$$${x}=\pm\mathrm{3} \\ $$
Commented by behi83417@gmail.com last updated on 23/Nov/18
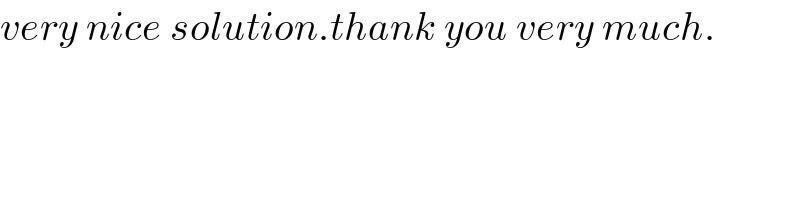
$${very}\:{nice}\:{solution}.{thank}\:{you}\:{very}\:{much}. \\ $$
