Question-51088

Question Number 51088 by ajfour last updated on 23/Dec/18

Commented by ajfour last updated on 23/Dec/18

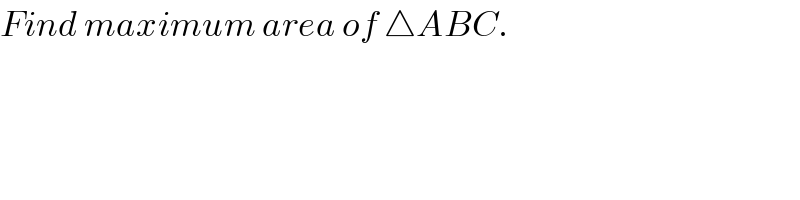
$${Find}\:{maximum}\:{area}\:{of}\:\bigtriangleup{ABC}. \\ $$

Answered by ajfour last updated on 23/Dec/18

For max △, AB= AC △ = ((((√(a^2 +R^2 ))+x))/2)×2(√(R^2 −x^2 )) let x = Rsin θ , then △ = Rcos θ(Rsin θ+d) where d = (√(a^2 +R^2 )) (d△/dθ) = R^2 (1−2sin^2 θ)−Rdsin θ (d△/dθ) = 0 ⇒ 2Rsin^2 θ+dsin θ−R = 0 ⇒ sin θ = −(d/(4R))+((√(d^2 +8R^2 ))/(4R)) △_(max) = R^2 (√(1−sin^2 θ))(sin θ+(d/R)) ...

$$\:\:{For}\:\:{max}\:\bigtriangleup,\:\:{AB}=\:{AC} \\ $$$$\:\:\:\bigtriangleup\:=\:\frac{\left(\sqrt{{a}^{\mathrm{2}} +{R}^{\mathrm{2}} }+{x}\right)}{\mathrm{2}}×\mathrm{2}\sqrt{{R}^{\mathrm{2}} −{x}^{\mathrm{2}} } \\ $$$${let}\:\:\:{x}\:=\:{R}\mathrm{sin}\:\theta\:,\:{then} \\ $$$$\:\:\bigtriangleup\:=\:{R}\mathrm{cos}\:\theta\left({R}\mathrm{sin}\:\theta+{d}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{where}\:\:{d}\:=\:\sqrt{{a}^{\mathrm{2}} +{R}^{\mathrm{2}} } \\ $$$$\frac{{d}\bigtriangleup}{{d}\theta}\:=\:{R}^{\mathrm{2}} \left(\mathrm{1}−\mathrm{2sin}\:^{\mathrm{2}} \theta\right)−{Rd}\mathrm{sin}\:\theta \\ $$$$\frac{{d}\bigtriangleup}{{d}\theta}\:=\:\mathrm{0}\:\:\Rightarrow\:\: \\ $$$$\:\:\:\:\:\:\:\:\mathrm{2}{R}\mathrm{sin}\:^{\mathrm{2}} \theta+{d}\mathrm{sin}\:\theta−{R}\:=\:\mathrm{0} \\ $$$$\Rightarrow\:\:\mathrm{sin}\:\theta\:=\:−\frac{{d}}{\mathrm{4}{R}}+\frac{\sqrt{{d}^{\mathrm{2}} +\mathrm{8}{R}^{\mathrm{2}} }}{\mathrm{4}{R}} \\ $$$$\bigtriangleup_{{max}} \:=\:{R}^{\mathrm{2}} \sqrt{\mathrm{1}−\mathrm{sin}\:^{\mathrm{2}} \theta}\left(\mathrm{sin}\:\theta+\frac{{d}}{{R}}\right) \\ $$$$… \\ $$

Answered by mr W last updated on 24/Dec/18

O=center of circle AO=d=(√(a^2 +R^2 )) ∠BOC=2θ A=(1/2)(2Rsin θ)(d+Rcos θ)=R^2 sin θ((d/R)+cos θ) A=R^2 sin θ(λ+cos θ) with λ=(d/R)=(√(1+(a^2 /R^2 ))) (dA/dθ)=0 cos θ(λ+cos θ)−sin^2 θ=0 2cos^2 θ+λ cos θ−1=0 ⇒cos θ=(((√(λ^2 +8))−λ)/4) ⇒sin θ=((√(8−2λ^2 +2λ(√(λ^2 +8))))/4) ⇒A_(max) =((R^2 (3λ+(√(λ^2 +8)))(√(8−2λ^2 +2λ(√(λ^2 +8)))))/(16)) if point A is on the circle, ⇒d=R, λ=1 A_(max) =((R^2 (3+(√9))(√(8−2+2(√9)))))/(16))=((R^2 6(√(12)))/(16))=((3(√3)R^2 )/4) ⇒area of inscribed equilateral triangle

$${O}={center}\:{of}\:{circle} \\ $$$${AO}={d}=\sqrt{{a}^{\mathrm{2}} +{R}^{\mathrm{2}} } \\ $$$$\angle{BOC}=\mathrm{2}\theta \\ $$$${A}=\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{2}{R}\mathrm{sin}\:\theta\right)\left({d}+{R}\mathrm{cos}\:\theta\right)={R}^{\mathrm{2}} \mathrm{sin}\:\theta\left(\frac{{d}}{{R}}+\mathrm{cos}\:\theta\right) \\ $$$${A}={R}^{\mathrm{2}} \mathrm{sin}\:\theta\left(\lambda+\mathrm{cos}\:\theta\right)\:{with}\:\lambda=\frac{{d}}{{R}}=\sqrt{\mathrm{1}+\frac{{a}^{\mathrm{2}} }{{R}^{\mathrm{2}} }} \\ $$$$\frac{{dA}}{{d}\theta}=\mathrm{0} \\ $$$$\mathrm{cos}\:\theta\left(\lambda+\mathrm{cos}\:\theta\right)−\mathrm{sin}^{\mathrm{2}} \:\theta=\mathrm{0} \\ $$$$\mathrm{2cos}^{\mathrm{2}} \:\theta+\lambda\:\mathrm{cos}\:\theta−\mathrm{1}=\mathrm{0} \\ $$$$\Rightarrow\mathrm{cos}\:\theta=\frac{\sqrt{\lambda^{\mathrm{2}} +\mathrm{8}}−\lambda}{\mathrm{4}} \\ $$$$\Rightarrow\mathrm{sin}\:\theta=\frac{\sqrt{\mathrm{8}−\mathrm{2}\lambda^{\mathrm{2}} +\mathrm{2}\lambda\sqrt{\lambda^{\mathrm{2}} +\mathrm{8}}}}{\mathrm{4}} \\ $$$$\Rightarrow{A}_{{max}} =\frac{{R}^{\mathrm{2}} \left(\mathrm{3}\lambda+\sqrt{\lambda^{\mathrm{2}} +\mathrm{8}}\right)\sqrt{\mathrm{8}−\mathrm{2}\lambda^{\mathrm{2}} +\mathrm{2}\lambda\sqrt{\lambda^{\mathrm{2}} +\mathrm{8}}}}{\mathrm{16}} \\ $$$$ \\ $$$${if}\:{point}\:{A}\:{is}\:{on}\:{the}\:{circle},\:\Rightarrow{d}={R},\:\lambda=\mathrm{1} \\ $$$${A}_{{max}} =\frac{\left.{R}^{\mathrm{2}} \left(\mathrm{3}+\sqrt{\mathrm{9}}\right)\sqrt{\mathrm{8}−\mathrm{2}+\mathrm{2}\sqrt{\mathrm{9}}}\right)}{\mathrm{16}}=\frac{{R}^{\mathrm{2}} \mathrm{6}\sqrt{\mathrm{12}}}{\mathrm{16}}=\frac{\mathrm{3}\sqrt{\mathrm{3}}{R}^{\mathrm{2}} }{\mathrm{4}} \\ $$$$\Rightarrow{area}\:{of}\:{inscribed}\:{equilateral}\:{triangle} \\ $$

Commented by ajfour last updated on 24/Dec/18

$${Thanks}\:{Sir}! \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply