Question-51636

Question Number 51636 by mr W last updated on 29/Dec/18

Commented by tanmay.chaudhury50@gmail.com last updated on 29/Dec/18

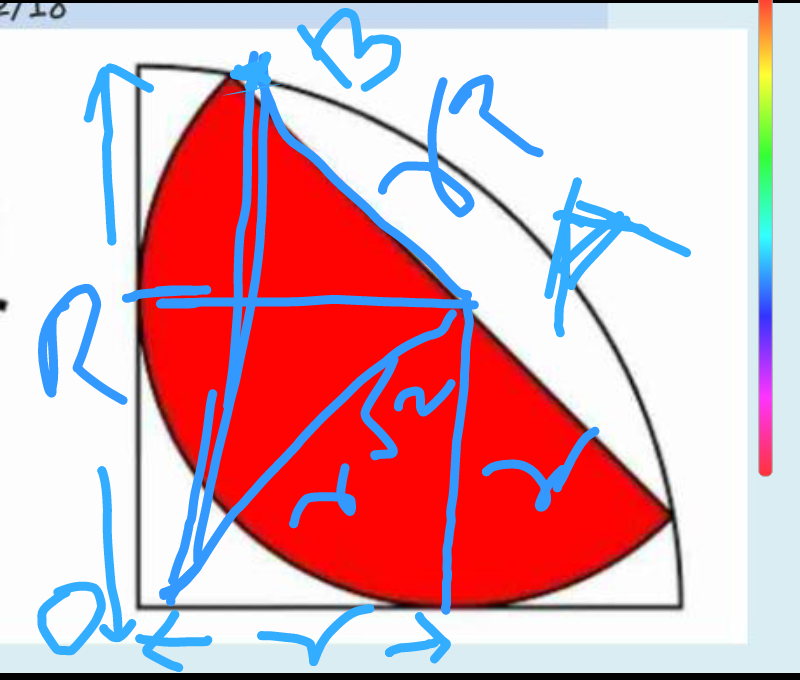
Commented by tanmay.chaudhury50@gmail.com last updated on 29/Dec/18

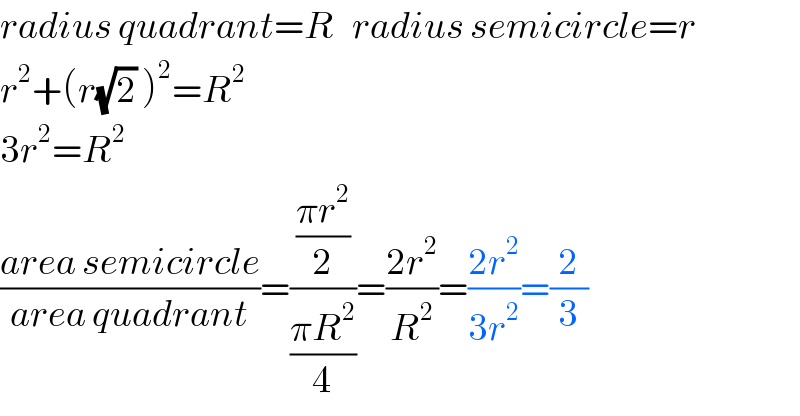
radius quadrant=R radius semicircle=r r^2 +(r(√2) )^2 =R^2 3r^2 =R^2 ((area semicircle)/(area quadrant))=(((πr^2 )/2)/((πR^2 )/4))=((2r^2 )/R^2 )=((2r^2 )/(3r^2 ))=(2/3)

$${radius}\:{quadrant}={R}\:\:\:{radius}\:{semicircle}={r} \\ $$$${r}^{\mathrm{2}} +\left({r}\sqrt{\mathrm{2}}\:\right)^{\mathrm{2}} ={R}^{\mathrm{2}} \\ $$$$\mathrm{3}{r}^{\mathrm{2}} ={R}^{\mathrm{2}} \\ $$$$\frac{{area}\:{semicircle}}{{area}\:{quadrant}}=\frac{\frac{\pi{r}^{\mathrm{2}} }{\mathrm{2}}}{\frac{\pi{R}^{\mathrm{2}} }{\mathrm{4}}}=\frac{\mathrm{2}{r}^{\mathrm{2}} }{{R}^{\mathrm{2}} }=\frac{\mathrm{2}{r}^{\mathrm{2}} }{\mathrm{3}{r}^{\mathrm{2}} }=\frac{\mathrm{2}}{\mathrm{3}} \\ $$

Commented by mr W last updated on 29/Dec/18