Question Number 51876 by Tinkutara last updated on 31/Dec/18

Commented by maxmathsup by imad last updated on 31/Dec/18
![let solve y^′ +2y =f(x) (e) (he) ⇒y^′ +2y =0 ⇒(y^′ /y)=−2 ⇒ln(y)=−2x +k ⇒y(x)=C e^(−2x) mvc method give y^′ =C^′ e^(−2x) −2C e^(−2x) (e) ⇔ C^′ e^(−2x) −2C e^(−2x) +2C e^(−2x) =f(x) ⇒C^′ =f(x) e^(2x) ⇒ C(x) =∫_0 ^x f(t)e^(2t) dt +λ with λ=C(0)=y(0) ⇒ y(x)=( ∫_0 ^x f(t) e^(2t) dt +y(0))e^(−2x) so if y(o)=0 ⇒ y(x) =e^(−2x) ∫_0 ^x f(t) e^(2t) dt ⇒y((3/2)) =e^(−3) ∫_0 ^(3/2) f(t)e^(2t) dt =e^(−3) ∫_0 ^1 e^(2t) dt =(e^(−3) /2)[ e^(2t) ]_0 ^1 =(e^(−3) /2)( e^2 −1) =((e^(−1) −e^(−3) )/2) =((e^2 −1)/(2e^3 )) .](https://www.tinkutara.com/question/Q51888.png)
$${let}\:{solve}\:{y}^{'} \:+\mathrm{2}{y}\:={f}\left({x}\right)\:\:\left({e}\right) \\ $$$$\left({he}\right)\:\Rightarrow{y}^{'} \:+\mathrm{2}{y}\:=\mathrm{0}\:\Rightarrow\frac{{y}^{'} }{{y}}=−\mathrm{2}\:\:\Rightarrow{ln}\left({y}\right)=−\mathrm{2}{x}\:+{k}\:\:\Rightarrow{y}\left({x}\right)={C}\:{e}^{−\mathrm{2}{x}} \\ $$$${mvc}\:{method}\:{give}\:{y}^{'} ={C}^{'} {e}^{−\mathrm{2}{x}} \:−\mathrm{2}{C}\:{e}^{−\mathrm{2}{x}} \\ $$$$\left({e}\right)\:\Leftrightarrow\:{C}^{'} \:{e}^{−\mathrm{2}{x}} \:−\mathrm{2}{C}\:{e}^{−\mathrm{2}{x}} \:+\mathrm{2}{C}\:{e}^{−\mathrm{2}{x}} ={f}\left({x}\right)\:\Rightarrow{C}^{'} ={f}\left({x}\right)\:{e}^{\mathrm{2}{x}} \:\Rightarrow \\ $$$${C}\left({x}\right)\:=\int_{\mathrm{0}} ^{{x}} {f}\left({t}\right){e}^{\mathrm{2}{t}} {dt}\:+\lambda\:\:{with}\:\lambda={C}\left(\mathrm{0}\right)={y}\left(\mathrm{0}\right)\:\Rightarrow \\ $$$${y}\left({x}\right)=\left(\:\int_{\mathrm{0}} ^{{x}} \:{f}\left({t}\right)\:{e}^{\mathrm{2}{t}} {dt}\:+{y}\left(\mathrm{0}\right)\right){e}^{−\mathrm{2}{x}} \:\:{so}\:{if}\:{y}\left({o}\right)=\mathrm{0}\:\Rightarrow \\ $$$${y}\left({x}\right)\:={e}^{−\mathrm{2}{x}} \:\int_{\mathrm{0}} ^{{x}} {f}\left({t}\right)\:{e}^{\mathrm{2}{t}} {dt}\:\Rightarrow{y}\left(\frac{\mathrm{3}}{\mathrm{2}}\right)\:={e}^{−\mathrm{3}} \:\int_{\mathrm{0}} ^{\frac{\mathrm{3}}{\mathrm{2}}} \:{f}\left({t}\right){e}^{\mathrm{2}{t}} \:{dt} \\ $$$$={e}^{−\mathrm{3}} \:\int_{\mathrm{0}} ^{\mathrm{1}} \:{e}^{\mathrm{2}{t}} \:{dt}\:=\frac{{e}^{−\mathrm{3}} }{\mathrm{2}}\left[\:{e}^{\mathrm{2}{t}} \right]_{\mathrm{0}} ^{\mathrm{1}} \:=\frac{{e}^{−\mathrm{3}} }{\mathrm{2}}\left(\:{e}^{\mathrm{2}} −\mathrm{1}\right)\:=\frac{{e}^{−\mathrm{1}} \:−{e}^{−\mathrm{3}} }{\mathrm{2}}\:=\frac{{e}^{\mathrm{2}} \:−\mathrm{1}}{\mathrm{2}{e}^{\mathrm{3}} }\:. \\ $$$$ \\ $$
Commented by Tinkutara last updated on 31/Dec/18
Thanks very much Sir!
Answered by tanmay.chaudhury50@gmail.com last updated on 31/Dec/18
![(dy/dx)+2y=f(x) [(dy/dx)+py=Q I.F=e^(∫pdx) ] intregating factor e^(∫pdx) =e^(∫2dx) =e^(2x) e^(2x) (dy/dx)+e^(2x) ×2y=e^(2x) f(x) e^(2x) ×(d/dx)(y)+y×(d/dx)(e^(2x) )=e^(2x) f(x) (d/dx)[y.e^(2x) ]=e^(2x) f(x) ∫d[ye^(2x) ]=∫e^(2x) f(x)dx ∫_0 ^(3/2) d[ye^(2x) ]=∫_0 ^1 e^(2x) f(x)dx+∫_1 ^(3/2) e^(2x) f(x)dx ∣ye^(2x) ∣_0 ^(3/2) =∫_0 ^1 e^(2x) ×1dx+∫_1 ^(3/2) e^(2x) ×0dx y((3/2))e^3 −y(0)e^(2×0) =∣(e^(2x) /2)∣_0 ^1 y((3/2))e^3 −0×e^0 =((e^2 −1)/2) y((3/2))e^3 =e^2 −1 y((3/2))=((e^2 −1)/(2e^3 )) now pls check....y((3/2))=((e^2 −1)/(2e^3 ))](https://www.tinkutara.com/question/Q51878.png)
$$\frac{{dy}}{{dx}}+\mathrm{2}{y}={f}\left({x}\right)\:\:\:\left[\frac{{dy}}{{dx}}+{py}={Q}\:\:\:\:{I}.{F}={e}^{\int{pdx}} \right] \\ $$$${intregating}\:{factor}\:{e}^{\int{pdx}} ={e}^{\int\mathrm{2}{dx}} ={e}^{\mathrm{2}{x}} \\ $$$${e}^{\mathrm{2}{x}} \frac{{dy}}{{dx}}+{e}^{\mathrm{2}{x}} ×\mathrm{2}{y}={e}^{\mathrm{2}{x}} {f}\left({x}\right) \\ $$$${e}^{\mathrm{2}{x}} ×\frac{{d}}{{dx}}\left({y}\right)+{y}×\frac{{d}}{{dx}}\left({e}^{\mathrm{2}{x}} \right)={e}^{\mathrm{2}{x}} {f}\left({x}\right) \\ $$$$\frac{{d}}{{dx}}\left[{y}.{e}^{\mathrm{2}{x}} \right]={e}^{\mathrm{2}{x}} {f}\left({x}\right) \\ $$$$\int{d}\left[{ye}^{\mathrm{2}{x}} \right]=\int{e}^{\mathrm{2}{x}} {f}\left({x}\right){dx} \\ $$$$ \\ $$$$\int_{\mathrm{0}} ^{\frac{\mathrm{3}}{\mathrm{2}}} {d}\left[{ye}^{\mathrm{2}{x}} \right]=\int_{\mathrm{0}} ^{\mathrm{1}} {e}^{\mathrm{2}{x}} {f}\left({x}\right){dx}+\int_{\mathrm{1}} ^{\frac{\mathrm{3}}{\mathrm{2}}} {e}^{\mathrm{2}{x}} {f}\left({x}\right){dx} \\ $$$$\mid{ye}^{\mathrm{2}{x}} \mid_{\mathrm{0}} ^{\frac{\mathrm{3}}{\mathrm{2}}} \:=\int_{\mathrm{0}} ^{\mathrm{1}} {e}^{\mathrm{2}{x}} ×\mathrm{1}{dx}+\int_{\mathrm{1}} ^{\frac{\mathrm{3}}{\mathrm{2}}} {e}^{\mathrm{2}{x}} ×\mathrm{0}{dx} \\ $$$${y}\left(\frac{\mathrm{3}}{\mathrm{2}}\right){e}^{\mathrm{3}} −{y}\left(\mathrm{0}\right){e}^{\mathrm{2}×\mathrm{0}} =\mid\frac{{e}^{\mathrm{2}{x}} }{\mathrm{2}}\mid_{\mathrm{0}} ^{\mathrm{1}} \\ $$$${y}\left(\frac{\mathrm{3}}{\mathrm{2}}\right){e}^{\mathrm{3}} −\mathrm{0}×{e}^{\mathrm{0}} =\frac{{e}^{\mathrm{2}} −\mathrm{1}}{\mathrm{2}} \\ $$$${y}\left(\frac{\mathrm{3}}{\mathrm{2}}\right){e}^{\mathrm{3}} ={e}^{\mathrm{2}} −\mathrm{1} \\ $$$${y}\left(\frac{\mathrm{3}}{\mathrm{2}}\right)=\frac{{e}^{\mathrm{2}} −\mathrm{1}}{\mathrm{2}{e}^{\mathrm{3}} } \\ $$$$ \\ $$$$ \\ $$$$\boldsymbol{{now}}\:\boldsymbol{{pls}}\:\boldsymbol{{check}}….\boldsymbol{{y}}\left(\frac{\mathrm{3}}{\mathrm{2}}\right)=\frac{\boldsymbol{{e}}^{\mathrm{2}} −\mathrm{1}}{\mathrm{2}\boldsymbol{{e}}^{\mathrm{3}} } \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 31/Dec/18

$$\boldsymbol{{i}}\:\boldsymbol{{have}}\:\boldsymbol{{rectified}}…\boldsymbol{{pls}}\:\boldsymbol{{check}}\:\boldsymbol{{now}}…\boldsymbol{{thsnks}} \\ $$$$\boldsymbol{{for}}\:\boldsymbol{{read}}\:\boldsymbol{{between}}\:\boldsymbol{{line}}\: \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 31/Dec/18
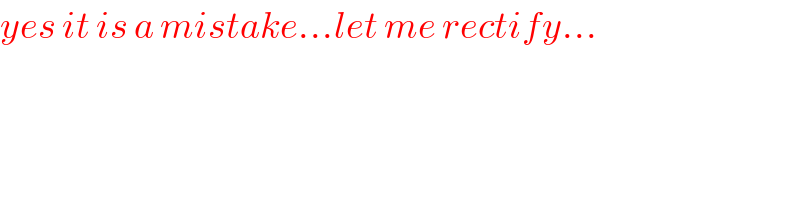
$${yes}\:{it}\:{is}\:{a}\:{mistake}…{let}\:{me}\:{rectify}… \\ $$
Commented by maxmathsup by imad last updated on 31/Dec/18
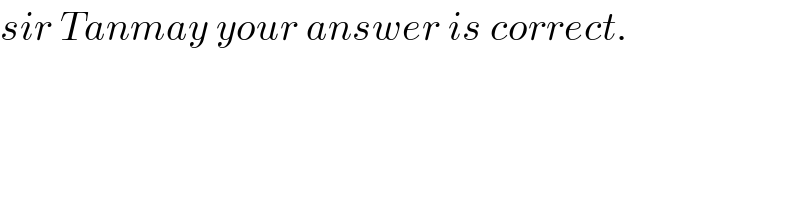
$${sir}\:{Tanmay}\:{your}\:{answer}\:{is}\:{correct}. \\ $$
Commented by Tinkutara last updated on 01/Jan/19
Thank you very much Sir! I got the answer. ��������
