Question Number 52911 by ajfour last updated on 15/Jan/19

Commented by ajfour last updated on 15/Jan/19

$${let}\:{for}\:{example}:\:\:{q}=\mathrm{5},\:{p}=\mathrm{7},\:{r}=\mathrm{4}\:. \\ $$
Commented by MJS last updated on 16/Jan/19
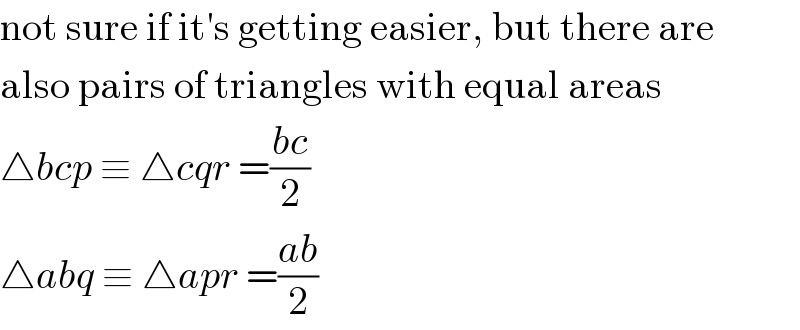
$$\mathrm{not}\:\mathrm{sure}\:\mathrm{if}\:\mathrm{it}'\mathrm{s}\:\mathrm{getting}\:\mathrm{easier},\:\mathrm{but}\:\mathrm{there}\:\mathrm{are} \\ $$$$\mathrm{also}\:\mathrm{pairs}\:\mathrm{of}\:\mathrm{triangles}\:\mathrm{with}\:\mathrm{equal}\:\mathrm{areas} \\ $$$$\bigtriangleup{bcp}\:\equiv\:\bigtriangleup{cqr}\:=\frac{{bc}}{\mathrm{2}} \\ $$$$\bigtriangleup{abq}\:\equiv\:\bigtriangleup{apr}\:=\frac{{ab}}{\mathrm{2}} \\ $$
Answered by mr W last updated on 15/Jan/19
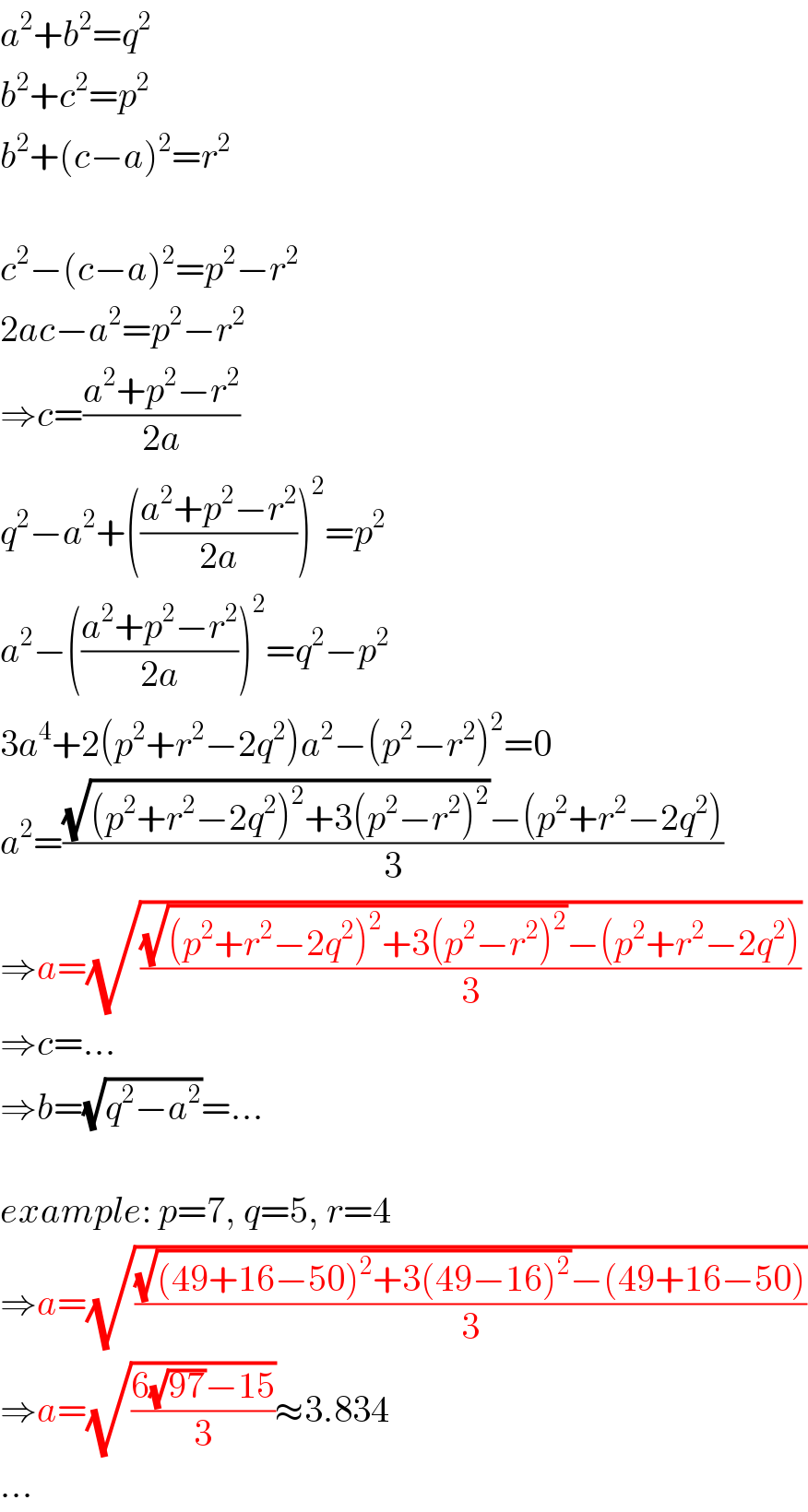
$${a}^{\mathrm{2}} +{b}^{\mathrm{2}} ={q}^{\mathrm{2}} \\ $$$${b}^{\mathrm{2}} +{c}^{\mathrm{2}} ={p}^{\mathrm{2}} \\ $$$${b}^{\mathrm{2}} +\left({c}−{a}\right)^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$$ \\ $$$${c}^{\mathrm{2}} −\left({c}−{a}\right)^{\mathrm{2}} ={p}^{\mathrm{2}} −{r}^{\mathrm{2}} \\ $$$$\mathrm{2}{ac}−{a}^{\mathrm{2}} ={p}^{\mathrm{2}} −{r}^{\mathrm{2}} \\ $$$$\Rightarrow{c}=\frac{{a}^{\mathrm{2}} +{p}^{\mathrm{2}} −{r}^{\mathrm{2}} }{\mathrm{2}{a}} \\ $$$${q}^{\mathrm{2}} −{a}^{\mathrm{2}} +\left(\frac{{a}^{\mathrm{2}} +{p}^{\mathrm{2}} −{r}^{\mathrm{2}} }{\mathrm{2}{a}}\right)^{\mathrm{2}} ={p}^{\mathrm{2}} \\ $$$${a}^{\mathrm{2}} −\left(\frac{{a}^{\mathrm{2}} +{p}^{\mathrm{2}} −{r}^{\mathrm{2}} }{\mathrm{2}{a}}\right)^{\mathrm{2}} ={q}^{\mathrm{2}} −{p}^{\mathrm{2}} \\ $$$$\mathrm{3}{a}^{\mathrm{4}} +\mathrm{2}\left({p}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{q}^{\mathrm{2}} \right){a}^{\mathrm{2}} −\left({p}^{\mathrm{2}} −{r}^{\mathrm{2}} \right)^{\mathrm{2}} =\mathrm{0} \\ $$$${a}^{\mathrm{2}} =\frac{\sqrt{\left({p}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{q}^{\mathrm{2}} \right)^{\mathrm{2}} +\mathrm{3}\left({p}^{\mathrm{2}} −{r}^{\mathrm{2}} \right)^{\mathrm{2}} }−\left({p}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{q}^{\mathrm{2}} \right)}{\mathrm{3}} \\ $$$$\Rightarrow{a}=\sqrt{\frac{\sqrt{\left({p}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{q}^{\mathrm{2}} \right)^{\mathrm{2}} +\mathrm{3}\left({p}^{\mathrm{2}} −{r}^{\mathrm{2}} \right)^{\mathrm{2}} }−\left({p}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{q}^{\mathrm{2}} \right)}{\mathrm{3}}} \\ $$$$\Rightarrow{c}=… \\ $$$$\Rightarrow{b}=\sqrt{{q}^{\mathrm{2}} −{a}^{\mathrm{2}} }=… \\ $$$$ \\ $$$${example}:\:{p}=\mathrm{7},\:{q}=\mathrm{5},\:{r}=\mathrm{4} \\ $$$$\Rightarrow{a}=\sqrt{\frac{\sqrt{\left(\mathrm{49}+\mathrm{16}−\mathrm{50}\right)^{\mathrm{2}} +\mathrm{3}\left(\mathrm{49}−\mathrm{16}\right)^{\mathrm{2}} }−\left(\mathrm{49}+\mathrm{16}−\mathrm{50}\right)}{\mathrm{3}}} \\ $$$$\Rightarrow{a}=\sqrt{\frac{\mathrm{6}\sqrt{\mathrm{97}}−\mathrm{15}}{\mathrm{3}}}\approx\mathrm{3}.\mathrm{834} \\ $$$$… \\ $$
Commented by ajfour last updated on 15/Jan/19
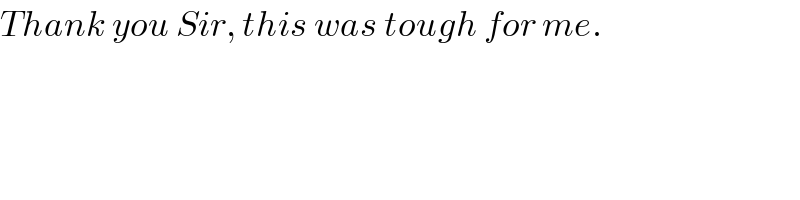
$${Thank}\:{you}\:{Sir},\:{this}\:{was}\:{tough}\:{for}\:{me}. \\ $$
Answered by MJS last updated on 16/Jan/19

$${a}=\sqrt{\frac{−{p}^{\mathrm{2}} +\mathrm{2}{q}^{\mathrm{2}} −{r}^{\mathrm{2}} }{\mathrm{3}}+\frac{\mathrm{2}\sqrt{{p}^{\mathrm{4}} +{q}^{\mathrm{4}} +{r}^{\mathrm{4}} −\left({p}^{\mathrm{2}} {q}^{\mathrm{2}} +{p}^{\mathrm{2}} {r}^{\mathrm{2}} +{q}^{\mathrm{2}} {r}^{\mathrm{2}} \right)}}{\mathrm{3}}} \\ $$$${b}=\sqrt{\frac{{p}^{\mathrm{2}} +{q}^{\mathrm{2}} +{r}^{\mathrm{2}} }{\mathrm{3}}−\frac{\mathrm{2}\sqrt{{p}^{\mathrm{4}} +{q}^{\mathrm{4}} +{r}^{\mathrm{4}} −\left({p}^{\mathrm{2}} {q}^{\mathrm{2}} +{p}^{\mathrm{2}} {r}^{\mathrm{2}} +{q}^{\mathrm{2}} {r}^{\mathrm{2}} \right)}}{\mathrm{3}}} \\ $$$${c}=\sqrt{\frac{\mathrm{2}{p}^{\mathrm{2}} −{q}^{\mathrm{2}} −{r}^{\mathrm{2}} }{\mathrm{3}}+\frac{\mathrm{2}\sqrt{{p}^{\mathrm{4}} +{q}^{\mathrm{4}} +{r}^{\mathrm{4}} −\left({p}^{\mathrm{2}} {q}^{\mathrm{2}} +{p}^{\mathrm{2}} {r}^{\mathrm{2}} +{q}^{\mathrm{2}} {r}^{\mathrm{2}} \right)}}{\mathrm{3}}} \\ $$$$\mathrm{with}\:{p}=\mathrm{7}\:{q}=\mathrm{5}\:{r}=\mathrm{4}\:\mathrm{I}\:\mathrm{get} \\ $$$${a}=\sqrt{−\mathrm{5}+\mathrm{2}\sqrt{\mathrm{97}}}\approx\mathrm{3}.\mathrm{83376} \\ $$$${b}=\sqrt{\mathrm{30}−\mathrm{2}\sqrt{\mathrm{97}}}\approx\mathrm{3}.\mathrm{20972} \\ $$$${c}=\sqrt{\mathrm{19}+\mathrm{2}\sqrt{\mathrm{97}}}\approx\mathrm{6}.\mathrm{22075} \\ $$
