Question-52991

Question Number 52991 by prakash jain last updated on 16/Jan/19

Commented by prakash jain last updated on 16/Jan/19

Correct option is 2. NTA official key has 3. Please feedback. This question is also from 9th Jan JEE main

Answered by tanmay.chaudhury50@gmail.com last updated on 16/Jan/19

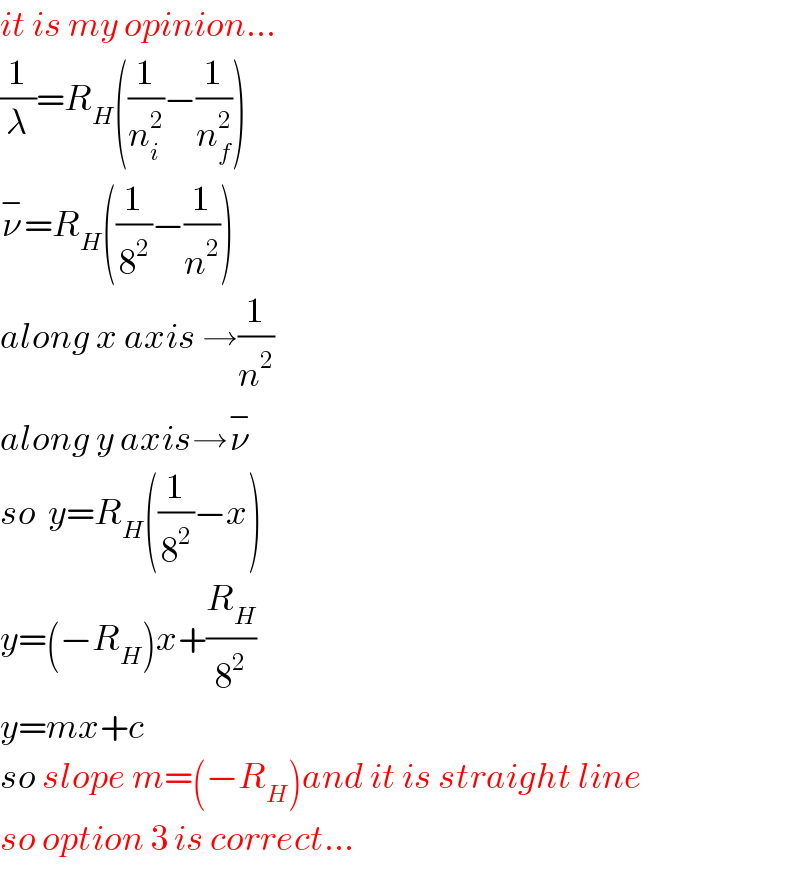
$${it}\:{is}\:{my}\:{opinion}… \\ $$$$\frac{\mathrm{1}}{\lambda}={R}_{{H}} \left(\frac{\mathrm{1}}{{n}_{{i}} ^{\mathrm{2}} }−\frac{\mathrm{1}}{{n}_{{f}} ^{\mathrm{2}} }\right) \\ $$$$\overset{−} {\nu}={R}_{{H}} \left(\frac{\mathrm{1}}{\mathrm{8}^{\mathrm{2}} }−\frac{\mathrm{1}}{{n}^{\mathrm{2}} }\right) \\ $$$${along}\:{x}\:{axis}\:\rightarrow\frac{\mathrm{1}}{{n}^{\mathrm{2}} } \\ $$$${along}\:{y}\:{axis}\rightarrow\overset{−} {\nu} \\ $$$${so}\:\:{y}={R}_{{H}} \left(\frac{\mathrm{1}}{\mathrm{8}^{\mathrm{2}} }−{x}\right) \\ $$$${y}=\left(−{R}_{{H}} \right){x}+\frac{{R}_{{H}} }{\mathrm{8}^{\mathrm{2}} } \\ $$$${y}={mx}+{c} \\ $$$${so}\:{slope}\:{m}=\left(−{R}_{{H}} \right){and}\:{it}\:{is}\:{straight}\:{line} \\ $$$${so}\:{option}\:\mathrm{3}\:{is}\:{correct}… \\ $$

Commented by prakash jain last updated on 16/Jan/19

$$\frac{\mathrm{1}}{\lambda}=\mathrm{R}\left[\frac{\mathrm{1}}{{n}_{{f}} ^{\mathrm{2}} }−\frac{\mathrm{1}}{{n}_{{i}} ^{\mathrm{2}} }\right] \\ $$$$\mathrm{I}\:\mathrm{did}\:\mathrm{a}\:\mathrm{google}\:\mathrm{search}. \\ $$

Commented by prakash jain last updated on 16/Jan/19

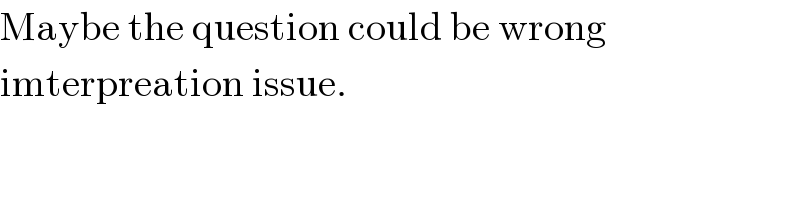
$$\mathrm{Maybe}\:\mathrm{the}\:\mathrm{question}\:\mathrm{could}\:\mathrm{be}\:\mathrm{wrong} \\ $$$$\mathrm{imterpreation}\:\mathrm{issue}. \\ $$

Commented by prakash jain last updated on 16/Jan/19

$$\mathrm{Can}\:\mathrm{you}\:\mathrm{check}\:\mathrm{the}\:\mathrm{last}\:\mathrm{question} \\ $$$$\mathrm{as}\:\mathrm{well}. \\ $$

Commented by prakash jain last updated on 16/Jan/19

Also the question says 8 to n. for example Balmer series emission spectrum is (1/2^2 - 1/n^2). From n to 2. So from 8 to n will mean n has to smaller than 8 for it to be emission spectrum.

Commented by tanmay.chaudhury50@gmail.com last updated on 16/Jan/19

$${pls}\:{upload}\:{question}\:{pls}…{which}\:{question}… \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 16/Jan/19

Commented by tanmay.chaudhury50@gmail.com last updated on 16/Jan/19

Commented by tanmay.chaudhury50@gmail.com last updated on 16/Jan/19

Commented by tanmay.chaudhury50@gmail.com last updated on 16/Jan/19

Commented by prakash jain last updated on 16/Jan/19

$$\mathrm{Question}\:\mathrm{52993} \\ $$

Commented by prakash jain last updated on 16/Jan/19

$$\mathrm{As}\:\mathrm{posted}\:\mathrm{in}\:\mathrm{your}\:\mathrm{material}\:\mathrm{balmer} \\ $$$$\mathrm{series}\:\mathrm{is}\:{n}\:\mathrm{to}\:\mathrm{2}\:\left({see}\:{arrows}\right).\:\mathrm{So}\:\mathrm{the}\:\mathrm{formula}\:\mathrm{is} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }−\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{2}} }. \\ $$$$\mathrm{For}\:\mathrm{8}\:\mathrm{to}\:{n}\:\mathrm{the}\:\mathrm{formula}\:\mathrm{should}\:\mathrm{be} \\ $$$$\frac{\mathrm{1}}{{n}^{\mathrm{2}} }−\frac{\mathrm{1}}{\mathrm{8}^{\mathrm{2}} }. \\ $$$$\mathrm{Am}\:\mathrm{I}\:\mathrm{wrong}? \\ $$

Commented by prakash jain last updated on 16/Jan/19

$${n}\:\mathrm{has}\:\mathrm{to}\:\mathrm{be}\:\mathrm{lower}\:\mathrm{than}\:\mathrm{8}\:\mathrm{for}\:\mathrm{it}\:\mathrm{to}\:\mathrm{be} \\ $$$$\mathrm{emission}\:\mathrm{spectrum}\:\mathrm{and}\:\mathrm{wavelength} \\ $$$$\mathrm{cannot}\:\mathrm{be}\:\mathrm{negative}. \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 16/Jan/19

$${ok}\:{sir}\:{let}\:{me}\:{consult}\:{other}\:{book}… \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 16/Jan/19

$${Q}\mathrm{52993}\:\:{belongs}\:{to}\:{chemistry}…{but}\:{i}\:{am}\:{weak}\:{in}\:{it}.. \\ $$

Terms of Service
Privacy Policy
Contact: info@tinkutara.com