Question Number 54661 by ajfour last updated on 08/Feb/19

Commented by ajfour last updated on 08/Feb/19

$${Given}\:\boldsymbol{{a}}\:{and}\:\boldsymbol{{b}},\:{find}\:\boldsymbol{{c}}. \\ $$
Commented by behi83417@gmail.com last updated on 08/Feb/19

Commented by behi83417@gmail.com last updated on 09/Feb/19
![AN=(√((a+b)^2 −(b−a)^2 ))=2(√(ab)) AP=(√((c+a)^2 −(c−a)^2 ))=2(√(ac)) cosNAB=((2(√(ab)))/(a+b)),cosPAC=((2(√(ac)))/(a+c)) sinNAB=((b−a)/(b+a)),sinPAC=((c−a)/(c+a)) cosCAB=(((a+b)^2 +(a+c)^2 −(b+c)^2 )/(2(a+b)(a+c)))= =((a^2 +ab+ac−bc)/((a+b)(a+c))) CA^� B+NA^� B+PA^� C=90^• ((a^2 +ab+ac−bc)/((a+b)(a+c)))=((2(√(ac)))/((a+b)(a+c)))(b−a)+((2(√(ab)))/((a+b)(a+c)))(c−a) ⇒a^2 +ab+ac−bc=2(b−a)(√(ac))+2(c−a)(√(ab)) ⇒a^2 +ab+ac−bc=2(b−a)(√(ac))+2c(√(ab))−2a(√(ab)) ⇒[a−(b+2(√(ab)))].c−2(b−a)(√a).(√c)+a(a+b+2(√(ab)))=0 △′=a(b−a)^2 −a[a^2 −(b+2(√(ab)))^2 ]= =a[b^2 +a^2 −2ab−[a^2 −(b^2 +4ab+4b(√(ab)))]]= =2ab[b+a+2(√(ab))]=2ab((√a)+(√b))^2 ⇒(√c)=(((b−a)(√a)±((√a)+(√b)).(√(2ab)))/(a−(b+2(√(ab))))) (√c)=(((b−a)(√a)±(a(√(2b))+b(√(2a))))/(a−(b+2(√(ab)))))= =(((b−a)+(√(2b))((√b)+(√a)))/(a−(b+2(√(ab)))))(√a) ⇒c=a.[(((b−a)±(√(2b))((√b)+(√a)))/(a−(b+2(√(ab)))))]^2 . [(b−a)±(√2)(b+(√(ab)))]^2 =(b−a)^2 ±2(√(2b))((√b)−(√a))((√b)+(√a))^2 +2b((√b)+(√a))^2 ]= =(b−a)^2 +2((√a)+(√b))^2 (b±(√(2b))((√b)−(√a))= =(b−a)^2 +2((√b)+(√a))^2 . { (([((√2)+1)b−(√(2ab))])),(([(1−(√2))b+(√(2ab))])) :} [a−(b+2(√(ab)))]^2 =a^2 +b^2 +4ab+4b(√(ab))−2ab−4a(√(ab))= =(a+b)^2 +4(b−a)(√(ab)) ⇒c=a.(((b−a)^2 −2(√b)((√a)+(√b))^2 [(√(2a))−((√2)+1)(√b)])/((a+b)^2 +4(b−a)(√(ab)))) or: c=a.(((b−a)^2 +2(√b)((√a)+(√b))^2 [(√(2a))−((√2)−1)(√b)])/((a+b)^2 +4(b−a)(√(ab)))) .](https://www.tinkutara.com/question/Q54671.png)
$${AN}=\sqrt{\left({a}+{b}\right)^{\mathrm{2}} −\left({b}−{a}\right)^{\mathrm{2}} }=\mathrm{2}\sqrt{{ab}} \\ $$$${AP}=\sqrt{\left({c}+{a}\right)^{\mathrm{2}} −\left({c}−{a}\right)^{\mathrm{2}} }=\mathrm{2}\sqrt{{ac}} \\ $$$${cosNAB}=\frac{\mathrm{2}\sqrt{{ab}}}{{a}+{b}},{cosPAC}=\frac{\mathrm{2}\sqrt{{ac}}}{{a}+{c}} \\ $$$${sinNAB}=\frac{{b}−{a}}{{b}+{a}},{sinPAC}=\frac{{c}−{a}}{{c}+{a}} \\ $$$${cosCAB}=\frac{\left({a}+{b}\right)^{\mathrm{2}} +\left({a}+{c}\right)^{\mathrm{2}} −\left({b}+{c}\right)^{\mathrm{2}} }{\mathrm{2}\left({a}+{b}\right)\left({a}+{c}\right)}= \\ $$$$=\frac{{a}^{\mathrm{2}} +{ab}+{ac}−{bc}}{\left({a}+{b}\right)\left({a}+{c}\right)} \\ $$$${C}\overset{�} {{A}B}+{N}\overset{�} {{A}B}+{P}\overset{�} {{A}C}=\mathrm{90}^{\bullet} \\ $$$$\frac{{a}^{\mathrm{2}} +{ab}+{ac}−{bc}}{\left({a}+{b}\right)\left({a}+{c}\right)}=\frac{\mathrm{2}\sqrt{{ac}}}{\left({a}+{b}\right)\left({a}+{c}\right)}\left({b}−{a}\right)+\frac{\mathrm{2}\sqrt{{ab}}}{\left({a}+{b}\right)\left({a}+{c}\right)}\left({c}−{a}\right) \\ $$$$\Rightarrow{a}^{\mathrm{2}} +{ab}+{ac}−{bc}=\mathrm{2}\left({b}−{a}\right)\sqrt{{ac}}+\mathrm{2}\left({c}−{a}\right)\sqrt{{ab}} \\ $$$$\Rightarrow{a}^{\mathrm{2}} +{ab}+{ac}−{bc}=\mathrm{2}\left({b}−{a}\right)\sqrt{{ac}}+\mathrm{2}{c}\sqrt{{ab}}−\mathrm{2}{a}\sqrt{{ab}} \\ $$$$\Rightarrow\left[{a}−\left({b}+\mathrm{2}\sqrt{{ab}}\right)\right].{c}−\mathrm{2}\left({b}−{a}\right)\sqrt{{a}}.\sqrt{{c}}+{a}\left({a}+{b}+\mathrm{2}\sqrt{{ab}}\right)=\mathrm{0} \\ $$$$\bigtriangleup'={a}\left({b}−{a}\right)^{\mathrm{2}} −{a}\left[{a}^{\mathrm{2}} −\left({b}+\mathrm{2}\sqrt{{ab}}\right)^{\mathrm{2}} \right]= \\ $$$$={a}\left[{b}^{\mathrm{2}} +{a}^{\mathrm{2}} −\mathrm{2}{ab}−\left[{a}^{\mathrm{2}} −\left({b}^{\mathrm{2}} +\mathrm{4}{ab}+\mathrm{4}{b}\sqrt{{ab}}\right)\right]\right]= \\ $$$$=\mathrm{2}{ab}\left[{b}+{a}+\mathrm{2}\sqrt{{ab}}\right]=\mathrm{2}{ab}\left(\sqrt{{a}}+\sqrt{{b}}\right)^{\mathrm{2}} \\ $$$$\Rightarrow\sqrt{{c}}=\frac{\left({b}−{a}\right)\sqrt{{a}}\pm\left(\sqrt{{a}}+\sqrt{{b}}\right).\sqrt{\mathrm{2}{ab}}}{{a}−\left({b}+\mathrm{2}\sqrt{{ab}}\right)} \\ $$$$\sqrt{{c}}=\frac{\left({b}−{a}\right)\sqrt{{a}}\pm\left({a}\sqrt{\mathrm{2}{b}}+{b}\sqrt{\mathrm{2}{a}}\right)}{{a}−\left({b}+\mathrm{2}\sqrt{{ab}}\right)}= \\ $$$$=\frac{\left({b}−{a}\right)+\sqrt{\mathrm{2}{b}}\left(\sqrt{{b}}+\sqrt{{a}}\right)}{{a}−\left({b}+\mathrm{2}\sqrt{{ab}}\right)}\sqrt{{a}} \\ $$$$\Rightarrow{c}={a}.\left[\frac{\left({b}−{a}\right)\pm\sqrt{\mathrm{2}{b}}\left(\sqrt{{b}}+\sqrt{{a}}\right)}{{a}−\left({b}+\mathrm{2}\sqrt{{ab}}\right)}\right]^{\mathrm{2}} . \\ $$$$\left.\left[\left({b}−{a}\right)\pm\sqrt{\mathrm{2}}\left({b}+\sqrt{{ab}}\right)\right]^{\mathrm{2}} =\left({b}−{a}\right)^{\mathrm{2}} \pm\mathrm{2}\sqrt{\mathrm{2}{b}}\left(\sqrt{{b}}−\sqrt{{a}}\right)\left(\sqrt{{b}}+\sqrt{{a}}\right)^{\mathrm{2}} +\mathrm{2}{b}\left(\sqrt{{b}}+\sqrt{{a}}\right)^{\mathrm{2}} \right]= \\ $$$$=\left({b}−{a}\right)^{\mathrm{2}} +\mathrm{2}\left(\sqrt{{a}}+\sqrt{{b}}\right)^{\mathrm{2}} \left({b}\pm\sqrt{\mathrm{2}{b}}\left(\sqrt{{b}}−\sqrt{{a}}\right)=\right. \\ $$$$=\left({b}−{a}\right)^{\mathrm{2}} +\mathrm{2}\left(\sqrt{{b}}+\sqrt{{a}}\right)^{\mathrm{2}} .\begin{cases}{\left[\left(\sqrt{\mathrm{2}}+\mathrm{1}\right){b}−\sqrt{\mathrm{2}{ab}}\right]}\\{\left[\left(\mathrm{1}−\sqrt{\mathrm{2}}\right){b}+\sqrt{\mathrm{2}{ab}}\right]}\end{cases} \\ $$$$\left[{a}−\left({b}+\mathrm{2}\sqrt{{ab}}\right)\right]^{\mathrm{2}} ={a}^{\mathrm{2}} +{b}^{\mathrm{2}} +\mathrm{4}{ab}+\mathrm{4}{b}\sqrt{{ab}}−\mathrm{2}{ab}−\mathrm{4}{a}\sqrt{{ab}}= \\ $$$$=\left({a}+{b}\right)^{\mathrm{2}} +\mathrm{4}\left({b}−{a}\right)\sqrt{{ab}} \\ $$$$\Rightarrow{c}={a}.\frac{\left({b}−{a}\right)^{\mathrm{2}} −\mathrm{2}\sqrt{{b}}\left(\sqrt{{a}}+\sqrt{{b}}\right)^{\mathrm{2}} \left[\sqrt{\mathrm{2}{a}}−\left(\sqrt{\mathrm{2}}+\mathrm{1}\right)\sqrt{{b}}\right]}{\left({a}+{b}\right)^{\mathrm{2}} +\mathrm{4}\left({b}−{a}\right)\sqrt{{ab}}} \\ $$$${or}: \\ $$$${c}={a}.\frac{\left({b}−{a}\right)^{\mathrm{2}} +\mathrm{2}\sqrt{{b}}\left(\sqrt{{a}}+\sqrt{{b}}\right)^{\mathrm{2}} \left[\sqrt{\mathrm{2}{a}}−\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)\sqrt{{b}}\right]}{\left({a}+{b}\right)^{\mathrm{2}} +\mathrm{4}\left({b}−{a}\right)\sqrt{{ab}}}\:. \\ $$
Answered by mr W last updated on 09/Feb/19
![[(√((a+c)^2 −(c−a)^2 ))−(b−a)]^2 +[(√((a+b)^2 −(b−a)^2 ))−(c−a)]^2 =(b+c)^2 [2(√(ac))−(b−a)]^2 +[2(√(ab))−(c−a)]^2 =(b+c)^2 4ac−4(b−a)(√(ac))+b^2 −2ab+a^2 +4ab−4(c−a)(√(ab))−2(a+b)c+a^2 −b^2 =0 ⇒(a−b−2(√(ab)))c−2(b−a)(√(ac))+a(a+b+2(√(ab)))=0 ⇒(√c)=(((a−b)(√a)±(√(2ab(a+b+2(√(ab))))))/(b−a+2(√(ab)))) (take “+” for the upper green circle and “−” for the lower blue circle) ⇒c=a[((a−b±(√(2b(a+b+2(√(ab))))))/(b−a+2(√(ab))))]^2](https://www.tinkutara.com/question/Q54683.png)
$$\left[\sqrt{\left({a}+{c}\right)^{\mathrm{2}} −\left({c}−{a}\right)^{\mathrm{2}} }−\left({b}−{a}\right)\right]^{\mathrm{2}} +\left[\sqrt{\left({a}+{b}\right)^{\mathrm{2}} −\left({b}−{a}\right)^{\mathrm{2}} }−\left({c}−{a}\right)\right]^{\mathrm{2}} =\left({b}+{c}\right)^{\mathrm{2}} \\ $$$$\left[\mathrm{2}\sqrt{{ac}}−\left({b}−{a}\right)\right]^{\mathrm{2}} +\left[\mathrm{2}\sqrt{{ab}}−\left({c}−{a}\right)\right]^{\mathrm{2}} =\left({b}+{c}\right)^{\mathrm{2}} \\ $$$$\mathrm{4}{ac}−\mathrm{4}\left({b}−{a}\right)\sqrt{{ac}}+{b}^{\mathrm{2}} −\mathrm{2}{ab}+{a}^{\mathrm{2}} +\mathrm{4}{ab}−\mathrm{4}\left({c}−{a}\right)\sqrt{{ab}}−\mathrm{2}\left({a}+{b}\right){c}+{a}^{\mathrm{2}} −{b}^{\mathrm{2}} =\mathrm{0} \\ $$$$\Rightarrow\left({a}−{b}−\mathrm{2}\sqrt{{ab}}\right){c}−\mathrm{2}\left({b}−{a}\right)\sqrt{{ac}}+{a}\left({a}+{b}+\mathrm{2}\sqrt{{ab}}\right)=\mathrm{0} \\ $$$$\Rightarrow\sqrt{{c}}=\frac{\left({a}−{b}\right)\sqrt{{a}}\pm\sqrt{\mathrm{2}{ab}\left({a}+{b}+\mathrm{2}\sqrt{{ab}}\right)}}{{b}−{a}+\mathrm{2}\sqrt{{ab}}} \\ $$$$\:\left({take}\:“+''\:{for}\:{the}\:{upper}\:{green}\:{circle}\right. \\ $$$$\left.\:{and}\:“−''\:{for}\:{the}\:{lower}\:{blue}\:{circle}\right) \\ $$$$\Rightarrow{c}={a}\left[\frac{{a}−{b}\pm\sqrt{\mathrm{2}{b}\left({a}+{b}+\mathrm{2}\sqrt{{ab}}\right)}}{{b}−{a}+\mathrm{2}\sqrt{{ab}}}\right]^{\mathrm{2}} \\ $$
Commented by ajfour last updated on 09/Feb/19
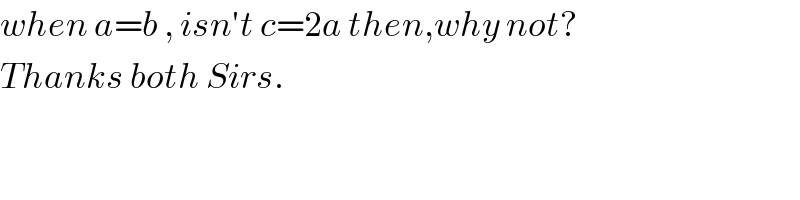
$${when}\:{a}={b}\:,\:{isn}'{t}\:{c}=\mathrm{2}{a}\:{then},{why}\:{not}? \\ $$$${Thanks}\:{both}\:{Sirs}. \\ $$
Commented by mr W last updated on 09/Feb/19

Commented by mr W last updated on 09/Feb/19

Commented by mr W last updated on 09/Feb/19

Commented by ajfour last updated on 09/Feb/19

$${Great}\:{Sir},\:{thanks}\:{for}\:{the}\:{images}. \\ $$
