Question Number 55561 by otchereabdullai@gmail.com last updated on 26/Feb/19

Commented by otchereabdullai@gmail.com last updated on 26/Feb/19

$${prof}\:{W}\:{please}\:{am}\:{learning}\:{this}\:{question} \\ $$$${solved}\:{for}\:{me}\:{on}\:{the}\:\mathrm{21}{st}\:{but}\:{i}\:{dont}\: \\ $$$${understand}\:{this}\:{part}\::\:{if}\:{n}=\mathrm{16}:\alpha_{{n}} =−\mathrm{5}<\mathrm{0} \\ $$$${and}\:{if}\:{n}=\mathrm{18}:\alpha_{{n}} =\mathrm{355}>\mathrm{180} \\ $$$${and}\:{how}\:{you}\:{got}\:{the}\:{n}=\mathrm{17}\:.\: \\ $$$${please}\:{help}\:{me}\:{prof}\:{W} \\ $$
Commented by mr W last updated on 27/Feb/19
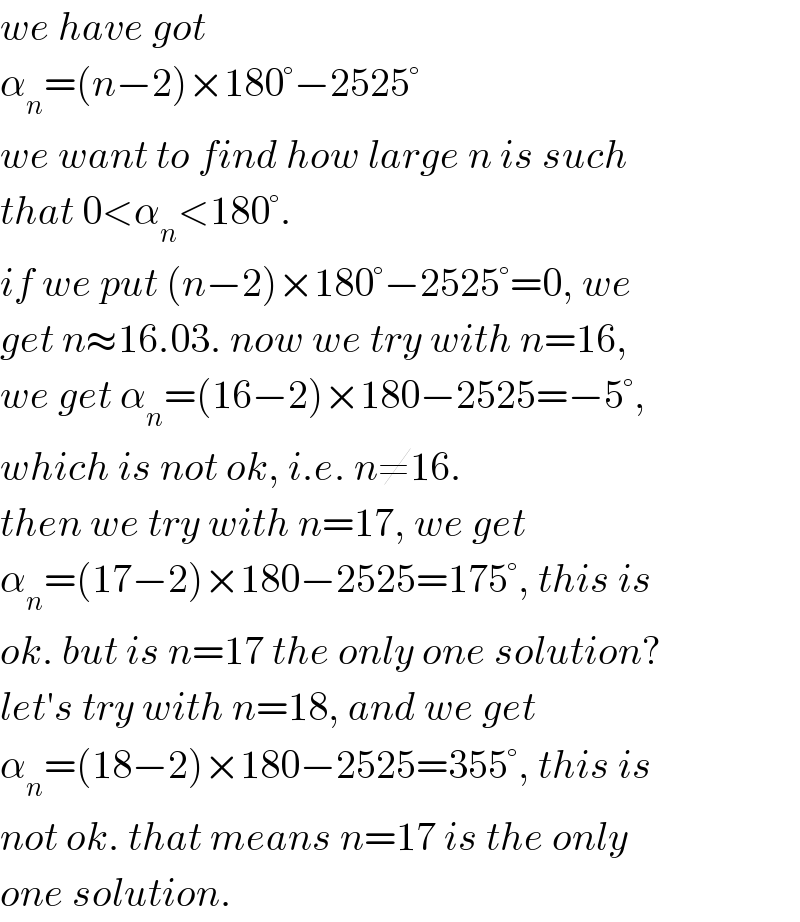
$${we}\:{have}\:{got} \\ $$$$\alpha_{{n}} =\left({n}−\mathrm{2}\right)×\mathrm{180}°−\mathrm{2525}° \\ $$$${we}\:{want}\:{to}\:{find}\:{how}\:{large}\:{n}\:{is}\:{such} \\ $$$${that}\:\mathrm{0}<\alpha_{{n}} <\mathrm{180}°. \\ $$$${if}\:{we}\:{put}\:\left({n}−\mathrm{2}\right)×\mathrm{180}°−\mathrm{2525}°=\mathrm{0},\:{we} \\ $$$${get}\:{n}\approx\mathrm{16}.\mathrm{03}.\:{now}\:{we}\:{try}\:{with}\:{n}=\mathrm{16}, \\ $$$${we}\:{get}\:\alpha_{{n}} =\left(\mathrm{16}−\mathrm{2}\right)×\mathrm{180}−\mathrm{2525}=−\mathrm{5}°, \\ $$$${which}\:{is}\:{not}\:{ok},\:{i}.{e}.\:{n}\neq\mathrm{16}. \\ $$$${then}\:{we}\:{try}\:{with}\:{n}=\mathrm{17},\:{we}\:{get} \\ $$$$\alpha_{{n}} =\left(\mathrm{17}−\mathrm{2}\right)×\mathrm{180}−\mathrm{2525}=\mathrm{175}°,\:{this}\:{is} \\ $$$${ok}.\:{but}\:{is}\:{n}=\mathrm{17}\:{the}\:{only}\:{one}\:{solution}? \\ $$$${let}'{s}\:{try}\:{with}\:{n}=\mathrm{18},\:{and}\:{we}\:{get} \\ $$$$\alpha_{{n}} =\left(\mathrm{18}−\mathrm{2}\right)×\mathrm{180}−\mathrm{2525}=\mathrm{355}°,\:{this}\:{is} \\ $$$${not}\:{ok}.\:{that}\:{means}\:{n}=\mathrm{17}\:{is}\:{the}\:{only} \\ $$$${one}\:{solution}. \\ $$
Commented by otchereabdullai@gmail.com last updated on 26/Feb/19

$${wow}!\:{wow}!\:{In}\:{fact}\:{you}\:{are}\:{an}\:{ideal} \\ $$$${prof}! \\ $$$$ \\ $$
