Question Number 55724 by Kunal12588 last updated on 03/Mar/19
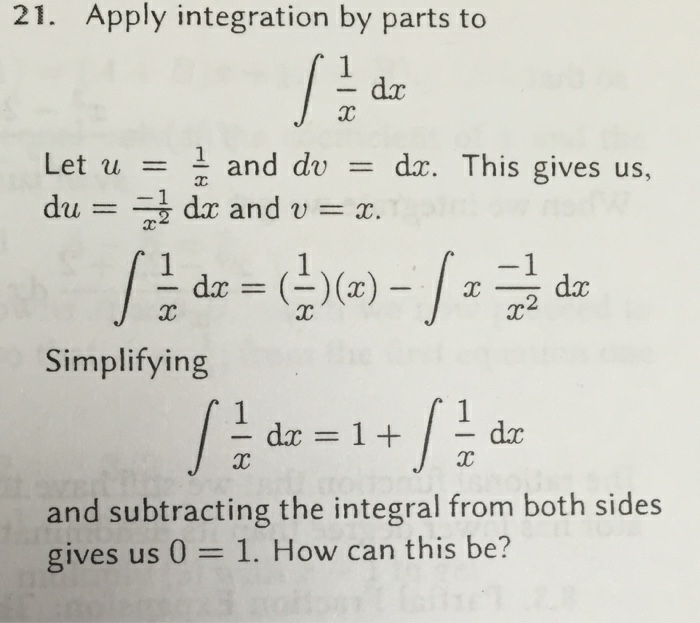
Commented by Kunal12588 last updated on 03/Mar/19

$${this}\:{make}\:{me}\:{stop}\:{learning}\:{calculus}. \\ $$
Commented by mr W last updated on 03/Mar/19
![∫(1/x)dx is not a certain value, but functions. the difference between a ∫(1/x)dx and an other ∫(1/x)dx must be a constant, so ∫(1/x)dx=1+∫(1/x)dx is ok. ∫(1/x)dx=2+∫(1/x)dx is also ok. ∫(1/x)dx=c+∫(1/x)dx is also ok. ∫(1/x)dx=c+∫(1/x)dx=c+ln x+C=ln x+C but if you compare values, you will get ∫_a ^( b) (1/x)dx=[(1/x)x]_a ^b −∫_a ^( b) (−(1/x^2 ))xdx ∫_a ^( b) (1/x)dx=[1]_a ^b +∫_a ^( b) (1/x)dx ∫_a ^( b) (1/x)dx=1−1+∫_a ^( b) (1/x)dx ∫_a ^( b) (1/x)dx=∫_a ^( b) (1/x)dx there is no contradiction! generally it is not wrong when you write ∫f(x)dx=1+∫f(x)dx](https://www.tinkutara.com/question/Q55731.png)
$$\int\frac{\mathrm{1}}{{x}}{dx}\:{is}\:{not}\:{a}\:{certain}\:{value},\:{but}\:{functions}. \\ $$$${the}\:{difference}\:{between}\:{a}\:\int\frac{\mathrm{1}}{{x}}{dx}\:{and} \\ $$$${an}\:{other}\:\int\frac{\mathrm{1}}{{x}}{dx}\:{must}\:{be}\:{a}\:{constant},\:{so} \\ $$$$\int\frac{\mathrm{1}}{{x}}{dx}=\mathrm{1}+\int\frac{\mathrm{1}}{{x}}{dx}\:{is}\:{ok}. \\ $$$$\int\frac{\mathrm{1}}{{x}}{dx}=\mathrm{2}+\int\frac{\mathrm{1}}{{x}}{dx}\:{is}\:{also}\:{ok}. \\ $$$$\int\frac{\mathrm{1}}{{x}}{dx}={c}+\int\frac{\mathrm{1}}{{x}}{dx}\:{is}\:{also}\:{ok}. \\ $$$$\int\frac{\mathrm{1}}{{x}}{dx}={c}+\int\frac{\mathrm{1}}{{x}}{dx}={c}+\mathrm{ln}\:{x}+{C}=\mathrm{ln}\:{x}+{C} \\ $$$$ \\ $$$${but}\:{if}\:{you}\:{compare}\:{values},\:{you}\:{will} \\ $$$${get} \\ $$$$\int_{{a}} ^{\:{b}} \frac{\mathrm{1}}{{x}}{dx}=\left[\frac{\mathrm{1}}{{x}}{x}\right]_{{a}} ^{{b}} −\int_{{a}} ^{\:{b}} \left(−\frac{\mathrm{1}}{{x}^{\mathrm{2}} }\right){xdx} \\ $$$$\int_{{a}} ^{\:{b}} \frac{\mathrm{1}}{{x}}{dx}=\left[\mathrm{1}\right]_{{a}} ^{{b}} +\int_{{a}} ^{\:{b}} \frac{\mathrm{1}}{{x}}{dx} \\ $$$$\int_{{a}} ^{\:{b}} \frac{\mathrm{1}}{{x}}{dx}=\mathrm{1}−\mathrm{1}+\int_{{a}} ^{\:{b}} \frac{\mathrm{1}}{{x}}{dx} \\ $$$$\int_{{a}} ^{\:{b}} \frac{\mathrm{1}}{{x}}{dx}=\int_{{a}} ^{\:{b}} \frac{\mathrm{1}}{{x}}{dx} \\ $$$${there}\:{is}\:{no}\:{contradiction}! \\ $$$$ \\ $$$${generally}\:{it}\:{is}\:{not}\:{wrong}\:{when}\:{you}\:{write} \\ $$$$\int{f}\left({x}\right){dx}=\mathrm{1}+\int{f}\left({x}\right){dx} \\ $$
Commented by Kunal12588 last updated on 03/Mar/19
$${thank}\:{god}.\:{Thank}\:{you}\:{very}\:{much}\:{sir}. \\ $$
Commented by mr W last updated on 03/Mar/19

$${glad}\:{to}\:{see}\:{that}\:{you}\:{do}\:{continue} \\ $$$${learning}\:{calculus}… \\ $$
