Question-55933

Question Number 55933 by Gulay last updated on 06/Mar/19

Commented by Gulay last updated on 06/Mar/19

$$\mathrm{sir}\:\mathrm{could}\:\mathrm{you}\:\mathrm{help}\:\mathrm{me} \\ $$

Answered by tanmay.chaudhury50@gmail.com last updated on 07/Mar/19

a=((√2)−1) a^2 =2−2(√2) +1 a^2 =3−2(√2) now log_(3−2(√2) ) 25 =b (3−2(√2) )^b =5^2 ((√2) −1)^(2b) =5^2 ((√2) −1)^b =5 b=log_(((√2)−1)) 5 now log_(((√2) −1)) (x−2)>log_(((√2) −1)) 5 (x−2)>5←it is wrong so correct is (x−2)<5 x<7

$${a}=\left(\sqrt{\mathrm{2}}−\mathrm{1}\right) \\ $$$${a}^{\mathrm{2}} =\mathrm{2}−\mathrm{2}\sqrt{\mathrm{2}}\:+\mathrm{1} \\ $$$${a}^{\mathrm{2}} =\mathrm{3}−\mathrm{2}\sqrt{\mathrm{2}}\: \\ $$$${now}\:{log}_{\mathrm{3}−\mathrm{2}\sqrt{\mathrm{2}}\:} \:\mathrm{25}\:\:={b} \\ $$$$\left(\mathrm{3}−\mathrm{2}\sqrt{\mathrm{2}}\:\right)^{{b}} =\mathrm{5}^{\mathrm{2}} \\ $$$$\left(\sqrt{\mathrm{2}}\:−\mathrm{1}\right)^{\mathrm{2}{b}} =\mathrm{5}^{\mathrm{2}} \\ $$$$\left(\sqrt{\mathrm{2}}\:−\mathrm{1}\right)^{{b}} =\mathrm{5} \\ $$$${b}={log}_{\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)} \mathrm{5} \\ $$$${now} \\ $$$${log}_{\left(\sqrt{\mathrm{2}}\:−\mathrm{1}\right)} \left({x}−\mathrm{2}\right)>{log}_{\left(\sqrt{\mathrm{2}}\:−\mathrm{1}\right)} \mathrm{5} \\ $$$$\left({x}−\mathrm{2}\right)>\mathrm{5}\leftarrow{it}\:{is}\:{wrong}\:{so}\:{correct}\:{is} \\ $$$$\left({x}−\mathrm{2}\right)<\mathrm{5} \\ $$$$ \\ $$$${x}<\mathrm{7} \\ $$

Commented by Gulay last updated on 06/Mar/19

$$\mathrm{thanks}\:\mathrm{sir} \\ $$

Commented by MJS last updated on 07/Mar/19

$$\mathrm{there}'\mathrm{s}\:\mathrm{a}\:\mathrm{mistake}\:\mathrm{of}\:\mathrm{sign}\:\mathrm{somewhere} \\ $$

Commented by MJS last updated on 07/Mar/19

...it happened between the red and black lines at the end: (√2)−1<1 ⇒ log_((√2)−1) t <0 for t>1 so the > changes to <

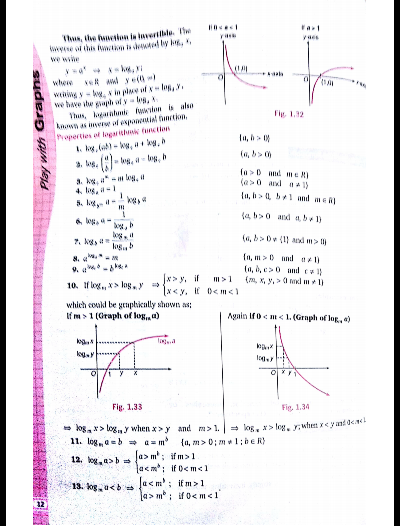
$$…\mathrm{it}\:\mathrm{happened}\:\mathrm{between}\:\mathrm{the}\:\mathrm{red}\:\mathrm{and}\:\mathrm{black}\:\mathrm{lines} \\ $$$$\mathrm{at}\:\mathrm{the}\:\mathrm{end}:\:\sqrt{\mathrm{2}}−\mathrm{1}<\mathrm{1}\:\Rightarrow\:\mathrm{log}_{\sqrt{\mathrm{2}}−\mathrm{1}} {t}\:<\mathrm{0}\:\mathrm{for}\:{t}>\mathrm{1} \\ $$$$\mathrm{so}\:\mathrm{the}\:>\:\mathrm{changes}\:\mathrm{to}\:< \\ $$$$ \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 07/Mar/19

$${yes}\:{sir}\:{you}\:{are}\:{right}…{thank}\:{you}\:{sir}.. \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 07/Mar/19

Answered by MJS last updated on 07/Mar/19

((ln (x−2))/(ln ((√2)−1)))>((ln 25)/(ln (3−2(√2)))) [25=5^2 ; 3−2(√2)=((√2)−1)^2 ] ((ln (x−2))/(ln ((√2)−1)))>((2ln 5)/(2ln ((√(2−1))))) [0<(√2)−1<1 ⇒ ln ((√2)−1)<0] ln (x−2)<ln 5 x−2<5 x<7

$$\frac{\mathrm{ln}\:\left({x}−\mathrm{2}\right)}{\mathrm{ln}\:\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)}>\frac{\mathrm{ln}\:\mathrm{25}}{\mathrm{ln}\:\left(\mathrm{3}−\mathrm{2}\sqrt{\mathrm{2}}\right)} \\ $$$$\:\:\:\:\:\left[\mathrm{25}=\mathrm{5}^{\mathrm{2}} ;\:\mathrm{3}−\mathrm{2}\sqrt{\mathrm{2}}=\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)^{\mathrm{2}} \right] \\ $$$$\frac{\mathrm{ln}\:\left({x}−\mathrm{2}\right)}{\mathrm{ln}\:\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)}>\frac{\mathrm{2ln}\:\mathrm{5}}{\mathrm{2ln}\:\left(\sqrt{\mathrm{2}−\mathrm{1}}\right)} \\ $$$$\:\:\:\:\:\left[\mathrm{0}<\sqrt{\mathrm{2}}−\mathrm{1}<\mathrm{1}\:\Rightarrow\:\mathrm{ln}\:\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)<\mathrm{0}\right] \\ $$$$\mathrm{ln}\:\left({x}−\mathrm{2}\right)<\mathrm{ln}\:\mathrm{5} \\ $$$${x}−\mathrm{2}<\mathrm{5} \\ $$$${x}<\mathrm{7} \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply