Question-55971

Question Number 55971 by bshahid010@gmail.com last updated on 07/Mar/19

Commented by tanmay.chaudhury50@gmail.com last updated on 07/Mar/19

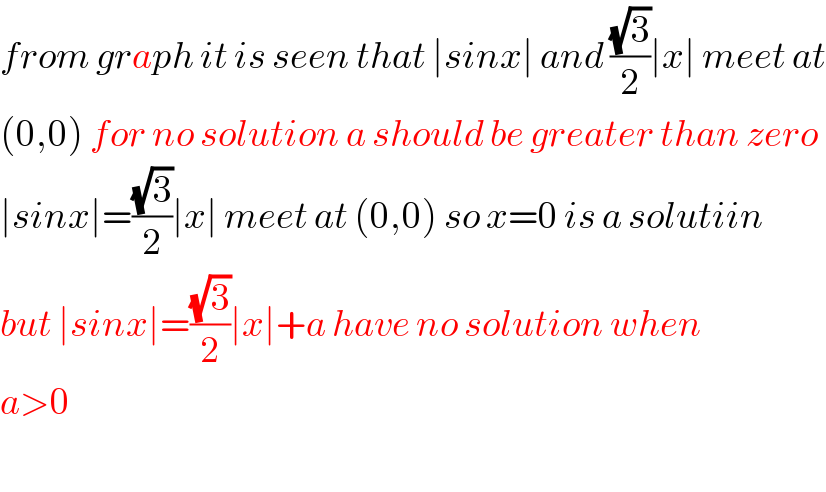
from graph it is seen that ∣sinx∣ and ((√3)/2)∣x∣ meet at (0,0) for no solution a should be greater than zero ∣sinx∣=((√3)/2)∣x∣ meet at (0,0) so x=0 is a solutiin but ∣sinx∣=((√3)/2)∣x∣+a have no solution when a>0

$${from}\:{graph}\:{it}\:{is}\:{seen}\:{that}\:\mid{sinx}\mid\:{and}\:\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mid{x}\mid\:{meet}\:{at} \\ $$$$\left(\mathrm{0},\mathrm{0}\right)\:{for}\:{no}\:{solution}\:{a}\:{should}\:{be}\:{greater}\:{than}\:{zero} \\ $$$$\mid{sinx}\mid=\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mid{x}\mid\:{meet}\:{at}\:\left(\mathrm{0},\mathrm{0}\right)\:{so}\:{x}=\mathrm{0}\:{is}\:{a}\:{solutiin} \\ $$$${but}\:\mid{sinx}\mid=\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mid{x}\mid+{a}\:{have}\:{no}\:{solution}\:{when} \\ $$$${a}>\mathrm{0} \\ $$$$ \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 07/Mar/19

Answered by mr W last updated on 07/Mar/19

Commented by mr W last updated on 07/Mar/19

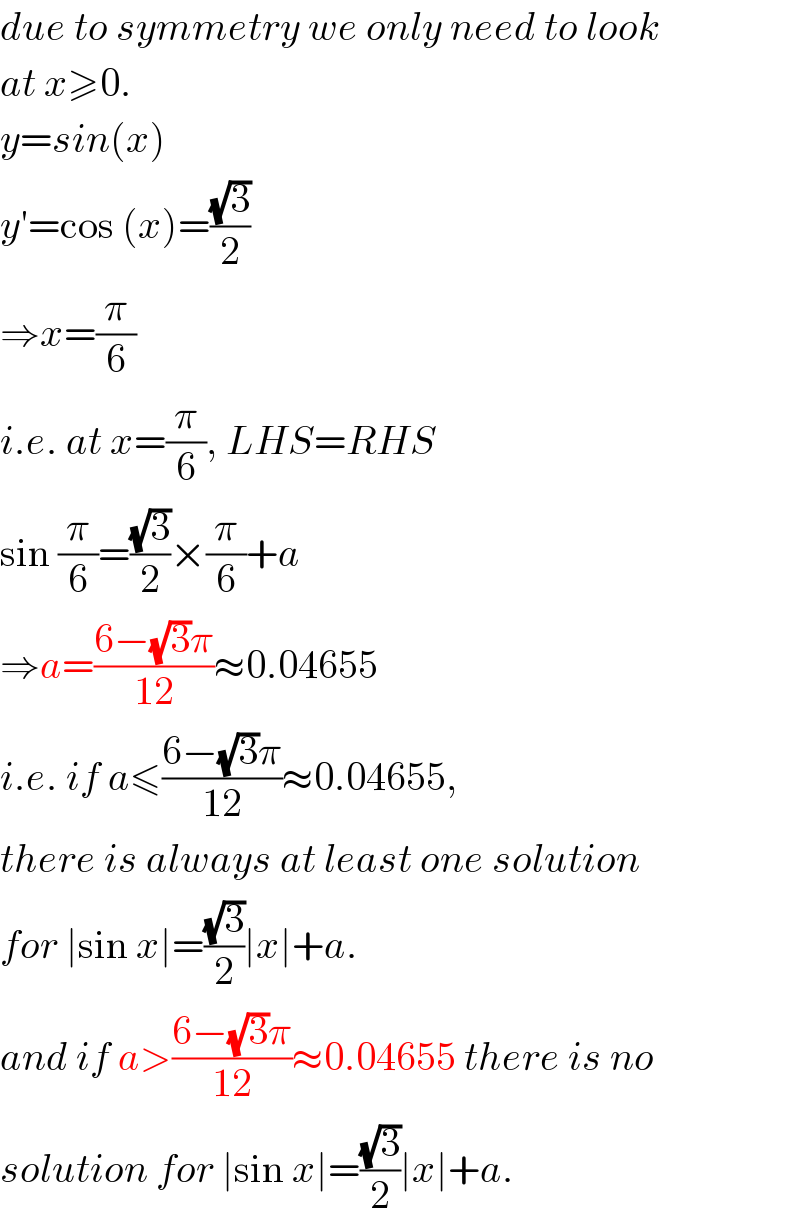
due to symmetry we only need to look at x≥0. y=sin(x) y′=cos (x)=((√3)/2) ⇒x=(π/6) i.e. at x=(π/6), LHS=RHS sin (π/6)=((√3)/2)×(π/6)+a ⇒a=((6−(√3)π)/(12))≈0.04655 i.e. if a≤((6−(√3)π)/(12))≈0.04655, there is always at least one solution for ∣sin x∣=((√3)/2)∣x∣+a. and if a>((6−(√3)π)/(12))≈0.04655 there is no solution for ∣sin x∣=((√3)/2)∣x∣+a.

$${due}\:{to}\:{symmetry}\:{we}\:{only}\:{need}\:{to}\:{look} \\ $$$${at}\:{x}\geqslant\mathrm{0}. \\ $$$${y}={sin}\left({x}\right) \\ $$$${y}'=\mathrm{cos}\:\left({x}\right)=\frac{\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$$\Rightarrow{x}=\frac{\pi}{\mathrm{6}} \\ $$$${i}.{e}.\:{at}\:{x}=\frac{\pi}{\mathrm{6}},\:{LHS}={RHS} \\ $$$$\mathrm{sin}\:\frac{\pi}{\mathrm{6}}=\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}×\frac{\pi}{\mathrm{6}}+{a} \\ $$$$\Rightarrow{a}=\frac{\mathrm{6}−\sqrt{\mathrm{3}}\pi}{\mathrm{12}}\approx\mathrm{0}.\mathrm{04655} \\ $$$${i}.{e}.\:{if}\:{a}\leqslant\frac{\mathrm{6}−\sqrt{\mathrm{3}}\pi}{\mathrm{12}}\approx\mathrm{0}.\mathrm{04655}, \\ $$$${there}\:{is}\:{always}\:{at}\:{least}\:{one}\:{solution} \\ $$$${for}\:\mid\mathrm{sin}\:{x}\mid=\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mid{x}\mid+{a}. \\ $$$${and}\:{if}\:{a}>\frac{\mathrm{6}−\sqrt{\mathrm{3}}\pi}{\mathrm{12}}\approx\mathrm{0}.\mathrm{04655}\:{there}\:{is}\:{no} \\ $$$${solution}\:{for}\:\mid\mathrm{sin}\:{x}\mid=\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mid{x}\mid+{a}. \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 07/Mar/19