Menu Close

Question-56289

Tinku Tara 0 Comments
Pin




Question Number 56289 by mr W last updated on 13/Mar/19
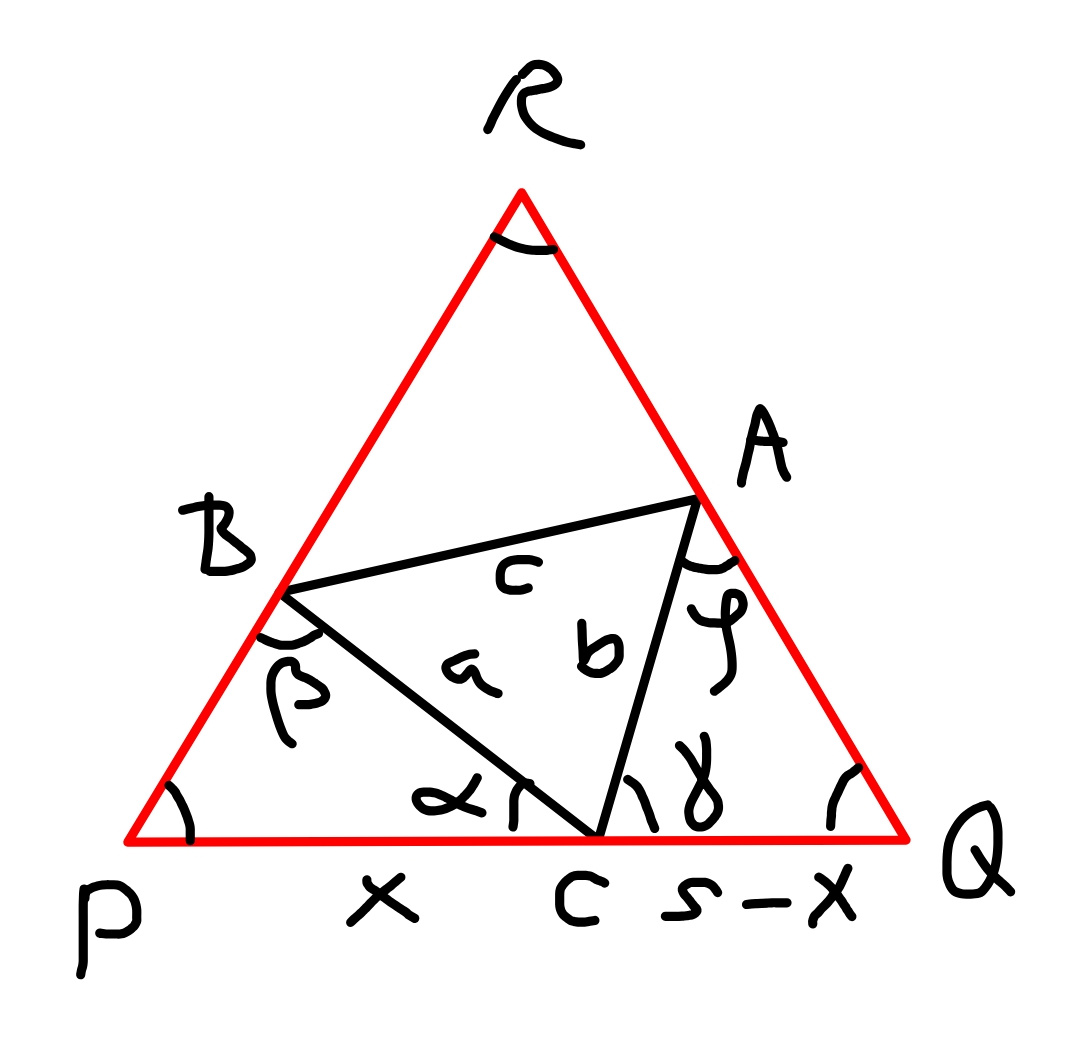
Commented by mr W last updated on 13/Mar/19
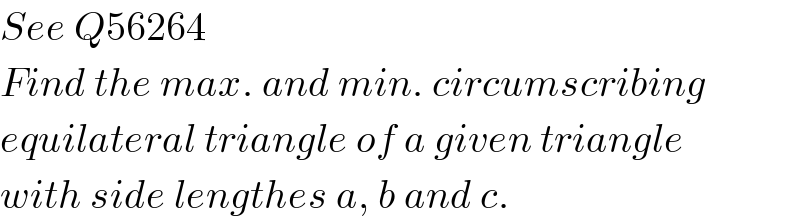
See Q56264 Find the max. and min. circumscribing equilateral triangle of a given triangle with side lengthes a, b and c.
$${See}\:{Q}\mathrm{56264} \\ $$$${Find}\:{the}\:{max}.\:{and}\:{min}.\:{circumscribing} \\ $$$${equilateral}\:{triangle}\:{of}\:{a}\:{given}\:{triangle} \\ $$$${with}\:{side}\:{lengthes}\:{a},\:{b}\:{and}\:{c}. \\ $$
Commented by ajfour last updated on 13/Mar/19
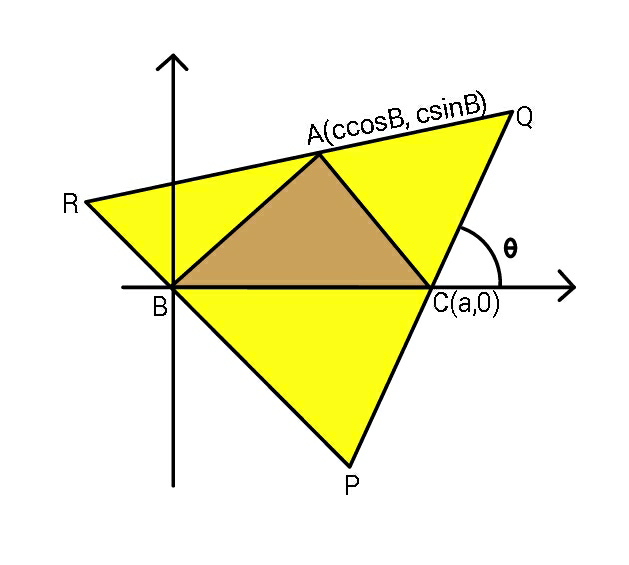
Commented by MJS last updated on 13/Mar/19
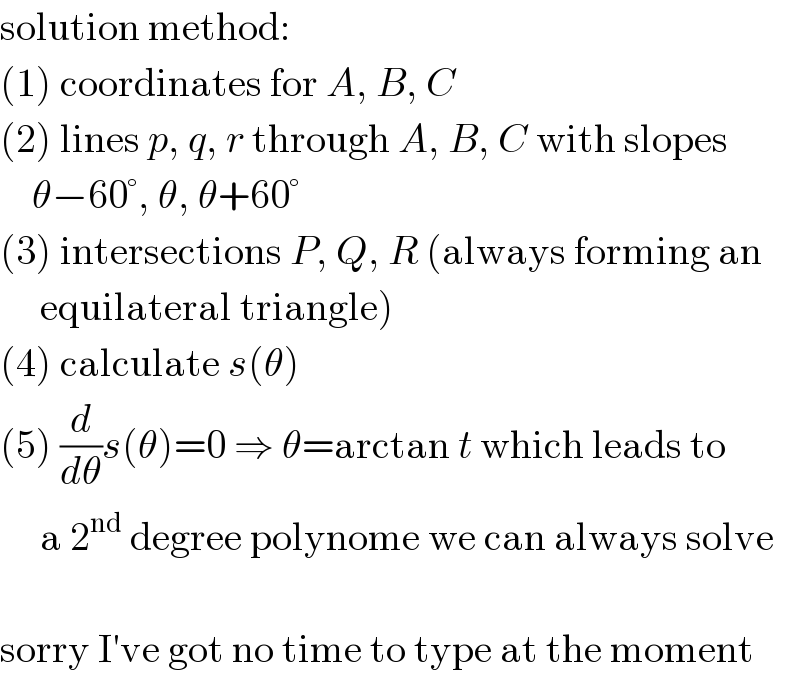
solution method: (1) coordinates for A, B, C (2) lines p, q, r through A, B, C with slopes θ−60°, θ, θ+60° (3) intersections P, Q, R (always forming an equilateral triangle) (4) calculate s(θ) (5) (d/dθ)s(θ)=0 ⇒ θ=arctan t which leads to a 2^(nd) degree polynome we can always solve sorry I′ve got no time to type at the moment
$$\mathrm{solution}\:\mathrm{method}: \\ $$$$\left(\mathrm{1}\right)\:\mathrm{coordinates}\:\mathrm{for}\:{A},\:{B},\:{C} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{lines}\:{p},\:{q},\:{r}\:\mathrm{through}\:{A},\:{B},\:{C}\:\mathrm{with}\:\mathrm{slopes} \\ $$$$\:\:\:\:\theta−\mathrm{60}°,\:\theta,\:\theta+\mathrm{60}° \\ $$$$\left(\mathrm{3}\right)\:\mathrm{intersections}\:{P},\:{Q},\:{R}\:\left(\mathrm{always}\:\mathrm{forming}\:\mathrm{an}\right. \\ $$$$\left.\:\:\:\:\:\mathrm{equilateral}\:\mathrm{triangle}\right) \\ $$$$\left(\mathrm{4}\right)\:\mathrm{calculate}\:{s}\left(\theta\right) \\ $$$$\left(\mathrm{5}\right)\:\frac{{d}}{{d}\theta}{s}\left(\theta\right)=\mathrm{0}\:\Rightarrow\:\theta=\mathrm{arctan}\:{t}\:\mathrm{which}\:\mathrm{leads}\:\mathrm{to} \\ $$$$\:\:\:\:\:\mathrm{a}\:\mathrm{2}^{\mathrm{nd}} \:\mathrm{degree}\:\mathrm{polynome}\:\mathrm{we}\:\mathrm{can}\:\mathrm{always}\:\mathrm{solve} \\ $$$$ \\ $$$$\mathrm{sorry}\:\mathrm{I}'\mathrm{ve}\:\mathrm{got}\:\mathrm{no}\:\mathrm{time}\:\mathrm{to}\:\mathrm{type}\:\mathrm{at}\:\mathrm{the}\:\mathrm{moment} \\ $$
Commented by mr W last updated on 14/Mar/19
perfectly done!
$${perfectly}\:{done}! \\ $$
Commented by ajfour last updated on 14/Mar/19
thank you Sir.
$${thank}\:{you}\:{Sir}. \\ $$
Commented by mr W last updated on 15/Mar/19
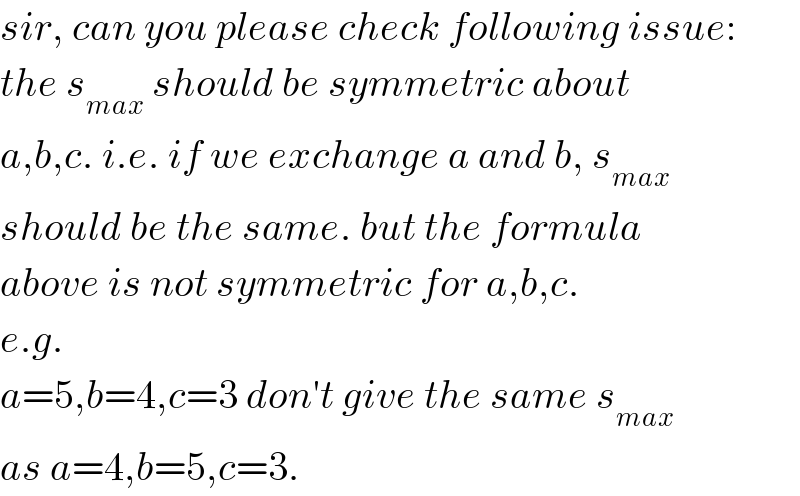
sir, can you please check following issue: the s_(max) should be symmetric about a,b,c. i.e. if we exchange a and b, s_(max) should be the same. but the formula above is not symmetric for a,b,c. e.g. a=5,b=4,c=3 don′t give the same s_(max) as a=4,b=5,c=3.
$${sir},\:{can}\:{you}\:{please}\:{check}\:{following}\:{issue}: \\ $$$${the}\:{s}_{{max}} \:{should}\:{be}\:{symmetric}\:{about} \\ $$$${a},{b},{c}.\:{i}.{e}.\:{if}\:{we}\:{exchange}\:{a}\:{and}\:{b},\:{s}_{{max}} \\ $$$${should}\:{be}\:{the}\:{same}.\:{but}\:{the}\:{formula} \\ $$$${above}\:{is}\:{not}\:{symmetric}\:{for}\:{a},{b},{c}. \\ $$$${e}.{g}. \\ $$$${a}=\mathrm{5},{b}=\mathrm{4},{c}=\mathrm{3}\:{don}'{t}\:{give}\:{the}\:{same}\:{s}_{{max}} \\ $$$${as}\:{a}=\mathrm{4},{b}=\mathrm{5},{c}=\mathrm{3}. \\ $$
Commented by ajfour last updated on 15/Mar/19
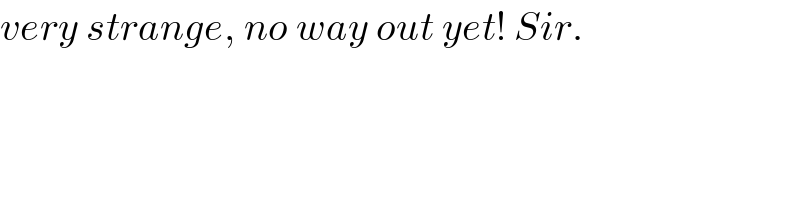
very strange, no way out yet! Sir.
$${very}\:{strange},\:{no}\:{way}\:{out}\:{yet}!\:{Sir}. \\ $$
Answered by mr W last updated on 13/Mar/19
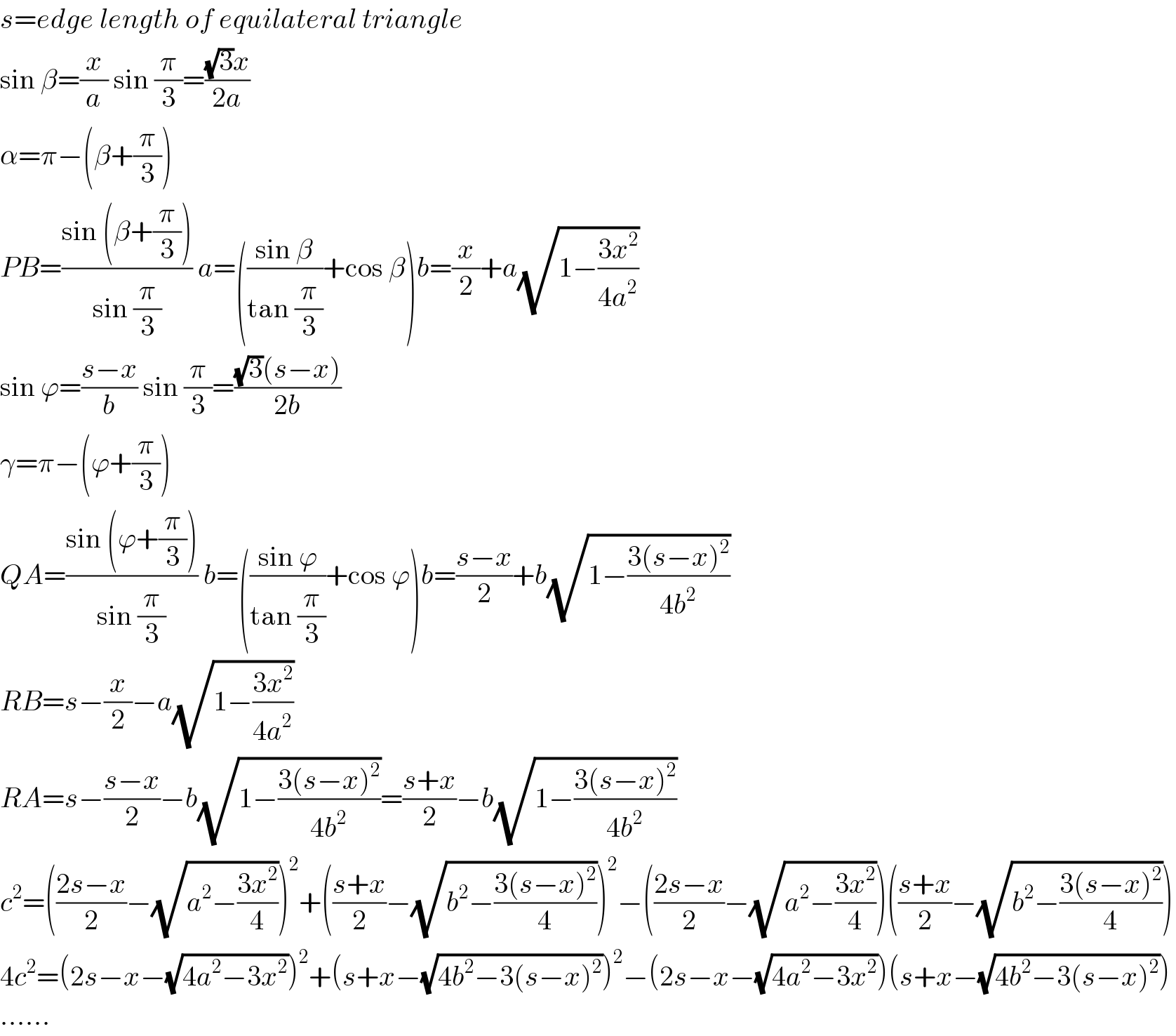
s=edge length of equilateral triangle sin β=(x/a) sin (π/3)=(((√3)x)/(2a)) α=π−(β+(π/3)) PB=((sin (β+(π/3)))/(sin (π/3))) a=(((sin β)/(tan (π/3)))+cos β)b=(x/2)+a(√(1−((3x^2 )/(4a^2 )))) sin ϕ=((s−x)/b) sin (π/3)=(((√3)(s−x))/(2b)) γ=π−(ϕ+(π/3)) QA=((sin (ϕ+(π/3)))/(sin (π/3))) b=(((sin ϕ)/(tan (π/3)))+cos ϕ)b=((s−x)/2)+b(√(1−((3(s−x)^2 )/(4b^2 )))) RB=s−(x/2)−a(√(1−((3x^2 )/(4a^2 )))) RA=s−((s−x)/2)−b(√(1−((3(s−x)^2 )/(4b^2 ))))=((s+x)/2)−b(√(1−((3(s−x)^2 )/(4b^2 )))) c^2 =(((2s−x)/2)−(√(a^2 −((3x^2 )/4))))^2 +(((s+x)/2)−(√(b^2 −((3(s−x)^2 )/4))))^2 −(((2s−x)/2)−(√(a^2 −((3x^2 )/4))))(((s+x)/2)−(√(b^2 −((3(s−x)^2 )/4)))) 4c^2 =(2s−x−(√(4a^2 −3x^2 )))^2 +(s+x−(√(4b^2 −3(s−x)^2 )))^2 −(2s−x−(√(4a^2 −3x^2 )))(s+x−(√(4b^2 −3(s−x)^2 ))) ......
$${s}={edge}\:{length}\:{of}\:{equilateral}\:{triangle} \\ $$$$\mathrm{sin}\:\beta=\frac{{x}}{{a}}\:\mathrm{sin}\:\frac{\pi}{\mathrm{3}}=\frac{\sqrt{\mathrm{3}}{x}}{\mathrm{2}{a}} \\ $$$$\alpha=\pi−\left(\beta+\frac{\pi}{\mathrm{3}}\right) \\ $$$${PB}=\frac{\mathrm{sin}\:\left(\beta+\frac{\pi}{\mathrm{3}}\right)}{\mathrm{sin}\:\frac{\pi}{\mathrm{3}}}\:{a}=\left(\frac{\mathrm{sin}\:\beta}{\mathrm{tan}\:\frac{\pi}{\mathrm{3}}}+\mathrm{cos}\:\beta\right){b}=\frac{{x}}{\mathrm{2}}+{a}\sqrt{\mathrm{1}−\frac{\mathrm{3}{x}^{\mathrm{2}} }{\mathrm{4}{a}^{\mathrm{2}} }} \\ $$$$\mathrm{sin}\:\varphi=\frac{{s}−{x}}{{b}}\:\mathrm{sin}\:\frac{\pi}{\mathrm{3}}=\frac{\sqrt{\mathrm{3}}\left({s}−{x}\right)}{\mathrm{2}{b}} \\ $$$$\gamma=\pi−\left(\varphi+\frac{\pi}{\mathrm{3}}\right) \\ $$$${QA}=\frac{\mathrm{sin}\:\left(\varphi+\frac{\pi}{\mathrm{3}}\right)}{\mathrm{sin}\:\frac{\pi}{\mathrm{3}}}\:{b}=\left(\frac{\mathrm{sin}\:\varphi}{\mathrm{tan}\:\frac{\pi}{\mathrm{3}}}+\mathrm{cos}\:\varphi\right){b}=\frac{{s}−{x}}{\mathrm{2}}+{b}\sqrt{\mathrm{1}−\frac{\mathrm{3}\left({s}−{x}\right)^{\mathrm{2}} }{\mathrm{4}{b}^{\mathrm{2}} }} \\ $$$${RB}={s}−\frac{{x}}{\mathrm{2}}−{a}\sqrt{\mathrm{1}−\frac{\mathrm{3}{x}^{\mathrm{2}} }{\mathrm{4}{a}^{\mathrm{2}} }} \\ $$$${RA}={s}−\frac{{s}−{x}}{\mathrm{2}}−{b}\sqrt{\mathrm{1}−\frac{\mathrm{3}\left({s}−{x}\right)^{\mathrm{2}} }{\mathrm{4}{b}^{\mathrm{2}} }}=\frac{{s}+{x}}{\mathrm{2}}−{b}\sqrt{\mathrm{1}−\frac{\mathrm{3}\left({s}−{x}\right)^{\mathrm{2}} }{\mathrm{4}{b}^{\mathrm{2}} }} \\ $$$${c}^{\mathrm{2}} =\left(\frac{\mathrm{2}{s}−{x}}{\mathrm{2}}−\sqrt{{a}^{\mathrm{2}} −\frac{\mathrm{3}{x}^{\mathrm{2}} }{\mathrm{4}}}\right)^{\mathrm{2}} +\left(\frac{{s}+{x}}{\mathrm{2}}−\sqrt{{b}^{\mathrm{2}} −\frac{\mathrm{3}\left({s}−{x}\right)^{\mathrm{2}} }{\mathrm{4}}}\right)^{\mathrm{2}} −\left(\frac{\mathrm{2}{s}−{x}}{\mathrm{2}}−\sqrt{{a}^{\mathrm{2}} −\frac{\mathrm{3}{x}^{\mathrm{2}} }{\mathrm{4}}}\right)\left(\frac{{s}+{x}}{\mathrm{2}}−\sqrt{{b}^{\mathrm{2}} −\frac{\mathrm{3}\left({s}−{x}\right)^{\mathrm{2}} }{\mathrm{4}}}\right) \\ $$$$\mathrm{4}{c}^{\mathrm{2}} =\left(\mathrm{2}{s}−{x}−\sqrt{\mathrm{4}{a}^{\mathrm{2}} −\mathrm{3}{x}^{\mathrm{2}} }\right)^{\mathrm{2}} +\left({s}+{x}−\sqrt{\mathrm{4}{b}^{\mathrm{2}} −\mathrm{3}\left({s}−{x}\right)^{\mathrm{2}} }\right)^{\mathrm{2}} −\left(\mathrm{2}{s}−{x}−\sqrt{\mathrm{4}{a}^{\mathrm{2}} −\mathrm{3}{x}^{\mathrm{2}} }\right)\left({s}+{x}−\sqrt{\mathrm{4}{b}^{\mathrm{2}} −\mathrm{3}\left({s}−{x}\right)^{\mathrm{2}} }\right) \\ $$$$…… \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *