Question Number 56971 by tanmay.chaudhury50@gmail.com last updated on 27/Mar/19

Commented by tanmay.chaudhury50@gmail.com last updated on 27/Mar/19

$${source}\:..{book} \\ $$
Answered by mr W last updated on 27/Mar/19
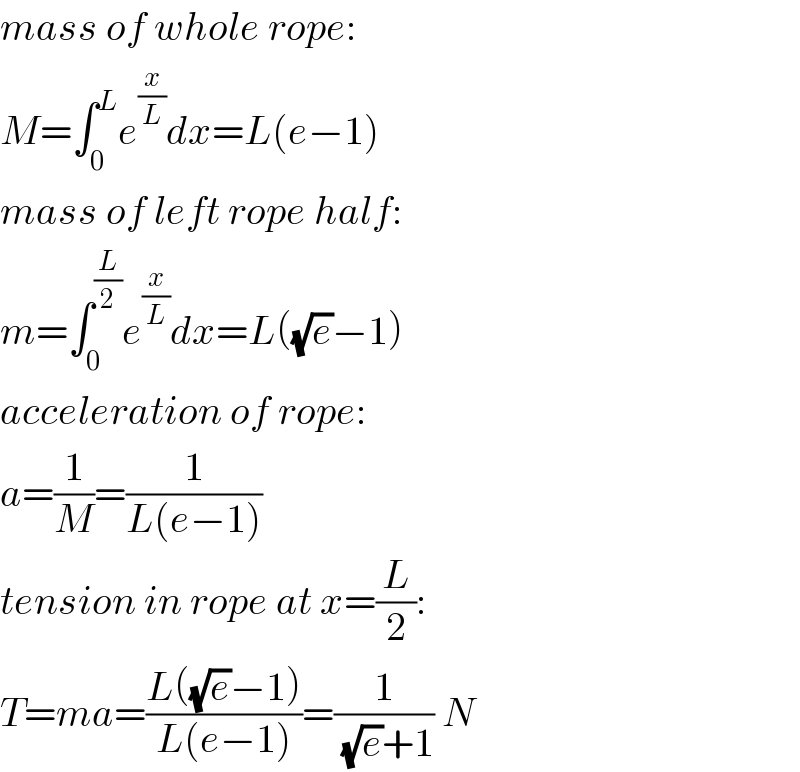
$${mass}\:{of}\:{whole}\:{rope}: \\ $$$${M}=\int_{\mathrm{0}} ^{{L}} {e}^{\frac{{x}}{{L}}} {dx}={L}\left({e}−\mathrm{1}\right) \\ $$$${mass}\:{of}\:{left}\:{rope}\:{half}: \\ $$$${m}=\int_{\mathrm{0}} ^{\frac{{L}}{\mathrm{2}}} {e}^{\frac{{x}}{{L}}} {dx}={L}\left(\sqrt{{e}}−\mathrm{1}\right) \\ $$$${acceleration}\:{of}\:{rope}: \\ $$$${a}=\frac{\mathrm{1}}{{M}}=\frac{\mathrm{1}}{{L}\left({e}−\mathrm{1}\right)} \\ $$$${tension}\:{in}\:{rope}\:{at}\:{x}=\frac{{L}}{\mathrm{2}}: \\ $$$${T}={ma}=\frac{{L}\left(\sqrt{{e}}−\mathrm{1}\right)}{{L}\left({e}−\mathrm{1}\right)}=\frac{\mathrm{1}}{\:\sqrt{{e}}+\mathrm{1}}\:{N} \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 27/Mar/19
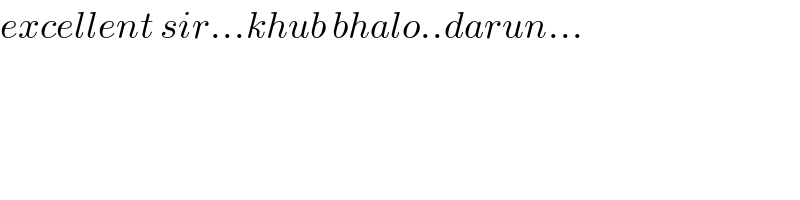
$${excellent}\:{sir}…{khub}\:{bhalo}..{darun}… \\ $$
