Question-60413

Question Number 60413 by tanmay last updated on 20/May/19

Commented by Meritguide1234 last updated on 21/May/19

why do you post same question and solition from goiit page by Sourav De

Commented by MJS last updated on 21/May/19

why not ?

Commented by tanmay last updated on 21/May/19

w e a r e a l l s t u d e n t s \dots w e a r e n o t T o m a s h a r d y

o r R a m a n u j a m \dots w e l e a r n f r o m e a c h o t h e r

w h a t d o [y o u w a n t t o s a y . . i j u s t s h a r e d t h e s o l u t i o n

t o o t h e r s \dots i a m n o t i n t h e s i d e o f o t h e r s

w h o t h i n k t h a t t h e y k n o w e v e r y t h i n g \dots i a m s t i l l

a s t u d e n t \dots i h a v e m i l e s t o g o . .

Commented by maxmathsup by imad last updated on 26/May/19

t h i s p l a t f o r m i s o p e n t o a l l k i n d o f e x e r c i c e s w e d o n o t d i s t i n g u i c h

t h a t a n d t h a t t h a n k s t o s i r T i n k u t a r a \dots .

Answered by tanmay last updated on 21/May/19

\int_{a}^{b} f (x) d x = \int_{a}^{b} f (a + b - x) d x

I = \int_{0}^{1} \frac{{t a n}^{- 1} (\frac{x}{x + 1})}{{t a n}^{- 1} (\frac{1 + 2 x - 2 x^{2}}{2})} d x

= \int_{0}^{1} \frac{{t a n}^{- 1} (\frac{1 - x}{1 - x + 1})}{{t a n}^{- 1} (\frac{1 + 2 (1 - x) - 2 {(1 - x)}^{2}}{2})} d x

= \int_{0}^{1} \frac{{t a n}^{- 1} (\frac{1 - x}{2 - x})}{{t a n}^{- 1} (\frac{1 + 2 - 2 x - 2 + 4 x - 2 x^{2}}{2})} d x

= \int_{0}^{1} \frac{{t a n}^{- 1} (\frac{1 - x}{1 + x})}{{t a n}^{- 1} (\frac{1 + 2 x - 2 x^{2}}{2})} d x

2 I = \int_{0}^{1} \frac{{t a n}^{- 1} (\frac{x}{x + 1}) + {t a n}^{- 1} (\frac{1 - x}{2 - x})}{{t a n}^{- 1} (\frac{1 + 2 x - 2 x^{2}}{2})} d x

n o w N_{r}

{t a n}^{- 1} (\frac{x}{x + 1}) + {t a n}^{- 1} (\frac{1 - x}{2 - x})

= {t a n}^{- 1} (\frac{\frac{x}{x + 1} + \frac{1 - x}{2 - x}}{1 - \frac{x (1 - x)}{(x + 1) (2 - x)}})

= {t a n}^{- 1} (\frac{2 x - x^{2} + 1 - x^{2}}{2 x - x^{2} + 2 - x - x + x^{2}})

= {t a n}^{- 1} (\frac{1 + 2 x - 2 x^{2}}{2}) = D_{r}

s o \frac{N_{r}}{D_{r}} = 1

2 I = \int_{0}^{1} 1 \times d x

2 I = 1

I = \frac{1}{2}

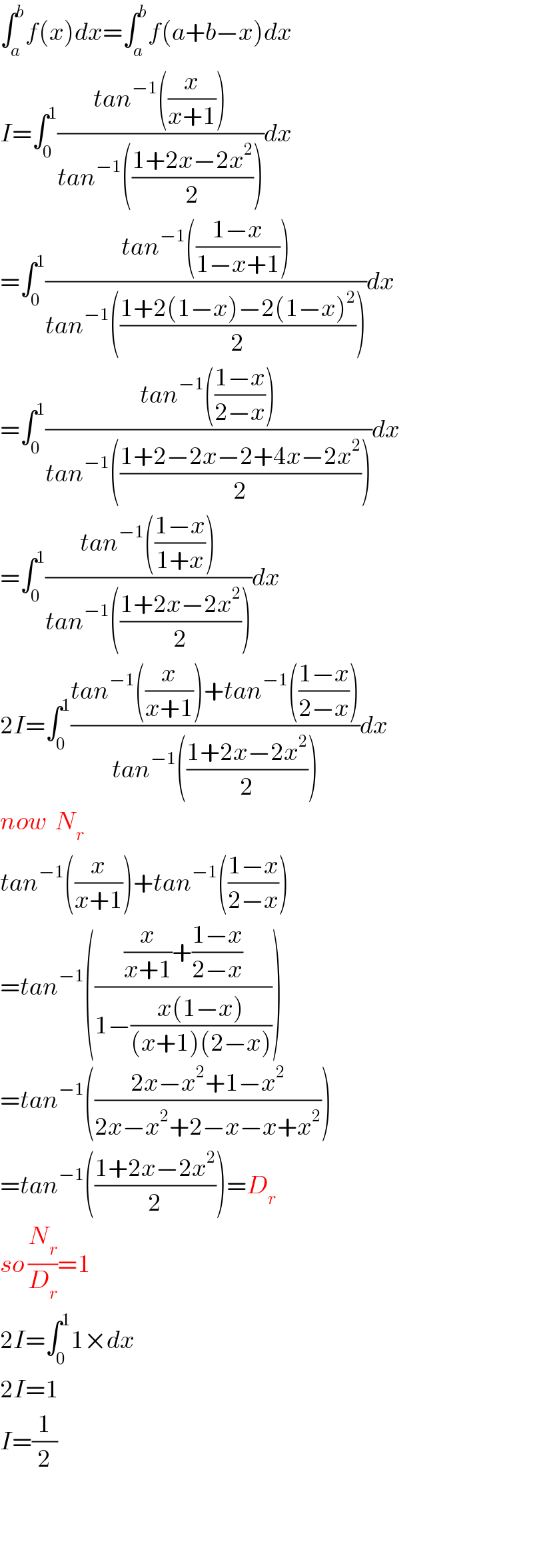
Commented by Meritguide1234 last updated on 21/May/19