Question Number 60788 by ajfour last updated on 25/May/19
Commented by ajfour last updated on 25/May/19

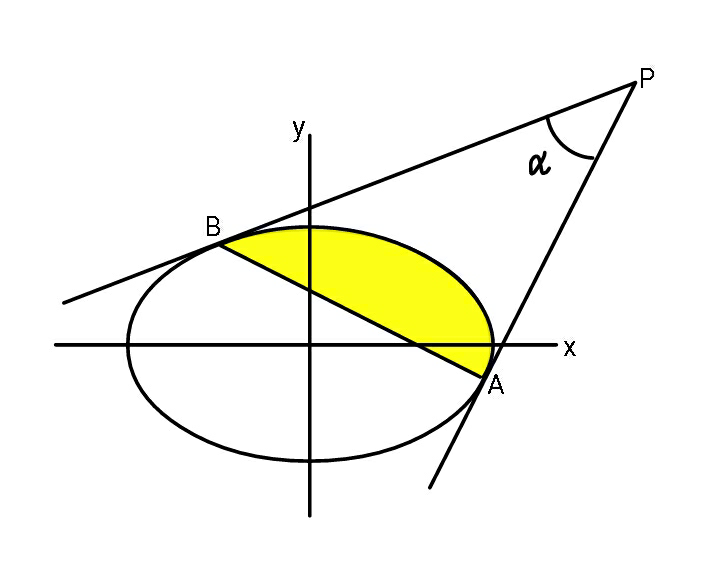
$${In}\:{terms}\:{of}\:\alpha\:{and}\:{ellipse}\:{parameters} \\ $$$${a}\:{and}\:{b},\:{find}\:{maximum}\:{colored}\:{area}. \\ $$
Answered by mr W last updated on 27/May/19
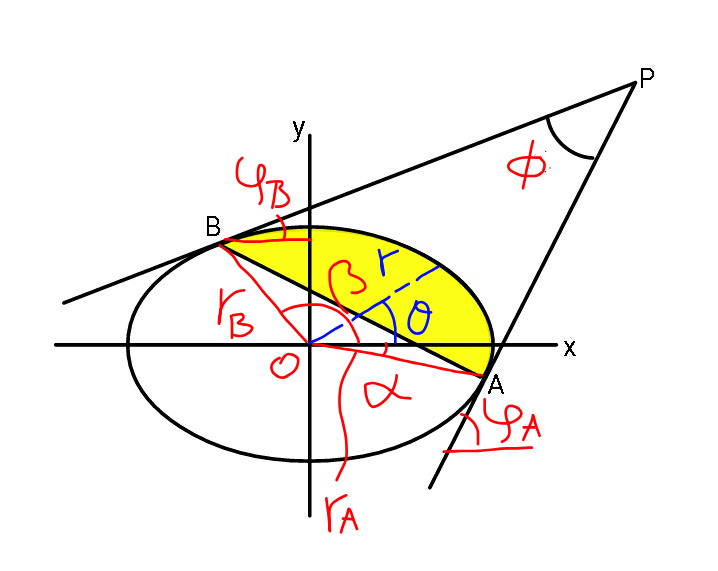
![α in diagram will be replaced by ∅. let μ=(a/b)≥1 (x^2 /a^2 )+(y^2 /b^2 )=1 ((r^2 cos^2 θ)/a^2 )+((r^2 sin^2 θ)/b^2 )=1 ⇒r^2 =((a^2 b^2 )/(a^2 sin^2 θ+b^2 cos^2 θ)) ((2x)/a^2 )+((2yy′)/b^2 )=0 ((r cos θ)/a^2 )+((r sin θy′)/b^2 )=0 ⇒y′=tan ϕ=−(a^2 /(b^2 tan θ))=−(μ^2 /(tan θ)) at A: r_A , θ_A =α, ϕ_A at B: r_B , θ_A =β, ϕ_B tan ϕ_A =−(a^2 /(b^2 tan α)) tan ϕ_B =−(a^2 /(b^2 tan β)) ∅=ϕ_A −ϕ_B tan ∅=tan (ϕ_A −ϕ_B )=((−(a^2 /(b^2 tan α))+(a^2 /(b^2 tan β)))/(1+(a^4 /(b^4 tan α tan β)))) ⇒tan ∅=((a/b))^2 ((tan α−tan β)/(((a/b))^4 +tan α tan β)) ⇒tan ∅=μ^2 ((tan α−tan β)/(μ^4 +tan α tan β)) A=shaded area A=∫_θ_A ^θ_B ((r^2 dθ)/2)−((r_A r_B sin (θ_B −θ_A ))/2) A=(1/2)∫_α ^β ((a^2 b^2 dθ)/(a^2 sin^2 θ+b^2 cos^2 θ))−((sin (β−α))/2)(√(((a^2 b^2 )/(a^2 sin^2 α+b^2 cos^2 α))×((a^2 b^2 )/(a^2 sin^2 β+b^2 cos^2 β)))) A=((a^2 b^2 )/2)∫_α ^β (dθ/(a^2 sin^2 θ+b^2 cos^2 θ))−((a^2 b^2 sin (β−α))/2)(√(1/((a^2 sin^2 α+b^2 cos^2 α)(a^2 sin^2 β+b^2 cos^2 β)))) A=((ab)/2)[tan^(−1) (((a tan β)/b))−tan^(−1) (((a tan α)/b))]−((a^2 b^2 sin (β−α))/(2b^2 ))(√(1/((μ^2 sin^2 α+cos^2 α)(μ^2 sin^2 β+cos^2 β)))) A=((ab)/2)[tan^(−1) (μ tan β)−tan^(−1) (μ tan α)]−((μ sin (β−α))/2)(√(1/((μ^2 sin^2 α+cos^2 α)(μ^2 sin^2 β+cos^2 β)))) A=((ab)/2){[tan^(−1) (μ tan β)−tan^(−1) (μ tan α)]−((μ sin (β−α))/( (√((μ^2 sin^2 α+cos^2 α)(μ^2 sin^2 β+cos^2 β)))))} =((ab)/2)×f ⇒f=tan^(−1) (μ tan β)−tan^(−1) (μ tan α)−((μ sin (β−α))/( (√((μ^2 sin^2 α+cos^2 α)(μ^2 sin^2 β+cos^2 β))))) ⇒f=tan^(−1) [((μ(tan β−tan α) )/(1+μ^2 tan β tan α))]−((μ(tan β−tan α))/( (√((1+μ^2 tan^2 α)(1+μ^2 tan^2 β))))) let p=μ tan α, q=μ tan β ⇒f=tan^(−1) (((q−p)/(1+qp)))−((q−p)/( (√((p^2 +1)(q^2 +1))))) ...(i) tan ∅=μ^3 ×((μ tan α−μ tan β)/(μ^6 +μ^2 tan α tan β)) ((tan ∅)/μ^3 )=((p−q)/(μ^6 +pq )) tan ∅ μ^6 +tan ∅ pq=μ^3 p−μ^3 q ⇒q=((μ^3 (p−μ^3 tan ∅))/(μ^3 +p tan ∅)) ...(ii)](https://www.tinkutara.com/question/Q60843.png)
$$\alpha\:{in}\:{diagram}\:{will}\:{be}\:{replaced}\:{by}\:\varnothing. \\ $$$${let}\:\mu=\frac{{a}}{{b}}\geqslant\mathrm{1} \\ $$$$\frac{{x}^{\mathrm{2}} }{{a}^{\mathrm{2}} }+\frac{{y}^{\mathrm{2}} }{{b}^{\mathrm{2}} }=\mathrm{1} \\ $$$$\frac{{r}^{\mathrm{2}} \mathrm{cos}^{\mathrm{2}} \:\theta}{{a}^{\mathrm{2}} }+\frac{{r}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\theta}{{b}^{\mathrm{2}} }=\mathrm{1} \\ $$$$\Rightarrow{r}^{\mathrm{2}} =\frac{{a}^{\mathrm{2}} {b}^{\mathrm{2}} }{{a}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\theta+{b}^{\mathrm{2}} \mathrm{cos}^{\mathrm{2}} \:\theta} \\ $$$$\frac{\mathrm{2}{x}}{{a}^{\mathrm{2}} }+\frac{\mathrm{2}{yy}'}{{b}^{\mathrm{2}} }=\mathrm{0} \\ $$$$\frac{{r}\:\mathrm{cos}\:\theta}{{a}^{\mathrm{2}} }+\frac{{r}\:\mathrm{sin}\:\theta{y}'}{{b}^{\mathrm{2}} }=\mathrm{0} \\ $$$$\Rightarrow{y}'=\mathrm{tan}\:\varphi=−\frac{{a}^{\mathrm{2}} }{{b}^{\mathrm{2}} \:\mathrm{tan}\:\theta}=−\frac{\mu^{\mathrm{2}} }{\mathrm{tan}\:\theta} \\ $$$${at}\:{A}:\:{r}_{{A}} ,\:\theta_{{A}} =\alpha,\:\varphi_{{A}} \\ $$$${at}\:{B}:\:{r}_{{B}} ,\:\theta_{{A}} =\beta,\:\varphi_{{B}} \\ $$$$\mathrm{tan}\:\varphi_{{A}} =−\frac{{a}^{\mathrm{2}} }{{b}^{\mathrm{2}} \:\mathrm{tan}\:\alpha} \\ $$$$\mathrm{tan}\:\varphi_{{B}} =−\frac{{a}^{\mathrm{2}} }{{b}^{\mathrm{2}} \:\mathrm{tan}\:\beta} \\ $$$$ \\ $$$$\varnothing=\varphi_{{A}} −\varphi_{{B}} \\ $$$$\mathrm{tan}\:\varnothing=\mathrm{tan}\:\left(\varphi_{{A}} −\varphi_{{B}} \right)=\frac{−\frac{{a}^{\mathrm{2}} }{{b}^{\mathrm{2}} \mathrm{tan}\:\alpha}+\frac{{a}^{\mathrm{2}} }{{b}^{\mathrm{2}} \mathrm{tan}\:\beta}}{\mathrm{1}+\frac{{a}^{\mathrm{4}} }{{b}^{\mathrm{4}} \mathrm{tan}\:\alpha\:\mathrm{tan}\:\beta}} \\ $$$$\Rightarrow\mathrm{tan}\:\varnothing=\left(\frac{{a}}{{b}}\right)^{\mathrm{2}} \frac{\mathrm{tan}\:\alpha−\mathrm{tan}\:\beta}{\left(\frac{{a}}{{b}}\right)^{\mathrm{4}} +\mathrm{tan}\:\alpha\:\mathrm{tan}\:\beta} \\ $$$$\Rightarrow\mathrm{tan}\:\varnothing=\mu^{\mathrm{2}} \frac{\mathrm{tan}\:\alpha−\mathrm{tan}\:\beta}{\mu^{\mathrm{4}} +\mathrm{tan}\:\alpha\:\mathrm{tan}\:\beta} \\ $$$$ \\ $$$${A}={shaded}\:{area} \\ $$$${A}=\int_{\theta_{{A}} } ^{\theta_{{B}} } \frac{{r}^{\mathrm{2}} {d}\theta}{\mathrm{2}}−\frac{{r}_{{A}} {r}_{{B}} \mathrm{sin}\:\left(\theta_{{B}} −\theta_{{A}} \right)}{\mathrm{2}} \\ $$$${A}=\frac{\mathrm{1}}{\mathrm{2}}\int_{\alpha} ^{\beta} \frac{{a}^{\mathrm{2}} {b}^{\mathrm{2}} \:{d}\theta}{{a}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\theta+{b}^{\mathrm{2}} \mathrm{cos}^{\mathrm{2}} \:\theta}−\frac{\mathrm{sin}\:\left(\beta−\alpha\right)}{\mathrm{2}}\sqrt{\frac{{a}^{\mathrm{2}} {b}^{\mathrm{2}} }{{a}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\alpha+{b}^{\mathrm{2}} \mathrm{cos}^{\mathrm{2}} \:\alpha}×\frac{{a}^{\mathrm{2}} {b}^{\mathrm{2}} }{{a}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\beta+{b}^{\mathrm{2}} \mathrm{cos}^{\mathrm{2}} \:\beta}} \\ $$$${A}=\frac{{a}^{\mathrm{2}} {b}^{\mathrm{2}} }{\mathrm{2}}\int_{\alpha} ^{\beta} \frac{{d}\theta}{{a}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\theta+{b}^{\mathrm{2}} \mathrm{cos}^{\mathrm{2}} \:\theta}−\frac{{a}^{\mathrm{2}} {b}^{\mathrm{2}} \mathrm{sin}\:\left(\beta−\alpha\right)}{\mathrm{2}}\sqrt{\frac{\mathrm{1}}{\left({a}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\alpha+{b}^{\mathrm{2}} \mathrm{cos}^{\mathrm{2}} \:\alpha\right)\left({a}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\beta+{b}^{\mathrm{2}} \mathrm{cos}^{\mathrm{2}} \:\beta\right)}} \\ $$$${A}=\frac{{ab}}{\mathrm{2}}\left[\mathrm{tan}^{−\mathrm{1}} \left(\frac{{a}\:\mathrm{tan}\:\beta}{{b}}\right)−\mathrm{tan}^{−\mathrm{1}} \left(\frac{{a}\:\mathrm{tan}\:\alpha}{{b}}\right)\right]−\frac{{a}^{\mathrm{2}} {b}^{\mathrm{2}} \mathrm{sin}\:\left(\beta−\alpha\right)}{\mathrm{2}{b}^{\mathrm{2}} }\sqrt{\frac{\mathrm{1}}{\left(\mu^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\alpha+\mathrm{cos}^{\mathrm{2}} \:\alpha\right)\left(\mu^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\beta+\mathrm{cos}^{\mathrm{2}} \:\beta\right)}} \\ $$$${A}=\frac{{ab}}{\mathrm{2}}\left[\mathrm{tan}^{−\mathrm{1}} \left(\mu\:\mathrm{tan}\:\beta\right)−\mathrm{tan}^{−\mathrm{1}} \left(\mu\:\mathrm{tan}\:\alpha\right)\right]−\frac{\mu\:\mathrm{sin}\:\left(\beta−\alpha\right)}{\mathrm{2}}\sqrt{\frac{\mathrm{1}}{\left(\mu^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\alpha+\mathrm{cos}^{\mathrm{2}} \:\alpha\right)\left(\mu^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\beta+\mathrm{cos}^{\mathrm{2}} \:\beta\right)}} \\ $$$${A}=\frac{{ab}}{\mathrm{2}}\left\{\left[\mathrm{tan}^{−\mathrm{1}} \left(\mu\:\mathrm{tan}\:\beta\right)−\mathrm{tan}^{−\mathrm{1}} \left(\mu\:\mathrm{tan}\:\alpha\right)\right]−\frac{\mu\:\mathrm{sin}\:\left(\beta−\alpha\right)}{\:\sqrt{\left(\mu^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\alpha+\mathrm{cos}^{\mathrm{2}} \:\alpha\right)\left(\mu^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\beta+\mathrm{cos}^{\mathrm{2}} \:\beta\right)}}\right\} \\ $$$$=\frac{{ab}}{\mathrm{2}}×{f} \\ $$$$\Rightarrow{f}=\mathrm{tan}^{−\mathrm{1}} \left(\mu\:\mathrm{tan}\:\beta\right)−\mathrm{tan}^{−\mathrm{1}} \left(\mu\:\mathrm{tan}\:\alpha\right)−\frac{\mu\:\mathrm{sin}\:\left(\beta−\alpha\right)}{\:\sqrt{\left(\mu^{\mathrm{2}} \:\mathrm{sin}^{\mathrm{2}} \:\alpha+\mathrm{cos}^{\mathrm{2}} \:\alpha\right)\left(\mu^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\beta+\mathrm{cos}^{\mathrm{2}} \:\beta\right)}} \\ $$$$\Rightarrow{f}=\mathrm{tan}^{−\mathrm{1}} \left[\frac{\mu\left(\mathrm{tan}\:\beta−\mathrm{tan}\:\alpha\right)\:}{\mathrm{1}+\mu^{\mathrm{2}} \:\mathrm{tan}\:\beta\:\mathrm{tan}\:\alpha}\right]−\frac{\mu\left(\mathrm{tan}\:\beta−\mathrm{tan}\:\alpha\right)}{\:\sqrt{\left(\mathrm{1}+\mu^{\mathrm{2}} \:\mathrm{tan}^{\mathrm{2}} \:\alpha\right)\left(\mathrm{1}+\mu^{\mathrm{2}} \mathrm{tan}^{\mathrm{2}} \:\beta\right)}} \\ $$$${let}\:{p}=\mu\:\mathrm{tan}\:\alpha,\:{q}=\mu\:\mathrm{tan}\:\beta \\ $$$$\Rightarrow{f}=\mathrm{tan}^{−\mathrm{1}} \left(\frac{{q}−{p}}{\mathrm{1}+{qp}}\right)−\frac{{q}−{p}}{\:\sqrt{\left({p}^{\mathrm{2}} \:+\mathrm{1}\right)\left({q}^{\mathrm{2}} +\mathrm{1}\right)}}\:\:\:…\left({i}\right) \\ $$$$\mathrm{tan}\:\varnothing=\mu^{\mathrm{3}} ×\frac{\mu\:\mathrm{tan}\:\alpha−\mu\:\mathrm{tan}\:\beta}{\mu^{\mathrm{6}} +\mu^{\mathrm{2}} \:\mathrm{tan}\:\alpha\:\mathrm{tan}\:\beta} \\ $$$$\frac{\mathrm{tan}\:\varnothing}{\mu^{\mathrm{3}} }=\frac{{p}−{q}}{\mu^{\mathrm{6}} +{pq}\:} \\ $$$$\mathrm{tan}\:\varnothing\:\mu^{\mathrm{6}} +\mathrm{tan}\:\varnothing\:{pq}=\mu^{\mathrm{3}} {p}−\mu^{\mathrm{3}} {q} \\ $$$$\Rightarrow{q}=\frac{\mu^{\mathrm{3}} \left({p}−\mu^{\mathrm{3}} \mathrm{tan}\:\varnothing\right)}{\mu^{\mathrm{3}} +{p}\:\mathrm{tan}\:\varnothing}\:\:\:…\left({ii}\right) \\ $$
Commented by ajfour last updated on 27/May/19
$${Thanks}\:{so}\:{much}\:{Sir},\:{let}\:{me} \\ $$$${some}\:{time}\:{to}\:{follow}\:{it}\:{determinedly}! \\ $$
Commented by mr W last updated on 27/May/19
Answered by ajfour last updated on 27/May/19
![r=((ab)/( (√(a^2 sin^2 θ+b^2 cos^2 θ)))) A=∫_δ ^( δ+ξ) ((r^2 dθ)/2)−(1/2)r_A r_B sin ξ ((xcos δ)/a)+((ysin δ)/b)=1 ⇒ m_A =−(b/(atan δ)) m_B =−(b/(atan (δ+ξ))) tan α=((−(b/a)[(1/(tan δ))−(1/(tan (δ+ξ)))])/(1+(b^2 /(a^2 tan δtan (δ+ξ))))) ⇒ a^2 tan αtan δtan (δ+ξ)+b^2 tan α = ab[tan (δ+ξ)−tan δ] ⇒ tan (δ+ξ)=(b/a)(((btan α+atan δ)/(b−atan αtan δ))) ξ(δ)=tan^(−1) {(b/a)(((btan α+atan δ)/(b−atan αtan δ)))}−δ A=∫_δ ^( δ+ξ(δ)) ((r^2 dθ)/2)−(1/2)r_δ r_(δ+ξ(δ)) sin ξ(δ) (dA/dδ)=0 should yield a suitable 𝛅.](https://www.tinkutara.com/question/Q60929.png)
$${r}=\frac{{ab}}{\:\sqrt{{a}^{\mathrm{2}} \mathrm{sin}\:^{\mathrm{2}} \theta+{b}^{\mathrm{2}} \mathrm{cos}\:^{\mathrm{2}} \theta}} \\ $$$${A}=\int_{\delta} ^{\:\delta+\xi} \:\frac{{r}^{\mathrm{2}} {d}\theta}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}{r}_{{A}} {r}_{{B}} \mathrm{sin}\:\xi \\ $$$$\frac{{x}\mathrm{cos}\:\delta}{{a}}+\frac{{y}\mathrm{sin}\:\delta}{{b}}=\mathrm{1}\:\:\Rightarrow\:{m}_{{A}} =−\frac{{b}}{{a}\mathrm{tan}\:\delta} \\ $$$${m}_{{B}} =−\frac{{b}}{{a}\mathrm{tan}\:\left(\delta+\xi\right)} \\ $$$$\mathrm{tan}\:\alpha=\frac{−\frac{{b}}{{a}}\left[\frac{\mathrm{1}}{\mathrm{tan}\:\delta}−\frac{\mathrm{1}}{\mathrm{tan}\:\left(\delta+\xi\right)}\right]}{\mathrm{1}+\frac{{b}^{\mathrm{2}} }{{a}^{\mathrm{2}} \mathrm{tan}\:\delta\mathrm{tan}\:\left(\delta+\xi\right)}} \\ $$$$\Rightarrow\:{a}^{\mathrm{2}} \mathrm{tan}\:\alpha\mathrm{tan}\:\delta\mathrm{tan}\:\left(\delta+\xi\right)+{b}^{\mathrm{2}} \mathrm{tan}\:\alpha \\ $$$$\:\:\:\:\:\:=\:{ab}\left[\mathrm{tan}\:\left(\delta+\xi\right)−\mathrm{tan}\:\delta\right] \\ $$$$\Rightarrow\:\mathrm{tan}\:\left(\delta+\xi\right)=\frac{{b}}{{a}}\left(\frac{{b}\mathrm{tan}\:\alpha+{a}\mathrm{tan}\:\delta}{{b}−{a}\mathrm{tan}\:\alpha\mathrm{tan}\:\delta}\right) \\ $$$$\xi\left(\delta\right)=\mathrm{tan}^{−\mathrm{1}} \left\{\frac{{b}}{{a}}\left(\frac{{b}\mathrm{tan}\:\alpha+{a}\mathrm{tan}\:\delta}{{b}−{a}\mathrm{tan}\:\alpha\mathrm{tan}\:\delta}\right)\right\}−\delta \\ $$$${A}=\int_{\delta} ^{\:\delta+\xi\left(\delta\right)} \:\frac{{r}^{\mathrm{2}} {d}\theta}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}{r}_{\delta} {r}_{\delta+\xi\left(\delta\right)} \mathrm{sin}\:\xi\left(\delta\right) \\ $$$$\frac{{dA}}{{d}\delta}=\mathrm{0}\:\:\:{should}\:{yield}\:{a}\:{suitable}\:\boldsymbol{\delta}. \\ $$
