Question-60981

Question Number 60981 by necx1 last updated on 28/May/19

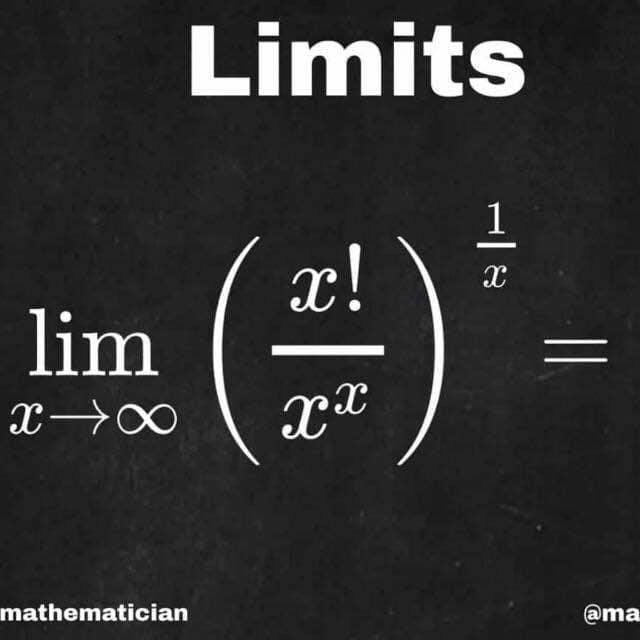
Commented by Prithwish sen last updated on 28/May/19

Let

A = \lim_{x \to \infty} {(\frac{x!}{x^{x}})}^{\frac{1}{x}}

∴ lnA = \lim_{x \to \infty} \frac{1}{x} \ln (\frac{1.2.3 \dots \dots \dots . . x}{x . x . x \dots \dots \dots \dots x})

= \lim_{x \to \infty} \frac{1}{x} (\ln \frac{1}{x} + \ln \frac{2}{x} + \dots \dots . . \ln \frac{x}{x})

= \lim_{x \to \infty} \frac{1}{x} \underset{r = 1}{\sum^{x}} \ln \frac{r}{x}

Missing \left or extra \right

= - 1

∴ A = e^{- 1} = \frac{1}{e}

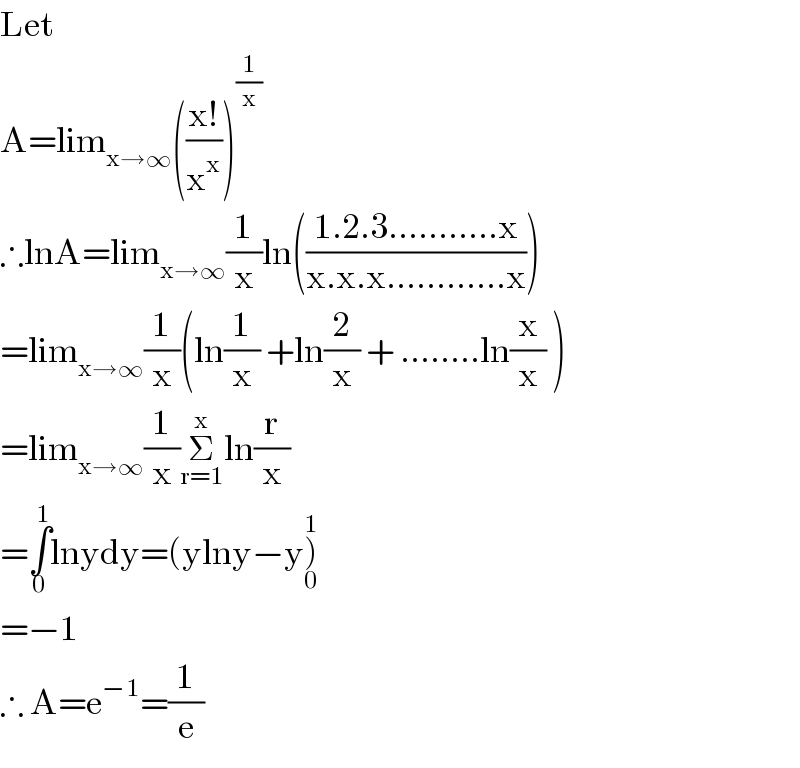
Commented by tanmay last updated on 28/May/19

b a h d a r u n e x c e l l e n t \dots

Commented by Prithwish sen last updated on 28/May/19

thank you sir .

Commented by ajfour last updated on 28/May/19

E x t r e m e l y g r e a t S i r, t h a n k s a l o t .

Commented by Prithwish sen last updated on 28/May/19

welcome .

Commented by Mr X pcx last updated on 28/May/19

s t i r l i n g g o r m u l a e

n! \sim n^{n} e^{- n} \sqrt{2 π n} o r

x! \sim x^{x} e^{- x} \sqrt{2 π x} \Rightarrow \frac{x!}{x^{x}} \sim e^{- x} \sqrt{2 π x} \Rightarrow

{(\frac{x!}{x^{x}})}^{\frac{1}{x}} = {(e^{- x} \sqrt{2 π x})}^{\frac{1}{x}} = A (x)

l n (A (x)) = \frac{1}{x} l n (e^{- x} \sqrt{2 π x})

= - 1 + \frac{1}{2 x} {l n (2 π) + l n (x)}

= - 1 + \frac{l n (2 π)}{2 x} + \frac{l n (x)}{2 x} \to - 1 (x \to + \infty)

\Rightarrow {l i m}_{x \to + \infty} A (x) = e^{- 1} = \frac{1}{e}

s o {l i m}_{x \to + \infty} {(\frac{x!}{x^{x}})}^{\frac{1}{x}} = \frac{1}{e} .

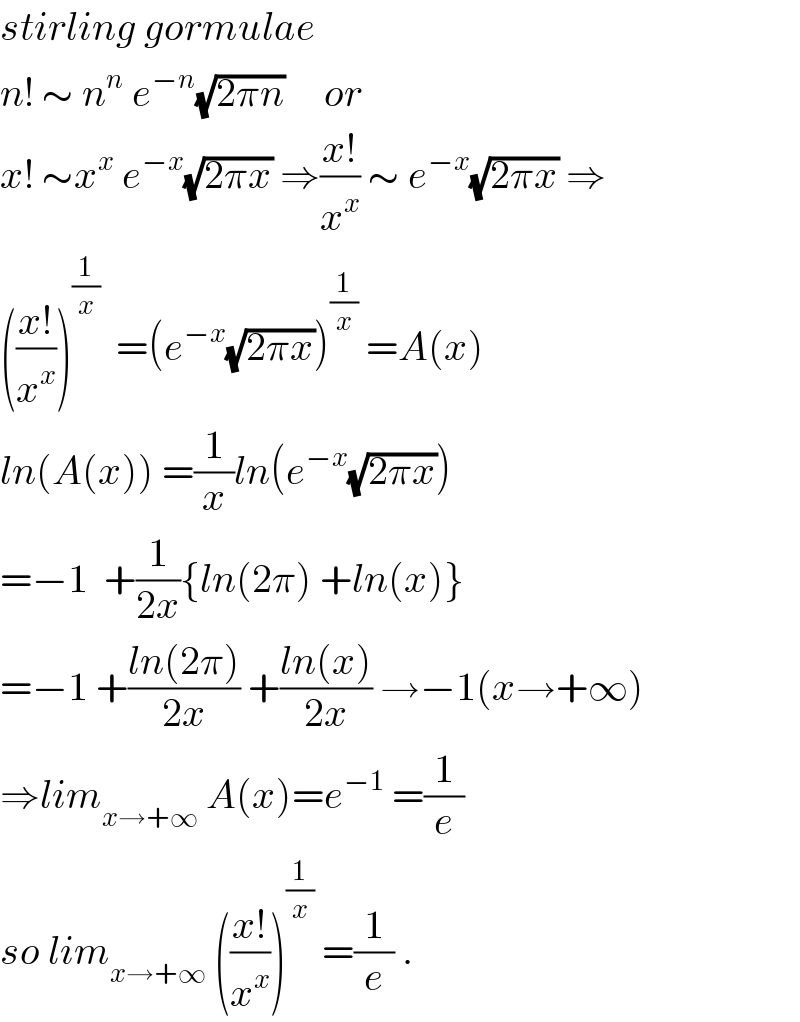
Answered by MJS last updated on 28/May/19

tried with {Stirling}^{'} s formula

x! \approx {(\frac{x}{e})}^{x} \sqrt{2 π x}

{(\frac{x!}{x^{x}})}^{\frac{1}{x}} = \frac{x!^{\frac{1}{x}}}{x} \approx \frac{1}{x} {({(\frac{x}{e})}^{x} \sqrt{2 π x})}^{\frac{1}{x}} = \frac{1}{x} \times \frac{x}{e} {(2 π x)}^{\frac{1}{2 x}} =

= \frac{1}{e} \times 2^{\frac{1}{2 x}} \times π^{\frac{1}{2 x}} \times x^{\frac{1}{2 x}}

\lim_{x \to \infty} \frac{1}{e} \times 2^{\frac{1}{2 x}} \times π^{\frac{1}{2 x}} \times x^{\frac{1}{2 x}} = \frac{1}{e}

this seems right, computing shows

{(\frac{100!}{100^{100}})}^{\frac{1}{100}} \approx . 379927

{(\frac{200!}{200^{200}})}^{\frac{1}{200}} \approx . 374502

{(\frac{300!}{300^{300}})}^{\frac{1}{300}} \approx . 372533

{(\frac{400!}{400^{400}})}^{\frac{1}{400}} \approx . 371498

\frac{1}{e} \approx . 367879

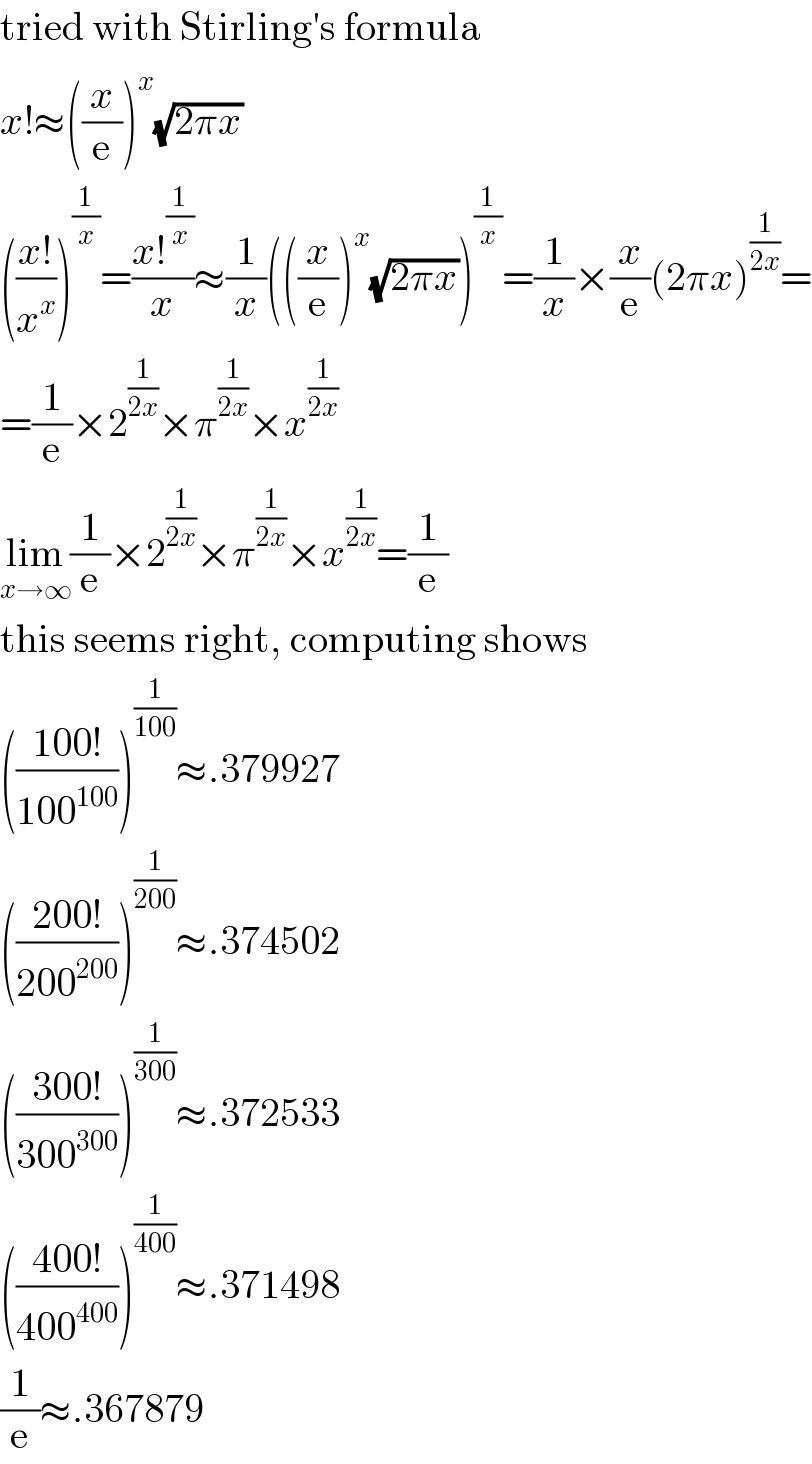
Commented by tanmay last updated on 28/May/19

b a h d a r u n e x c e l l e n t \dots o n e p r o b l e m b u t s o l u t i o n

t h r e e m e t h o d \dots

Answered by tanmay last updated on 28/May/19

\lim_{x \to \infty} \frac{a_{x + 1}}{a_{x}}

= \lim_{x \to \infty} \frac{{\frac{(x + 1)!}{{(x + 1)}^{x + 1}}}}{(\frac{x!}{x^{x}})}

= \lim_{x \to \infty} \frac{(x + 1)!}{x!} \times \frac{1}{\frac{{(x + 1)}^{x + 1}}{x^{x}}}

= \lim_{x \to \infty} (x + 1) \times \frac{1}{(x + 1) {(\frac{x + 1}{x})}^{x}}

= \lim_{x \to \infty} \frac{1}{{(1 + \frac{1}{x})}^{x}}

n o w t = \frac{1}{x}

y = \lim_{t \to 0} \frac{1}{{(1 + t)}^{\frac{1}{t}}}

l n y = \lim_{t \to 0} [\frac{- l n (1 + t)}{t}]

l n y = - 1

y = e^{- 1}

s o \lim_{x \to \infty} {(\frac{x!}{x^{x}})}^{\frac{1}{x}} = e^{- 1} \to i t i s t h e a n s w e r

u s i n g c a u c h y s e c o n d t h e o r e m o n l i m i t

\lim_{n \to \infty} {(a_{n})}^{\frac{1}{n}} = l

i f \lim_{n \to \infty} \frac{a_{n + 1}}{a_{n}} = l

Commented by necx1 last updated on 28/May/19

G o d b l e s s y o u s i r

Facebook Tweet Pin