Question Number 62322 by aliesam last updated on 19/Jun/19
Commented by arcana last updated on 19/Jun/19
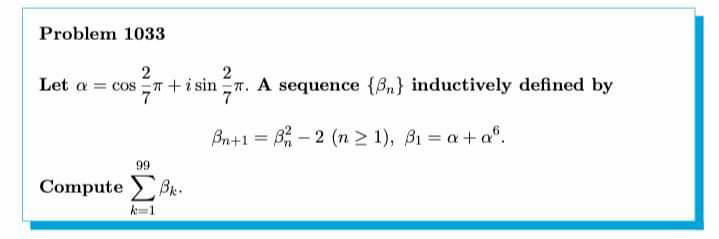
![β_1 =α+α^6 β_2 =(α+α^6 )^2 −2=α^2 +2α^7 +α^(12) −2 β_2 =α^2 +α^5 β_3 =(α^2 +α^5 )^2 −2=α^4 +2α^7 +α^(10) −2 β_3 =α^4 +α^3 β_4 =(α^4 +α^3 )^2 −2=α^8 +2α^7 +α^6 −2 β_4 =α+α^6 =β_1 ⇒Σ_1 ^(99) β_k =33Σ_1 ^3 β_k =33[β_1 +β_2 +β_3 ] =33[α+α^6 +α^2 +α^5 +α^4 +α^3 ]=−33](https://www.tinkutara.com/question/Q62328.png)
$$\beta_{\mathrm{1}} =\alpha+\alpha^{\mathrm{6}} \\ $$$$\beta_{\mathrm{2}} =\left(\alpha+\alpha^{\mathrm{6}} \right)^{\mathrm{2}} −\mathrm{2}=\alpha^{\mathrm{2}} +\mathrm{2}\alpha^{\mathrm{7}} +\alpha^{\mathrm{12}} −\mathrm{2} \\ $$$$\beta_{\mathrm{2}} =\alpha^{\mathrm{2}} +\alpha^{\mathrm{5}} \\ $$$$\beta_{\mathrm{3}} =\left(\alpha^{\mathrm{2}} +\alpha^{\mathrm{5}} \right)^{\mathrm{2}} −\mathrm{2}=\alpha^{\mathrm{4}} +\mathrm{2}\alpha^{\mathrm{7}} +\alpha^{\mathrm{10}} −\mathrm{2} \\ $$$$\beta_{\mathrm{3}} =\alpha^{\mathrm{4}} +\alpha^{\mathrm{3}} \\ $$$$\beta_{\mathrm{4}} =\left(\alpha^{\mathrm{4}} +\alpha^{\mathrm{3}} \right)^{\mathrm{2}} −\mathrm{2}=\alpha^{\mathrm{8}} +\mathrm{2}\alpha^{\mathrm{7}} +\alpha^{\mathrm{6}} −\mathrm{2} \\ $$$$\beta_{\mathrm{4}} =\alpha+\alpha^{\mathrm{6}} =\beta_{\mathrm{1}} \\ $$$$\Rightarrow\underset{\mathrm{1}} {\overset{\mathrm{99}} {\sum}}\beta_{{k}} =\mathrm{33}\underset{\mathrm{1}} {\overset{\mathrm{3}} {\sum}}\beta_{{k}} =\mathrm{33}\left[\beta_{\mathrm{1}} +\beta_{\mathrm{2}} +\beta_{\mathrm{3}} \right] \\ $$$$=\mathrm{33}\left[\alpha+\alpha^{\mathrm{6}} +\alpha^{\mathrm{2}} +\alpha^{\mathrm{5}} +\alpha^{\mathrm{4}} +\alpha^{\mathrm{3}} \right]=−\mathrm{33} \\ $$$$ \\ $$$$ \\ $$
Commented by alphaprime last updated on 19/Jun/19
what's the source of this question ?! please let us know
