Menu Close

Question-62938

Tinku Tara 0 Comments
Pin




Question Number 62938 by ajfour last updated on 27/Jun/19
Commented by ajfour last updated on 27/Jun/19
Question 62861 (revisit)
$${Question}\:\mathrm{62861}\:\left({revisit}\right) \\ $$
Answered by ajfour last updated on 27/Jun/19
let A(x_1 ,x_1 ^2 ) , B(x_2 ,x_2 )^2 , C(x_3 ,x_3 ^2 ) cicumcentre of △ be (h,k) R=(s/( (√3))) (x−h)^2 +(x^2 −k)^2 =s^2 /3 This has four roots x_1 ,x_2 ,x_3 ,x_4 x^4 +(1−2k)x^2 −2hx+(h^2 +k^2 −(s^2 /3))=0 x_1 x_2 x_3 x_4 =h^2 +k^2 −s^2 /3 ....(i) x_1 x_2 x_3 +x_4 (x_1 x_2 +x_2 x_3 +x_3 x_1 )=2h ...(ii) (x_1 x_2 +x_2 x_3 +x_3 x_1 ) +x_4 (x_1 +x_2 +x_3 )=1−2k ..(iii) x_1 +x_2 +x_3 +x_4 =0 ...(iv) But (h,k) also being centroid of △ABC, we have x_1 +x_2 +x_3 =3h ...(v) x_1 ^2 +x_2 ^2 +x_3 ^2 =3k ....(vi) from (iv)&(v) x_1 +x_2 +x_3 = 3h , x_4 =−3h (x_1 +x_2 +x_3 )^2 =x_1 ^2 +x_2 ^2 +x_3 ^2 +2(x_1 x_2 +x_2 x_3 +x_3 x_1 ) ⇒ x_1 x_2 +x_2 x_3 +x_3 x_1 =((9h^2 −3k)/2) Now from (ii) x_1 x_2 x_3 = 2h+3h(((9h^2 −3k)/2)) And using (i) 2h+3h(((9h^2 −3k)/2))+(1/(3h))(h^2 +k^2 −(s^2 /3))=0 .....(I) And using (iii) ((9h^2 −3k)/2) − 9h^2 =1−2k ⇒ k = 9h^2 +2 ......(II) using this in (I) 12h^2 +81h^4 −27h^2 (9h^2 +2)+2h^2 +2(9h^2 +2)^2 −((2s^2 )/3)=0 ⇒ 32h^2 =((2s^2 )/3)−8 ⇒ h^2 =((s^2 −12)/(48)) = (s^2 /(48))−(1/4) k = ((3s^2 )/(16))−(1/4) Now eq. below has four roots, x_4 =−3h = −((√(3(s^2 −12)))/4) x_A is the smaller positive one among x_1 , x_2 , and x_3 . x^4 +(1−2k)x^2 −2hx+(h^2 +k^2 −(s^2 /3))=0 . ___________________________ but as x_1 x_2 x_3 =−(((h^2 +k^2 −s^2 /3)/(3h)))=−q x_1 x_2 +x_2 x_3 +x_3 x_1 =((9h^2 −3k)/2) = p and x_1 +x_2 +x_3 =3h we can solve rather a cubic x^3 −3hx^2 +px+q=0 with h= ((√(s^2 −12))/(4(√3))) , k = ((3s^2 )/(16))−(1/4) , q= ((h^2 +k^2 −s^2 /3)/(3h)) , p=((9h^2 −3k)/2) ■
$${let}\:{A}\left({x}_{\mathrm{1}} ,{x}_{\mathrm{1}} ^{\mathrm{2}} \right)\:,\:{B}\left({x}_{\mathrm{2}} ,{x}_{\mathrm{2}} \right)^{\mathrm{2}} ,\:{C}\left({x}_{\mathrm{3}} ,{x}_{\mathrm{3}} ^{\mathrm{2}} \right) \\ $$$${cicumcentre}\:{of}\:\bigtriangleup\:{be}\:\left({h},{k}\right) \\ $$$${R}=\frac{{s}}{\:\sqrt{\mathrm{3}}} \\ $$$$\left({x}−{h}\right)^{\mathrm{2}} +\left({x}^{\mathrm{2}} −{k}\right)^{\mathrm{2}} ={s}^{\mathrm{2}} /\mathrm{3} \\ $$$${This}\:{has}\:{four}\:{roots}\:{x}_{\mathrm{1}} ,{x}_{\mathrm{2}} ,{x}_{\mathrm{3}} ,{x}_{\mathrm{4}} \\ $$$$\:{x}^{\mathrm{4}} +\left(\mathrm{1}−\mathrm{2}{k}\right){x}^{\mathrm{2}} −\mathrm{2}{hx}+\left({h}^{\mathrm{2}} +{k}^{\mathrm{2}} −\frac{{s}^{\mathrm{2}} }{\mathrm{3}}\right)=\mathrm{0} \\ $$$${x}_{\mathrm{1}} {x}_{\mathrm{2}} {x}_{\mathrm{3}} {x}_{\mathrm{4}} ={h}^{\mathrm{2}} +{k}^{\mathrm{2}} −{s}^{\mathrm{2}} /\mathrm{3}\:\:\:\:….\left({i}\right) \\ $$$${x}_{\mathrm{1}} {x}_{\mathrm{2}} {x}_{\mathrm{3}} +{x}_{\mathrm{4}} \left({x}_{\mathrm{1}} {x}_{\mathrm{2}} +{x}_{\mathrm{2}} {x}_{\mathrm{3}} +{x}_{\mathrm{3}} {x}_{\mathrm{1}} \right)=\mathrm{2}{h}\:\:\:…\left({ii}\right) \\ $$$$\left({x}_{\mathrm{1}} {x}_{\mathrm{2}} +{x}_{\mathrm{2}} {x}_{\mathrm{3}} +{x}_{\mathrm{3}} {x}_{\mathrm{1}} \right)\:\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\:\:+{x}_{\mathrm{4}} \left({x}_{\mathrm{1}} +{x}_{\mathrm{2}} +{x}_{\mathrm{3}} \right)=\mathrm{1}−\mathrm{2}{k}\:\:\:..\left({iii}\right) \\ $$$$\:{x}_{\mathrm{1}} +{x}_{\mathrm{2}} +{x}_{\mathrm{3}} +{x}_{\mathrm{4}} =\mathrm{0}\:\:\:\:\:\:\:\:\:\:…\left({iv}\right) \\ $$$$\:\:\:\:{But}\:\left({h},{k}\right)\:{also}\:{being}\:{centroid} \\ $$$${of}\:\bigtriangleup{ABC},\:{we}\:{have} \\ $$$$\:\:\:\:{x}_{\mathrm{1}} +{x}_{\mathrm{2}} +{x}_{\mathrm{3}} =\mathrm{3}{h}\:\:\:\:\:\:\:…\left({v}\right) \\ $$$$\:\:\:\:{x}_{\mathrm{1}} ^{\mathrm{2}} +{x}_{\mathrm{2}} ^{\mathrm{2}} +{x}_{\mathrm{3}} ^{\mathrm{2}} =\mathrm{3}{k}\:\:\:\:\:\:\:….\left({vi}\right) \\ $$$${from}\:\left({iv}\right)\&\left({v}\right) \\ $$$$\:\:\:{x}_{\mathrm{1}} +{x}_{\mathrm{2}} +{x}_{\mathrm{3}} =\:\mathrm{3}{h}\:\:,\:{x}_{\mathrm{4}} =−\mathrm{3}{h} \\ $$$$\left({x}_{\mathrm{1}} +{x}_{\mathrm{2}} +{x}_{\mathrm{3}} \right)^{\mathrm{2}} ={x}_{\mathrm{1}} ^{\mathrm{2}} +{x}_{\mathrm{2}} ^{\mathrm{2}} +{x}_{\mathrm{3}} ^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+\mathrm{2}\left({x}_{\mathrm{1}} {x}_{\mathrm{2}} +{x}_{\mathrm{2}} {x}_{\mathrm{3}} +{x}_{\mathrm{3}} {x}_{\mathrm{1}} \right) \\ $$$$\Rightarrow\:{x}_{\mathrm{1}} {x}_{\mathrm{2}} +{x}_{\mathrm{2}} {x}_{\mathrm{3}} +{x}_{\mathrm{3}} {x}_{\mathrm{1}} =\frac{\mathrm{9}{h}^{\mathrm{2}} −\mathrm{3}{k}}{\mathrm{2}} \\ $$$${Now}\:{from}\:\left({ii}\right) \\ $$$$\:\:{x}_{\mathrm{1}} {x}_{\mathrm{2}} {x}_{\mathrm{3}} =\:\mathrm{2}{h}+\mathrm{3}{h}\left(\frac{\mathrm{9}{h}^{\mathrm{2}} −\mathrm{3}{k}}{\mathrm{2}}\right) \\ $$$${And}\:{using}\:\left({i}\right) \\ $$$$\:\:\mathrm{2}{h}+\mathrm{3}{h}\left(\frac{\mathrm{9}{h}^{\mathrm{2}} −\mathrm{3}{k}}{\mathrm{2}}\right)+\frac{\mathrm{1}}{\mathrm{3}{h}}\left({h}^{\mathrm{2}} +{k}^{\mathrm{2}} −\frac{{s}^{\mathrm{2}} }{\mathrm{3}}\right)=\mathrm{0} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:…..\left({I}\right) \\ $$$${And}\:{using}\:\left({iii}\right) \\ $$$$\frac{\mathrm{9}{h}^{\mathrm{2}} −\mathrm{3}{k}}{\mathrm{2}}\:−\:\mathrm{9}{h}^{\mathrm{2}} =\mathrm{1}−\mathrm{2}{k} \\ $$$$\Rightarrow\:{k}\:=\:\mathrm{9}{h}^{\mathrm{2}} +\mathrm{2}\:\:\:\:\:\:\:\:……\left({II}\right) \\ $$$${using}\:{this}\:{in}\:\left({I}\right) \\ $$$$\mathrm{12}{h}^{\mathrm{2}} +\mathrm{81}{h}^{\mathrm{4}} −\mathrm{27}{h}^{\mathrm{2}} \left(\mathrm{9}{h}^{\mathrm{2}} +\mathrm{2}\right)+\mathrm{2}{h}^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:+\mathrm{2}\left(\mathrm{9}{h}^{\mathrm{2}} +\mathrm{2}\right)^{\mathrm{2}} −\frac{\mathrm{2}{s}^{\mathrm{2}} }{\mathrm{3}}=\mathrm{0} \\ $$$$\Rightarrow\:\mathrm{32}{h}^{\mathrm{2}} =\frac{\mathrm{2}{s}^{\mathrm{2}} }{\mathrm{3}}−\mathrm{8} \\ $$$$\Rightarrow\:\boldsymbol{{h}}^{\mathrm{2}} =\frac{\boldsymbol{{s}}^{\mathrm{2}} −\mathrm{12}}{\mathrm{48}}\:\:=\:\frac{\boldsymbol{{s}}^{\mathrm{2}} }{\mathrm{48}}−\frac{\mathrm{1}}{\mathrm{4}} \\ $$$$\:\:\:\:\:\:\boldsymbol{{k}}\:=\:\frac{\mathrm{3}\boldsymbol{{s}}^{\mathrm{2}} }{\mathrm{16}}−\frac{\mathrm{1}}{\mathrm{4}} \\ $$$${Now}\:{eq}.\:{below}\:{has}\:{four}\:{roots}, \\ $$$${x}_{\mathrm{4}} =−\mathrm{3}{h}\:=\:−\frac{\sqrt{\mathrm{3}\left({s}^{\mathrm{2}} −\mathrm{12}\right)}}{\mathrm{4}} \\ $$$${x}_{{A}} \:\:{is}\:{the}\:{smaller}\:{positive}\:{one} \\ $$$${among}\:{x}_{\mathrm{1}} ,\:{x}_{\mathrm{2}} ,\:{and}\:{x}_{\mathrm{3}} . \\ $$$$\:{x}^{\mathrm{4}} +\left(\mathrm{1}−\mathrm{2}{k}\right){x}^{\mathrm{2}} −\mathrm{2}{hx}+\left({h}^{\mathrm{2}} +{k}^{\mathrm{2}} −\frac{{s}^{\mathrm{2}} }{\mathrm{3}}\right)=\mathrm{0}\:. \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_ \\ $$$${but}\:{as} \\ $$$$\:{x}_{\mathrm{1}} {x}_{\mathrm{2}} {x}_{\mathrm{3}} =−\left(\frac{{h}^{\mathrm{2}} +{k}^{\mathrm{2}} −{s}^{\mathrm{2}} /\mathrm{3}}{\mathrm{3}{h}}\right)=−{q} \\ $$$${x}_{\mathrm{1}} {x}_{\mathrm{2}} +{x}_{\mathrm{2}} {x}_{\mathrm{3}} +{x}_{\mathrm{3}} {x}_{\mathrm{1}} =\frac{\mathrm{9}{h}^{\mathrm{2}} −\mathrm{3}{k}}{\mathrm{2}}\:=\:{p} \\ $$$${and}\:\:\:{x}_{\mathrm{1}} +{x}_{\mathrm{2}} +{x}_{\mathrm{3}} =\mathrm{3}{h} \\ $$$${we}\:{can}\:{solve}\:{rather}\:{a}\:{cubic} \\ $$$$\:\:\:{x}^{\mathrm{3}} −\mathrm{3}{hx}^{\mathrm{2}} +{px}+{q}=\mathrm{0}\: \\ $$$${with}\:\:\:\:{h}=\:\frac{\sqrt{{s}^{\mathrm{2}} −\mathrm{12}}}{\mathrm{4}\sqrt{\mathrm{3}}}\:,\:{k}\:=\:\frac{\mathrm{3}{s}^{\mathrm{2}} }{\mathrm{16}}−\frac{\mathrm{1}}{\mathrm{4}}\:, \\ $$$$\:{q}=\:\frac{{h}^{\mathrm{2}} +{k}^{\mathrm{2}} −{s}^{\mathrm{2}} /\mathrm{3}}{\mathrm{3}{h}}\:,\:{p}=\frac{\mathrm{9}{h}^{\mathrm{2}} −\mathrm{3}{k}}{\mathrm{2}}\:\blacksquare \\ $$
Commented by mr W last updated on 27/Jun/19
a check with s=2(√3): h=0, k=2 q=0, p=−3 x^3 −3x=0 ⇒x=0 (point A) ⇒x=±(√3) (point B and C) ⇒correct!
$${a}\:{check}\:{with}\:{s}=\mathrm{2}\sqrt{\mathrm{3}}: \\ $$$${h}=\mathrm{0},\:{k}=\mathrm{2} \\ $$$${q}=\mathrm{0},\:{p}=−\mathrm{3} \\ $$$${x}^{\mathrm{3}} −\mathrm{3}{x}=\mathrm{0}\: \\ $$$$\Rightarrow{x}=\mathrm{0}\:\left({point}\:{A}\right) \\ $$$$\Rightarrow{x}=\pm\sqrt{\mathrm{3}}\:\left({point}\:{B}\:{and}\:{C}\right) \\ $$$$\Rightarrow{correct}! \\ $$
Commented by mr W last updated on 27/Jun/19
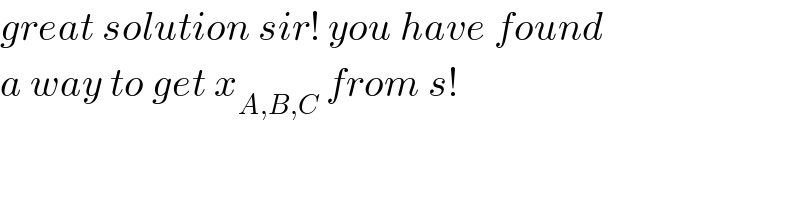
great solution sir! you have found a way to get x_(A,B,C) from s!
$${great}\:{solution}\:{sir}!\:{you}\:{have}\:{found} \\ $$$${a}\:{way}\:{to}\:{get}\:{x}_{{A},{B},{C}} \:{from}\:{s}! \\ $$
Commented by ajfour last updated on 27/Jun/19
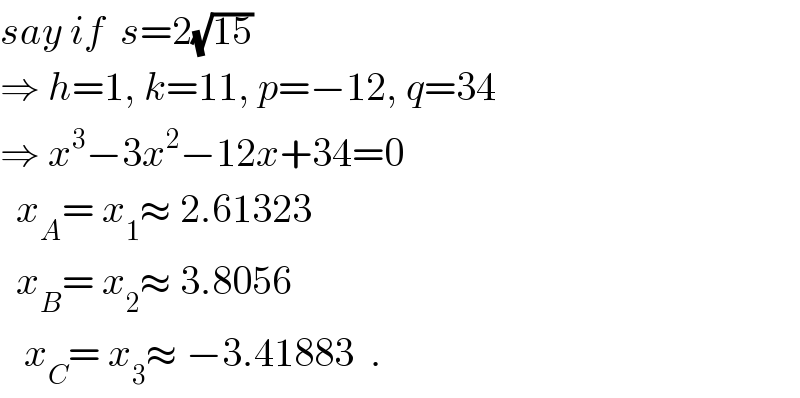
say if s=2(√(15)) ⇒ h=1, k=11, p=−12, q=34 ⇒ x^3 −3x^2 −12x+34=0 x_A = x_1 ≈ 2.61323 x_B = x_2 ≈ 3.8056 x_C = x_3 ≈ −3.41883 .
$${say}\:{if}\:\:{s}=\mathrm{2}\sqrt{\mathrm{15}} \\ $$$$\Rightarrow\:{h}=\mathrm{1},\:{k}=\mathrm{11},\:{p}=−\mathrm{12},\:{q}=\mathrm{34} \\ $$$$\Rightarrow\:{x}^{\mathrm{3}} −\mathrm{3}{x}^{\mathrm{2}} −\mathrm{12}{x}+\mathrm{34}=\mathrm{0} \\ $$$$\:\:{x}_{{A}} =\:{x}_{\mathrm{1}} \approx\:\mathrm{2}.\mathrm{61323} \\ $$$$\:\:{x}_{{B}} =\:{x}_{\mathrm{2}} \approx\:\mathrm{3}.\mathrm{8056} \\ $$$$\:\:\:{x}_{{C}} =\:{x}_{\mathrm{3}} \approx\:−\mathrm{3}.\mathrm{41883}\:\:. \\ $$
Commented by ajfour last updated on 27/Jun/19
Thank you sir, it only needed our focus on center of triangle which is centroid as well as circumcenter.
Commented by mr W last updated on 28/Jun/19
a stroke of genius!
$${a}\:{stroke}\:{of}\:{genius}! \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *