Question-65347

Question Number 65347 by Masumsiddiqui399@gmail.com last updated on 28/Jul/19

Answered by MJS last updated on 28/Jul/19

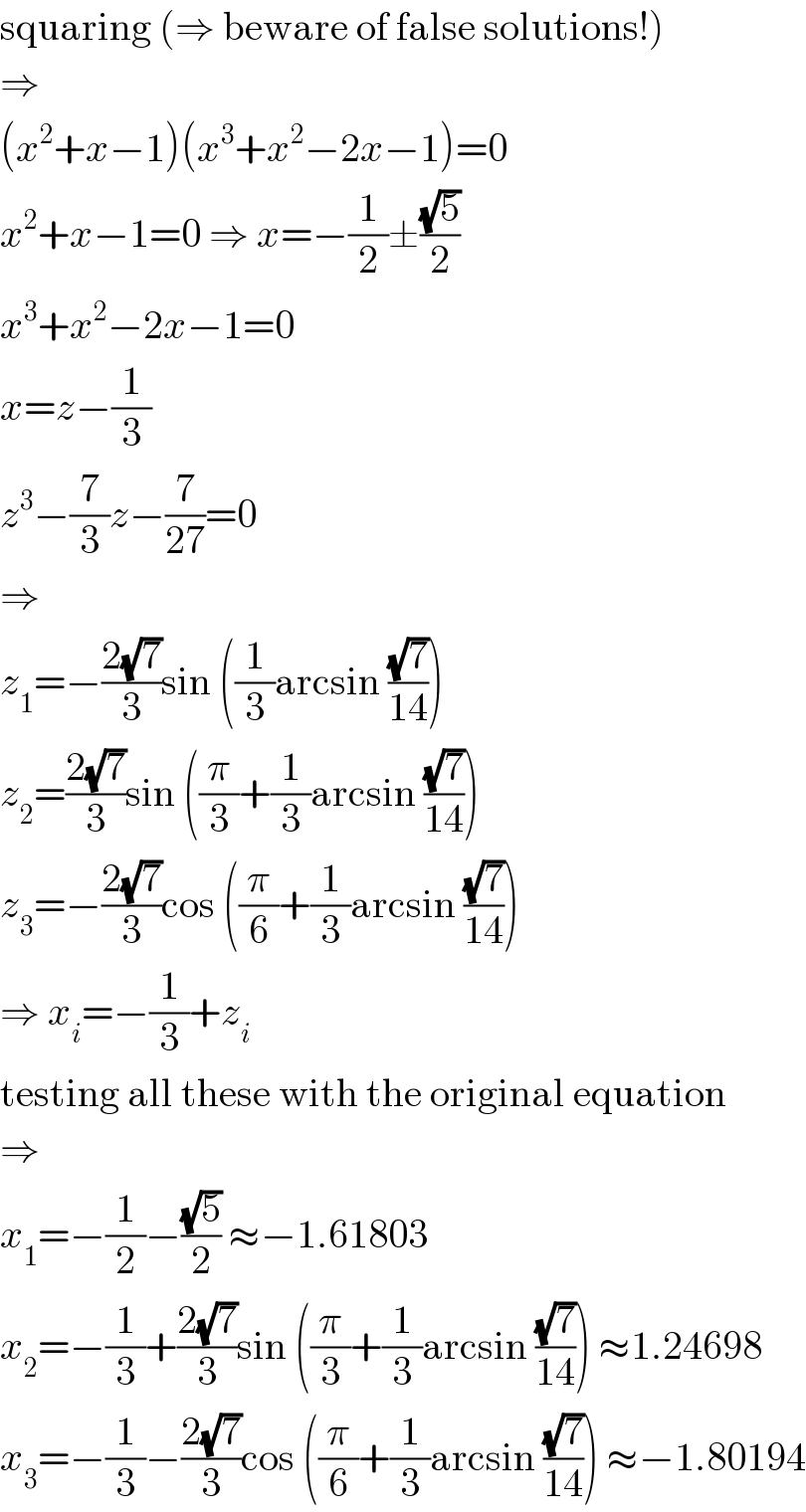
squaring (⇒ beware of false solutions!) ⇒ (x^2 +x−1)(x^3 +x^2 −2x−1)=0 x^2 +x−1=0 ⇒ x=−(1/2)±((√5)/2) x^3 +x^2 −2x−1=0 x=z−(1/3) z^3 −(7/3)z−(7/(27))=0 ⇒ z_1 =−((2(√7))/3)sin ((1/3)arcsin ((√7)/(14))) z_2 =((2(√7))/3)sin ((π/3)+(1/3)arcsin ((√7)/(14))) z_3 =−((2(√7))/3)cos ((π/6)+(1/3)arcsin ((√7)/(14))) ⇒ x_i =−(1/3)+z_i testing all these with the original equation ⇒ x_1 =−(1/2)−((√5)/2) ≈−1.61803 x_2 =−(1/3)+((2(√7))/3)sin ((π/3)+(1/3)arcsin ((√7)/(14))) ≈1.24698 x_3 =−(1/3)−((2(√7))/3)cos ((π/6)+(1/3)arcsin ((√7)/(14))) ≈−1.80194

$$\mathrm{squaring}\:\left(\Rightarrow\:\mathrm{beware}\:\mathrm{of}\:\mathrm{false}\:\mathrm{solutions}!\right) \\ $$$$\Rightarrow \\ $$$$\left({x}^{\mathrm{2}} +{x}−\mathrm{1}\right)\left({x}^{\mathrm{3}} +{x}^{\mathrm{2}} −\mathrm{2}{x}−\mathrm{1}\right)=\mathrm{0} \\ $$$${x}^{\mathrm{2}} +{x}−\mathrm{1}=\mathrm{0}\:\Rightarrow\:{x}=−\frac{\mathrm{1}}{\mathrm{2}}\pm\frac{\sqrt{\mathrm{5}}}{\mathrm{2}} \\ $$$${x}^{\mathrm{3}} +{x}^{\mathrm{2}} −\mathrm{2}{x}−\mathrm{1}=\mathrm{0} \\ $$$${x}={z}−\frac{\mathrm{1}}{\mathrm{3}} \\ $$$${z}^{\mathrm{3}} −\frac{\mathrm{7}}{\mathrm{3}}{z}−\frac{\mathrm{7}}{\mathrm{27}}=\mathrm{0} \\ $$$$\Rightarrow \\ $$$${z}_{\mathrm{1}} =−\frac{\mathrm{2}\sqrt{\mathrm{7}}}{\mathrm{3}}\mathrm{sin}\:\left(\frac{\mathrm{1}}{\mathrm{3}}\mathrm{arcsin}\:\frac{\sqrt{\mathrm{7}}}{\mathrm{14}}\right) \\ $$$${z}_{\mathrm{2}} =\frac{\mathrm{2}\sqrt{\mathrm{7}}}{\mathrm{3}}\mathrm{sin}\:\left(\frac{\pi}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{3}}\mathrm{arcsin}\:\frac{\sqrt{\mathrm{7}}}{\mathrm{14}}\right) \\ $$$${z}_{\mathrm{3}} =−\frac{\mathrm{2}\sqrt{\mathrm{7}}}{\mathrm{3}}\mathrm{cos}\:\left(\frac{\pi}{\mathrm{6}}+\frac{\mathrm{1}}{\mathrm{3}}\mathrm{arcsin}\:\frac{\sqrt{\mathrm{7}}}{\mathrm{14}}\right) \\ $$$$\Rightarrow\:{x}_{{i}} =−\frac{\mathrm{1}}{\mathrm{3}}+{z}_{{i}} \\ $$$$\mathrm{testing}\:\mathrm{all}\:\mathrm{these}\:\mathrm{with}\:\mathrm{the}\:\mathrm{original}\:\mathrm{equation} \\ $$$$\Rightarrow \\ $$$${x}_{\mathrm{1}} =−\frac{\mathrm{1}}{\mathrm{2}}−\frac{\sqrt{\mathrm{5}}}{\mathrm{2}}\:\approx−\mathrm{1}.\mathrm{61803} \\ $$$${x}_{\mathrm{2}} =−\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{2}\sqrt{\mathrm{7}}}{\mathrm{3}}\mathrm{sin}\:\left(\frac{\pi}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{3}}\mathrm{arcsin}\:\frac{\sqrt{\mathrm{7}}}{\mathrm{14}}\right)\:\approx\mathrm{1}.\mathrm{24698} \\ $$$${x}_{\mathrm{3}} =−\frac{\mathrm{1}}{\mathrm{3}}−\frac{\mathrm{2}\sqrt{\mathrm{7}}}{\mathrm{3}}\mathrm{cos}\:\left(\frac{\pi}{\mathrm{6}}+\frac{\mathrm{1}}{\mathrm{3}}\mathrm{arcsin}\:\frac{\sqrt{\mathrm{7}}}{\mathrm{14}}\right)\:\approx−\mathrm{1}.\mathrm{80194} \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply