Question Number 79340 by TawaTawa last updated on 24/Jan/20
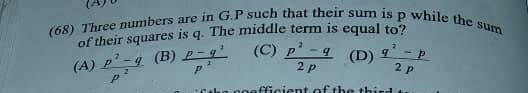
Commented by john santu last updated on 24/Jan/20
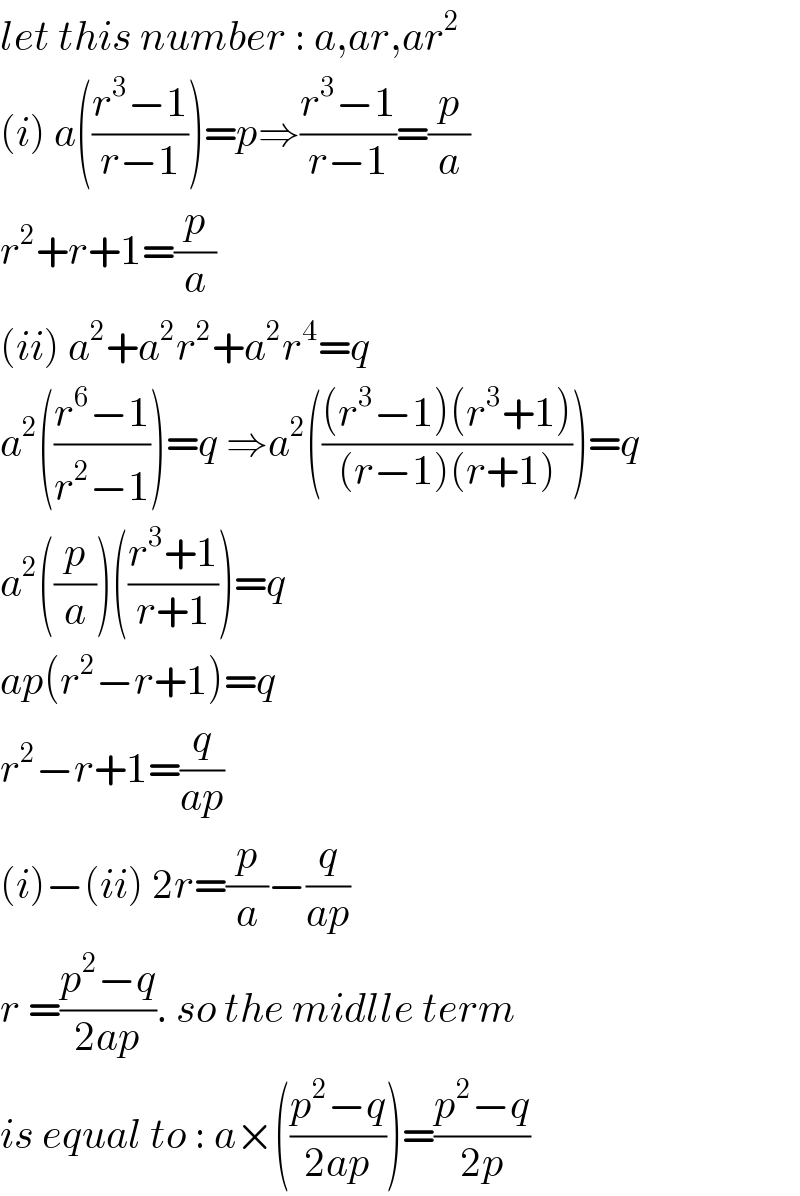
$${let}\:{this}\:{number}\::\:{a},{ar},{ar}^{\mathrm{2}} \\ $$$$\left({i}\right)\:{a}\left(\frac{{r}^{\mathrm{3}} −\mathrm{1}}{{r}−\mathrm{1}}\right)={p}\Rightarrow\frac{{r}^{\mathrm{3}} −\mathrm{1}}{{r}−\mathrm{1}}=\frac{{p}}{{a}} \\ $$$${r}^{\mathrm{2}} +{r}+\mathrm{1}=\frac{{p}}{{a}}\: \\ $$$$\left({ii}\right)\:{a}^{\mathrm{2}} +{a}^{\mathrm{2}} {r}^{\mathrm{2}} +{a}^{\mathrm{2}} {r}^{\mathrm{4}} ={q} \\ $$$${a}^{\mathrm{2}} \left(\frac{{r}^{\mathrm{6}} −\mathrm{1}}{{r}^{\mathrm{2}} −\mathrm{1}}\right)={q}\:\Rightarrow{a}^{\mathrm{2}} \left(\frac{\left({r}^{\mathrm{3}} −\mathrm{1}\right)\left({r}^{\mathrm{3}} +\mathrm{1}\right)}{\left({r}−\mathrm{1}\right)\left({r}+\mathrm{1}\right)}\right)={q} \\ $$$${a}^{\mathrm{2}} \left(\frac{{p}}{{a}}\right)\left(\frac{{r}^{\mathrm{3}} +\mathrm{1}}{{r}+\mathrm{1}}\right)={q} \\ $$$${ap}\left({r}^{\mathrm{2}} −{r}+\mathrm{1}\right)={q}\: \\ $$$${r}^{\mathrm{2}} −{r}+\mathrm{1}=\frac{{q}}{{ap}} \\ $$$$\left({i}\right)−\left({ii}\right)\:\mathrm{2}{r}=\frac{{p}}{{a}}−\frac{{q}}{{ap}} \\ $$$${r}\:=\frac{{p}^{\mathrm{2}} −{q}}{\mathrm{2}{ap}}.\:{so}\:{the}\:{midlle}\:{term} \\ $$$${is}\:{equal}\:{to}\::\:{a}×\left(\frac{{p}^{\mathrm{2}} −{q}}{\mathrm{2}{ap}}\right)=\frac{{p}^{\mathrm{2}} −{q}}{\mathrm{2}{p}} \\ $$
Commented by TawaTawa last updated on 24/Jan/20
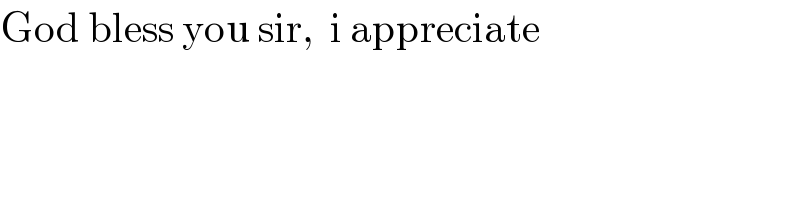
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir},\:\:\mathrm{i}\:\mathrm{appreciate} \\ $$
Commented by john santu last updated on 24/Jan/20

$${thanks}\:{you} \\ $$
Answered by john santu last updated on 24/Jan/20

$${ans}\:{C} \\ $$
