Question-80900

Question Number 80900 by ajfour last updated on 07/Feb/20

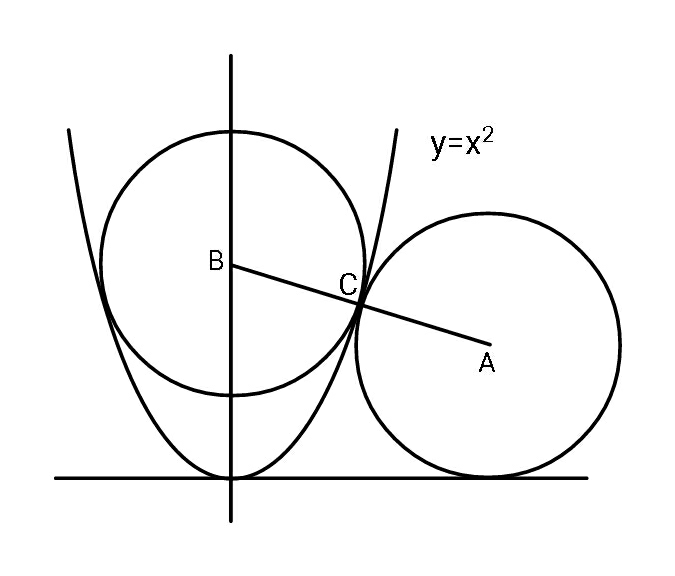
Commented by ajfour last updated on 07/Feb/20

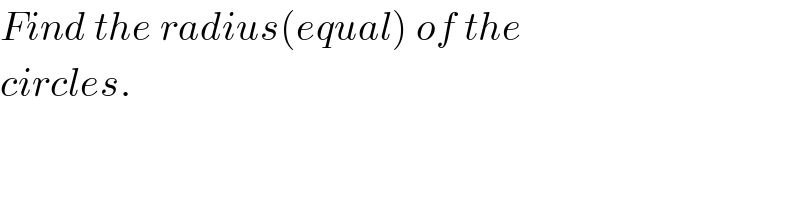
$${Find}\:{the}\:{radius}\left({equal}\right)\:{of}\:{the}\: \\ $$$${circles}. \\ $$

Answered by ajfour last updated on 07/Feb/20

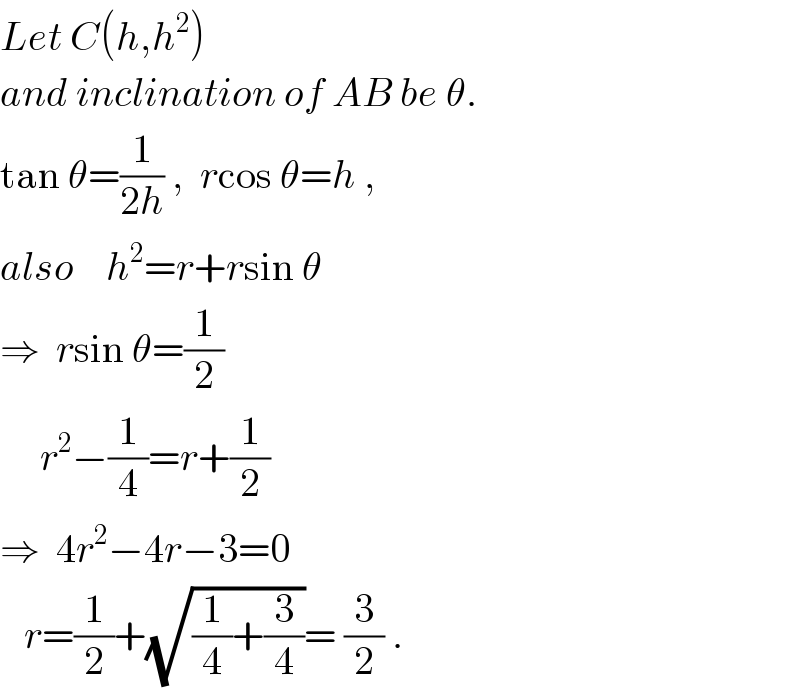
Let C(h,h^2 ) and inclination of AB be θ. tan θ=(1/(2h)) , rcos θ=h , also h^2 =r+rsin θ ⇒ rsin θ=(1/2) r^2 −(1/4)=r+(1/2) ⇒ 4r^2 −4r−3=0 r=(1/2)+(√((1/4)+(3/4)))= (3/2) .

$${Let}\:{C}\left({h},{h}^{\mathrm{2}} \right) \\ $$$${and}\:{inclination}\:{of}\:{AB}\:{be}\:\theta. \\ $$$$\mathrm{tan}\:\theta=\frac{\mathrm{1}}{\mathrm{2}{h}}\:,\:\:{r}\mathrm{cos}\:\theta={h}\:, \\ $$$${also}\:\:\:\:{h}^{\mathrm{2}} ={r}+{r}\mathrm{sin}\:\theta \\ $$$$\Rightarrow\:\:{r}\mathrm{sin}\:\theta=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\:\:\:\:\:{r}^{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{4}}={r}+\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\Rightarrow\:\:\mathrm{4}{r}^{\mathrm{2}} −\mathrm{4}{r}−\mathrm{3}=\mathrm{0} \\ $$$$\:\:\:{r}=\frac{\mathrm{1}}{\mathrm{2}}+\sqrt{\frac{\mathrm{1}}{\mathrm{4}}+\frac{\mathrm{3}}{\mathrm{4}}}=\:\frac{\mathrm{3}}{\mathrm{2}}\:. \\ $$

Commented by peter frank last updated on 08/Feb/20