Question Number 81654 by peter frank last updated on 14/Feb/20

Commented by peter frank last updated on 14/Feb/20
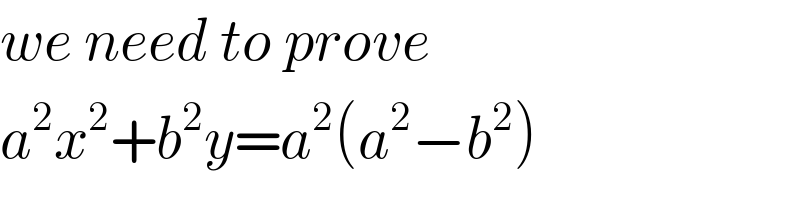
$${we}\:{need}\:{to}\:{prove}\: \\ $$$${a}^{\mathrm{2}} {x}^{\mathrm{2}} +{b}^{\mathrm{2}} {y}={a}^{\mathrm{2}} \left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right) \\ $$
Commented by peter frank last updated on 15/Feb/20
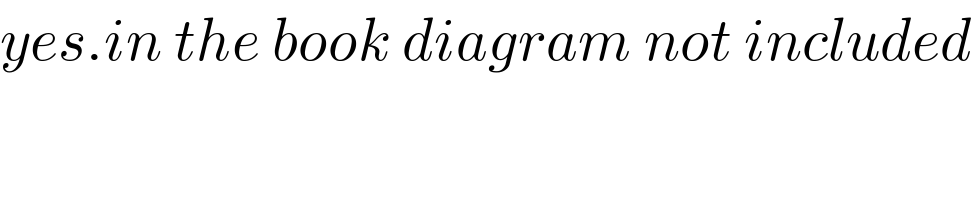
$${yes}.{in}\:{the}\:{book}\:{diagram}\:{not}\:{included} \\ $$
Commented by mr W last updated on 15/Feb/20

$${forget}\:{it}\:{sir}!\:{you}\:{also}\:{don}'{t}\:{know}\:{how}\:{the} \\ $$$${question}\:{is}\:{to}\:{understand}.\:{or}\:{can}\:{you}\: \\ $$$${show}\:{me}\:{a}\:{diagram}:\:{where}\:{is}\:{S}?\:\:{pass} \\ $$$${the}\:{perdendicular}\:{lines}\:{through}\:{the} \\ $$$${points}\:{D}\:{and}\:{P}?\:{where}\:{are}\:{the}\:{points} \\ $$$${D}\:{and}\:{P}?\: \\ $$
Commented by peter frank last updated on 15/Feb/20
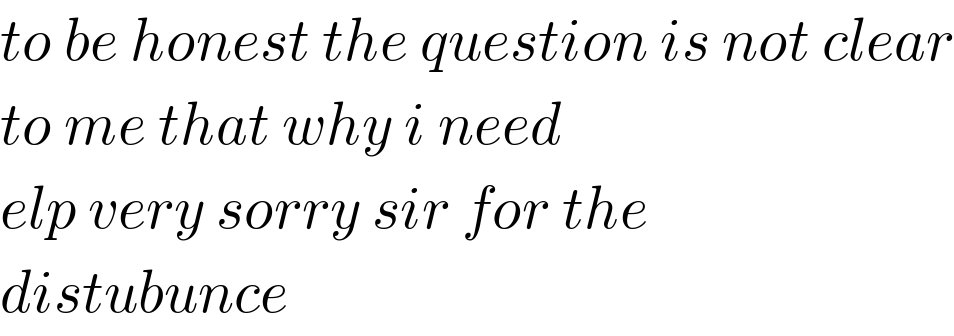
$${to}\:{be}\:{honest}\:{the}\:{question}\:{is}\:{not}\:{clear} \\ $$$${to}\:{me}\:{that}\:{why}\:{i}\:{need} \\ $$$${elp}\:{very}\:{sorry}\:{sir}\:{for}\:{the} \\ $$$${distubunce} \\ $$
Commented by peter frank last updated on 15/Feb/20
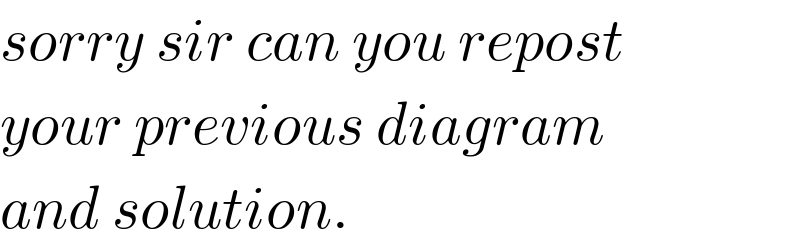
$${sorry}\:{sir}\:{can}\:{you}\:{repost} \\ $$$${your}\:{previous}\:{diagram} \\ $$$${and}\:{solution}. \\ $$
Commented by mr W last updated on 15/Feb/20
$${i}\:{have}\:{deleted},\:{because}\:{it}\:{doesn}'{t}\:{fit} \\ $$$${the}\:{question}. \\ $$
