Question Number 82431 by Power last updated on 21/Feb/20
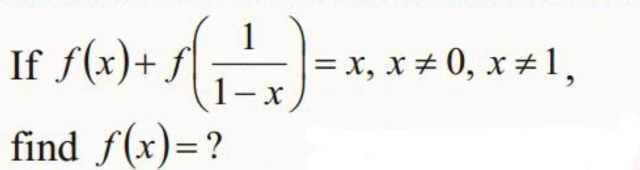
Commented by Power last updated on 21/Feb/20
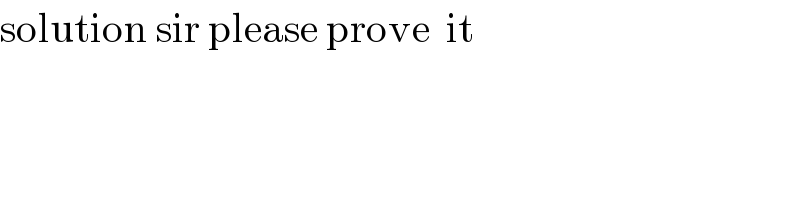
$$\mathrm{solution}\:\mathrm{sir}\:\mathrm{please}\:\mathrm{prove}\:\:\mathrm{it} \\ $$
Commented by mr W last updated on 21/Feb/20

$${what}'{s}\:{your}\:{result}\:{sir}?\:{f}\left({x}\right)=? \\ $$
Commented by mr W last updated on 21/Feb/20
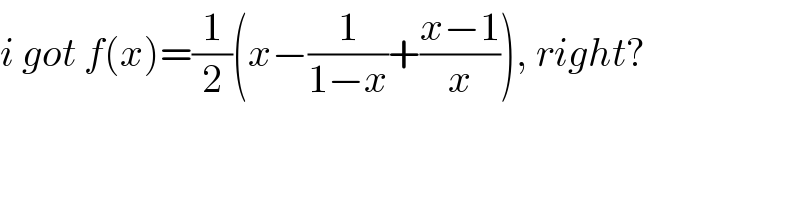
$${i}\:{got}\:{f}\left({x}\right)=\frac{\mathrm{1}}{\mathrm{2}}\left({x}−\frac{\mathrm{1}}{\mathrm{1}−{x}}+\frac{{x}−\mathrm{1}}{{x}}\right),\:{right}? \\ $$
Commented by Power last updated on 21/Feb/20

$$\mathrm{f}\left(\mathrm{x}\right)=\frac{\mathrm{x}^{\mathrm{3}} −\mathrm{x}+\mathrm{1}}{\mathrm{2x}\left(\mathrm{x}−\mathrm{1}\right)} \\ $$
Commented by mr W last updated on 21/Feb/20
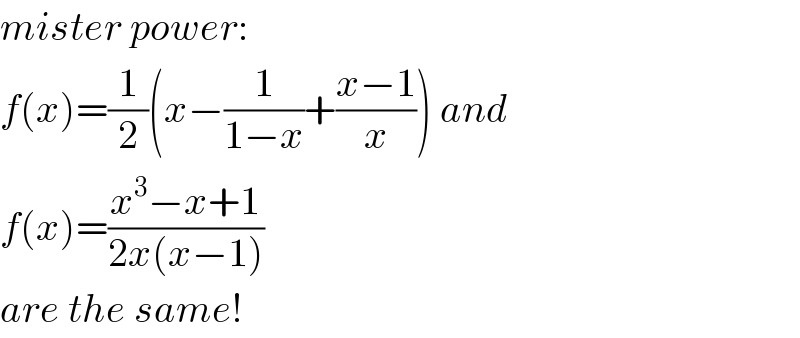
$${mister}\:{power}: \\ $$$${f}\left({x}\right)=\frac{\mathrm{1}}{\mathrm{2}}\left({x}−\frac{\mathrm{1}}{\mathrm{1}−{x}}+\frac{{x}−\mathrm{1}}{{x}}\right)\:{and}\: \\ $$$${f}\left({x}\right)=\frac{{x}^{\mathrm{3}} −{x}+\mathrm{1}}{\mathrm{2}{x}\left({x}−\mathrm{1}\right)} \\ $$$${are}\:{the}\:{same}! \\ $$
Answered by mr W last updated on 21/Feb/20
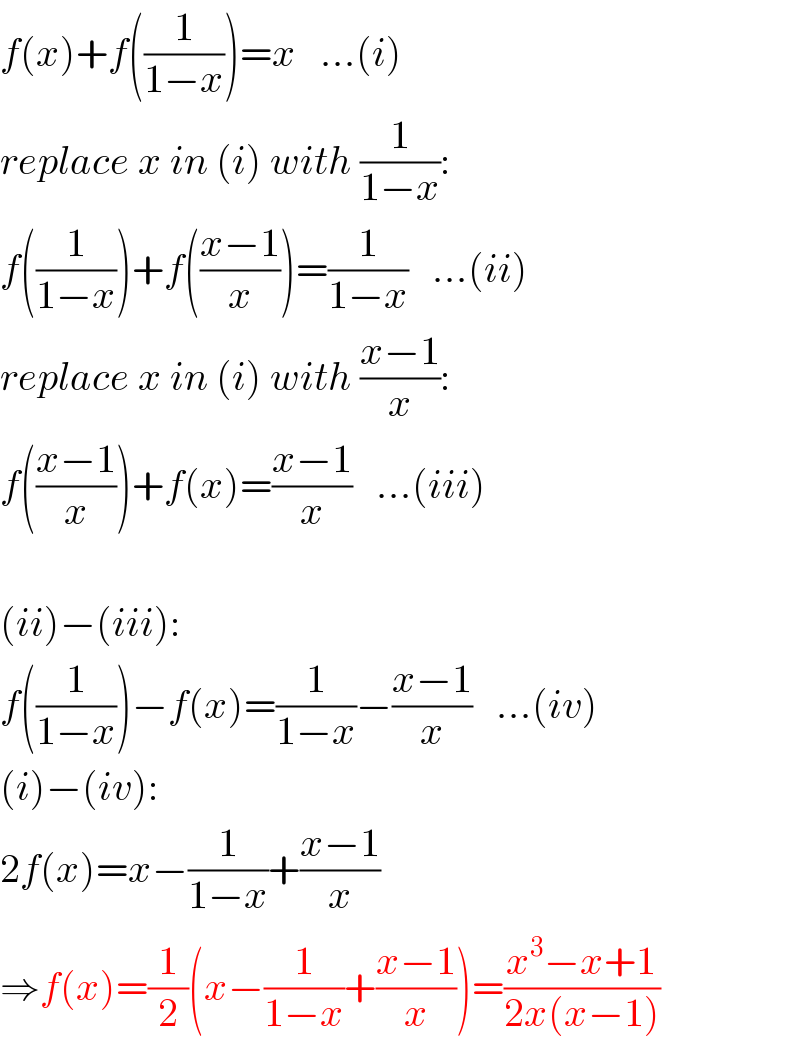
$${f}\left({x}\right)+{f}\left(\frac{\mathrm{1}}{\mathrm{1}−{x}}\right)={x}\:\:\:…\left({i}\right) \\ $$$${replace}\:{x}\:{in}\:\left({i}\right)\:{with}\:\frac{\mathrm{1}}{\mathrm{1}−{x}}: \\ $$$${f}\left(\frac{\mathrm{1}}{\mathrm{1}−{x}}\right)+{f}\left(\frac{{x}−\mathrm{1}}{{x}}\right)=\frac{\mathrm{1}}{\mathrm{1}−{x}}\:\:\:…\left({ii}\right) \\ $$$${replace}\:{x}\:{in}\:\left({i}\right)\:{with}\:\frac{{x}−\mathrm{1}}{{x}}: \\ $$$${f}\left(\frac{{x}−\mathrm{1}}{{x}}\right)+{f}\left({x}\right)=\frac{{x}−\mathrm{1}}{{x}}\:\:\:…\left({iii}\right) \\ $$$$ \\ $$$$\left({ii}\right)−\left({iii}\right): \\ $$$${f}\left(\frac{\mathrm{1}}{\mathrm{1}−{x}}\right)−{f}\left({x}\right)=\frac{\mathrm{1}}{\mathrm{1}−{x}}−\frac{{x}−\mathrm{1}}{{x}}\:\:\:…\left({iv}\right) \\ $$$$\left({i}\right)−\left({iv}\right): \\ $$$$\mathrm{2}{f}\left({x}\right)={x}−\frac{\mathrm{1}}{\mathrm{1}−{x}}+\frac{{x}−\mathrm{1}}{{x}} \\ $$$$\Rightarrow{f}\left({x}\right)=\frac{\mathrm{1}}{\mathrm{2}}\left({x}−\frac{\mathrm{1}}{\mathrm{1}−{x}}+\frac{{x}−\mathrm{1}}{{x}}\right)=\frac{{x}^{\mathrm{3}} −{x}+\mathrm{1}}{\mathrm{2}{x}\left({x}−\mathrm{1}\right)} \\ $$
Commented by Power last updated on 21/Feb/20
$$\mathrm{yes}\:\mathrm{thank}\:\mathrm{you}\:\mathrm{sir} \\ $$
