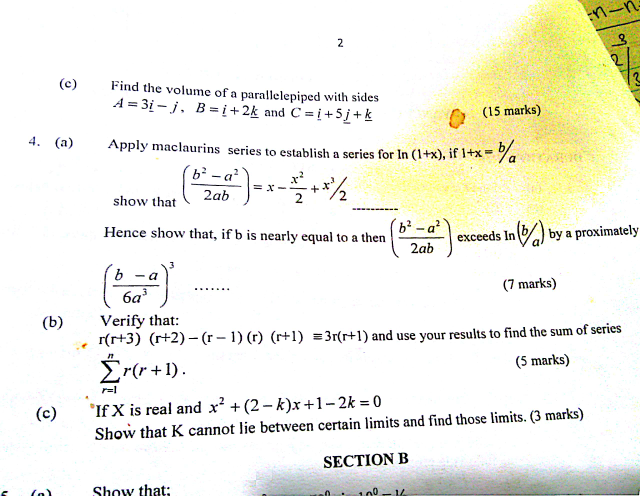
Question Number 83242 by peter frank last updated on 29/Feb/20
Commented by john santu last updated on 29/Feb/20
![(4b) Σ_(r = 1) ^n r(r+1) = Σ_(r = 1) ^n r^2 + Σ_(r = 1) ^n r = ((n(n+1)(2n+1))/6)+((n(n+1))/2) = ((n(n+1)(2n+1)+3n(n+1))/6) = ((n(n+1)[ 2n+1+3 ])/6) = ((n(n+1)(n+2))/3)](https://www.tinkutara.com/question/Q83248.png)
$$\left(\mathrm{4b}\right)\:\underset{\mathrm{r}\:=\:\mathrm{1}} {\overset{\mathrm{n}} {\sum}}\mathrm{r}\left(\mathrm{r}+\mathrm{1}\right)\:=\:\underset{\mathrm{r}\:=\:\mathrm{1}} {\overset{\mathrm{n}} {\sum}}\mathrm{r}^{\mathrm{2}} \:+\:\underset{\mathrm{r}\:=\:\mathrm{1}} {\overset{\mathrm{n}} {\sum}}\mathrm{r} \\ $$$$=\:\frac{\mathrm{n}\left(\mathrm{n}+\mathrm{1}\right)\left(\mathrm{2n}+\mathrm{1}\right)}{\mathrm{6}}+\frac{\mathrm{n}\left(\mathrm{n}+\mathrm{1}\right)}{\mathrm{2}} \\ $$$$=\:\frac{\mathrm{n}\left(\mathrm{n}+\mathrm{1}\right)\left(\mathrm{2n}+\mathrm{1}\right)+\mathrm{3n}\left(\mathrm{n}+\mathrm{1}\right)}{\mathrm{6}} \\ $$$$=\:\frac{\mathrm{n}\left(\mathrm{n}+\mathrm{1}\right)\left[\:\mathrm{2n}+\mathrm{1}+\mathrm{3}\:\right]}{\mathrm{6}} \\ $$$$=\:\frac{\mathrm{n}\left(\mathrm{n}+\mathrm{1}\right)\left(\mathrm{n}+\mathrm{2}\right)}{\mathrm{3}} \\ $$
Commented by peter frank last updated on 01/Mar/20

$$\mathrm{4}\:{a},{c} \\ $$
Commented by peter frank last updated on 01/Mar/20

$${thank}\:{you} \\ $$
