Question Number 84582 by jagoll last updated on 14/Mar/20

Commented by jagoll last updated on 14/Mar/20

$$\mathrm{dear}\:\mathrm{mr}\:\mathrm{W}.\:\mathrm{this}\:\mathrm{my}\:\mathrm{way}.\:\mathrm{it}'\mathrm{s} \\ $$$$\mathrm{correct}? \\ $$
Commented by jagoll last updated on 14/Mar/20

Commented by mr W last updated on 14/Mar/20

$${it}'{s}\:{not}\:{correct}\:{sir}!\:{the}\:{ellipse}\:{is}\:{just} \\ $$$${paned},\:{not}\:{rotated},\:{see}\:{picture}.\:{the} \\ $$$${correct}\:{image}\:{should}\:{be}\:{like}\:{the}\:{red} \\ $$$${one}. \\ $$
Commented by mr W last updated on 14/Mar/20

Commented by mr W last updated on 14/Mar/20

$${the}\:{image}\:{i}\:{got}: \\ $$
Commented by mr W last updated on 14/Mar/20

Commented by jagoll last updated on 14/Mar/20
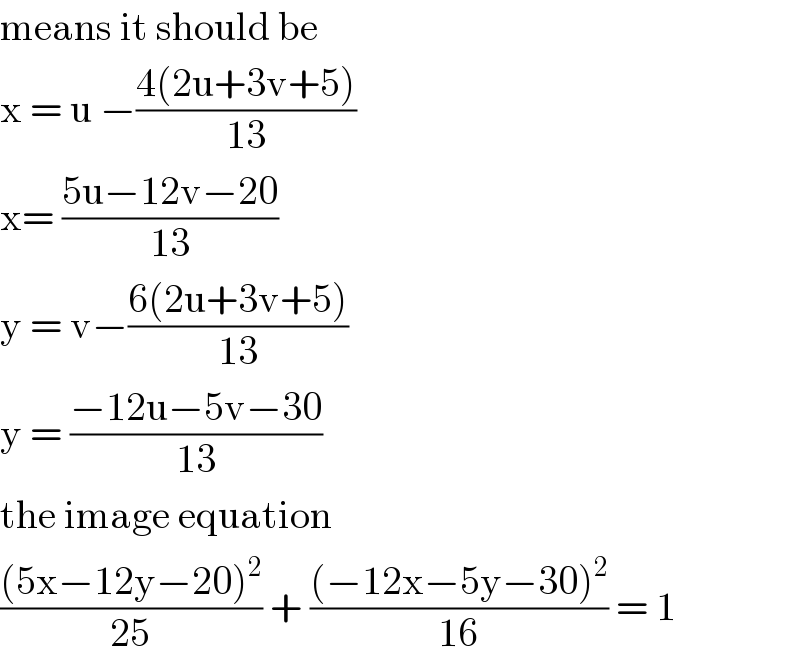
$$\mathrm{means}\:\mathrm{it}\:\mathrm{should}\:\mathrm{be}\: \\ $$$$\mathrm{x}\:=\:\mathrm{u}\:−\frac{\mathrm{4}\left(\mathrm{2u}+\mathrm{3v}+\mathrm{5}\right)}{\mathrm{13}}\: \\ $$$$\mathrm{x}=\:\frac{\mathrm{5u}−\mathrm{12v}−\mathrm{20}}{\mathrm{13}} \\ $$$$\mathrm{y}\:=\:\mathrm{v}−\frac{\mathrm{6}\left(\mathrm{2u}+\mathrm{3v}+\mathrm{5}\right)}{\mathrm{13}} \\ $$$$\mathrm{y}\:=\:\frac{−\mathrm{12u}−\mathrm{5v}−\mathrm{30}}{\mathrm{13}} \\ $$$$\mathrm{the}\:\mathrm{image}\:\mathrm{equation}\: \\ $$$$\frac{\left(\mathrm{5x}−\mathrm{12y}−\mathrm{20}\right)^{\mathrm{2}} }{\mathrm{25}}\:+\:\frac{\left(−\mathrm{12x}−\mathrm{5y}−\mathrm{30}\right)^{\mathrm{2}} }{\mathrm{16}}\:=\:\mathrm{1} \\ $$
Commented by jagoll last updated on 14/Mar/20

Commented by mr W last updated on 14/Mar/20

$${you}\:{forgot}\:{something}: \\ $$$$\frac{\left(\mathrm{5x}−\mathrm{12y}−\mathrm{20}\right)^{\mathrm{2}} }{\mathrm{25}×\mathrm{13}^{\mathrm{2}} }\:+\:\frac{\left(−\mathrm{12x}−\mathrm{5y}−\mathrm{30}\right)^{\mathrm{2}} }{\mathrm{16}×\mathrm{13}^{\mathrm{2}} }\:=\:\mathrm{1} \\ $$$${then}\:{it}'{s}\:{correct}. \\ $$
Commented by jagoll last updated on 14/Mar/20

$$\mathrm{ooo}…\mathrm{yes}.\:\mathrm{thank}\:\mathrm{you}\:\mathrm{sir}. \\ $$$$\mathrm{i}\:\mathrm{understand}\:\mathrm{now} \\ $$
