Question-85588

Question Number 85588 by TawaTawa1 last updated on 23/Mar/20

Commented by john santu last updated on 23/Mar/20

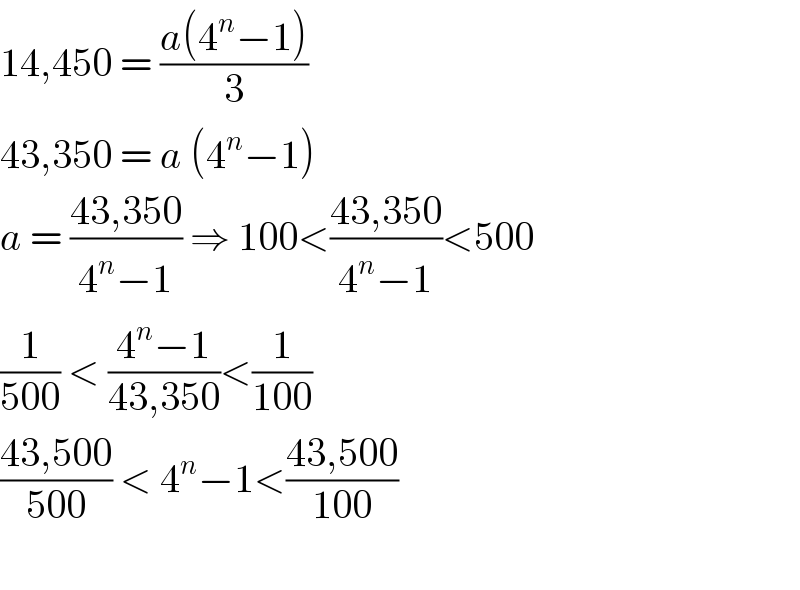
14,450 = ((a(4^n −1))/3) 43,350 = a (4^n −1) a = ((43,350)/(4^n −1)) ⇒ 100<((43,350)/(4^n −1))<500 (1/(500)) < ((4^n −1)/(43,350))<(1/(100)) ((43,500)/(500)) < 4^n −1<((43,500)/(100))

$$\mathrm{14},\mathrm{450}\:=\:\frac{{a}\left(\mathrm{4}^{{n}} −\mathrm{1}\right)}{\mathrm{3}} \\ $$$$\mathrm{43},\mathrm{350}\:=\:{a}\:\left(\mathrm{4}^{{n}} −\mathrm{1}\right) \\ $$$${a}\:=\:\frac{\mathrm{43},\mathrm{350}}{\mathrm{4}^{{n}} −\mathrm{1}}\:\Rightarrow\:\mathrm{100}<\frac{\mathrm{43},\mathrm{350}}{\mathrm{4}^{{n}} −\mathrm{1}}<\mathrm{500} \\ $$$$\frac{\mathrm{1}}{\mathrm{500}}\:<\:\frac{\mathrm{4}^{{n}} −\mathrm{1}}{\mathrm{43},\mathrm{350}}<\frac{\mathrm{1}}{\mathrm{100}} \\ $$$$\frac{\mathrm{43},\mathrm{500}}{\mathrm{500}}\:<\:\mathrm{4}^{{n}} −\mathrm{1}<\frac{\mathrm{43},\mathrm{500}}{\mathrm{100}} \\ $$$$ \\ $$

Commented by john santu last updated on 23/Mar/20

87<4^n −1<435 88 < 4^n < 436 log_4 (88) < n < log_4 (436)

$$\mathrm{87}<\mathrm{4}^{{n}} −\mathrm{1}<\mathrm{435} \\ $$$$\mathrm{88}\:<\:\mathrm{4}^{{n}} \:<\:\mathrm{436} \\ $$$$\mathrm{log}_{\mathrm{4}} \left(\mathrm{88}\right)\:<\:{n}\:<\:\mathrm{log}_{\mathrm{4}} \left(\mathrm{436}\right) \\ $$

Commented by TawaTawa1 last updated on 23/Mar/20

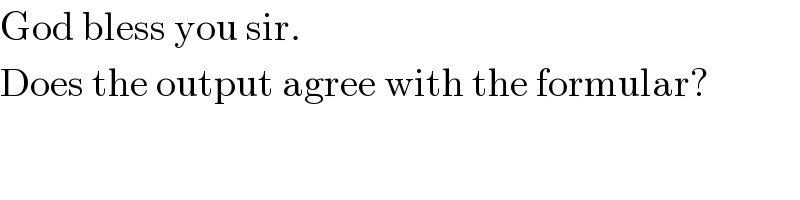
God bless you sir. Does the output agree with the formular?

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$$$\mathrm{Does}\:\mathrm{the}\:\mathrm{output}\:\mathrm{agree}\:\mathrm{with}\:\mathrm{the}\:\mathrm{formular}? \\ $$

Commented by jagoll last updated on 23/Mar/20

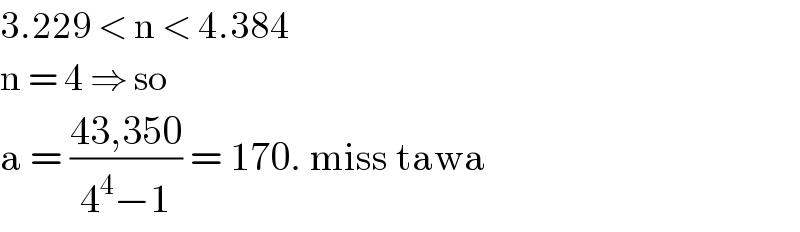
3.229 < n < 4.384 n = 4 ⇒ so a = ((43,350)/(4^4 −1)) = 170. miss tawa

$$\mathrm{3}.\mathrm{229}\:<\:\mathrm{n}\:<\:\mathrm{4}.\mathrm{384} \\ $$$$\mathrm{n}\:=\:\mathrm{4}\:\Rightarrow\:\mathrm{so}\: \\ $$$$\mathrm{a}\:=\:\frac{\mathrm{43},\mathrm{350}}{\mathrm{4}^{\mathrm{4}} −\mathrm{1}}\:=\:\mathrm{170}.\:\mathrm{miss}\:\mathrm{tawa} \\ $$

Commented by jagoll last updated on 23/Mar/20

the answer mr john is correct miss? i think is correct

$$\mathrm{the}\:\mathrm{answer}\:\mathrm{mr}\:\mathrm{john}\:\mathrm{is}\:\mathrm{correct}\:\mathrm{miss}? \\ $$$$\mathrm{i}\:\mathrm{think}\:\mathrm{is}\:\mathrm{correct} \\ $$

Commented by TawaTawa1 last updated on 23/Mar/20

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply