Question Number 87831 by aseer imad last updated on 06/Apr/20
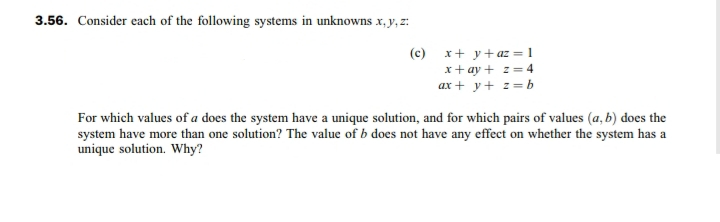
Commented by aseer imad last updated on 06/Apr/20
please solve the problem by row echilon form or reduced row echilon form.
TIA
Answered by arcana last updated on 06/Apr/20

$$\begin{pmatrix}{\mathrm{1}}&{\mathrm{1}}&{{a}}\\{\mathrm{1}}&{{a}}&{\mathrm{1}}\\{{a}}&{\mathrm{1}}&{\mathrm{1}}\end{pmatrix}\begin{pmatrix}{{x}}\\{{y}}\\{{z}}\end{pmatrix}\:=\:\begin{pmatrix}{\mathrm{1}}\\{\mathrm{4}}\\{{b}}\end{pmatrix} \\ $$$$ \\ $$$$\begin{pmatrix}{\mathrm{1}}&{\mathrm{1}}&{{a}}&{\mid}&{\mathrm{1}}\\{\mathrm{1}}&{{a}}&{\mathrm{1}}&{\mid}&{\mathrm{4}}\\{{a}}&{\mathrm{1}}&{\mathrm{1}}&{\mid}&{{b}}\end{pmatrix}\:\Rightarrow\begin{pmatrix}{\mathrm{1}}&{\mathrm{1}}&{{a}}&{\mid}&{\mathrm{1}}\\{\mathrm{0}}&{{a}−\mathrm{1}}&{\mathrm{1}−{a}}&{\mid}&{\mathrm{3}}\\{\mathrm{0}}&{\mathrm{1}−{a}}&{\mathrm{1}−{a}^{\mathrm{2}} }&{\mid}&{{b}−{a}}\end{pmatrix}\: \\ $$$$ \\ $$$$\Rightarrow\begin{pmatrix}{\mathrm{1}}&{\mathrm{1}}&{{a}}&{\mid}&{\mathrm{1}}\\{\mathrm{0}}&{{a}−\mathrm{1}}&{\mathrm{1}−{a}}&{\mid}&{\mathrm{3}}\\{\mathrm{0}}&{\mathrm{0}}&{\mathrm{2}−{a}−{a}^{\mathrm{2}} }&{\mid}&{{b}−{a}+\mathrm{3}}\end{pmatrix} \\ $$$$\mathrm{si}\:{a}=\mathrm{1}\:\mathrm{el}\:\mathrm{sistema}\:\mathrm{es}\:\mathrm{inconsistentente} \\ $$$$\mathrm{si}\:{a}=−\mathrm{2} \\ $$$$\bullet\mathrm{0}{x}+\mathrm{0}{y}+\:\mathrm{0}{z}=\mathrm{0}={b}+\mathrm{2}+\mathrm{3} \\ $$$$\Rightarrow{b}=−\mathrm{5} \\ $$$$\bullet\mathrm{0}{x}+\left(−\mathrm{2}−\mathrm{1}\right){y}+\left(\mathrm{1}−\left(−\mathrm{2}\right)\right){z}=\mathrm{3} \\ $$$$−{y}+{z}=\mathrm{1}\Rightarrow{z}=\mathrm{1}+{y} \\ $$$$\bullet{x}+{y}−\mathrm{2}{z}=\mathrm{1} \\ $$$${x}+{y}−\mathrm{2}−\mathrm{2}{y}={x}−{y}−\mathrm{2}=\mathrm{1} \\ $$$$\Rightarrow{x}={y}+\mathrm{3} \\ $$$$\mathrm{El}\:\mathrm{conjunto}\:\mathrm{de}\:\mathrm{soluciones}\:\mathrm{es} \\ $$$$\left\{\begin{pmatrix}{{x}}\\{{y}}\\{{z}}\end{pmatrix}\:\in\mathbb{R}^{\mathrm{3}} \::\:{z}=\mathrm{1}+{y}\:\wedge\:\:{x}={y}+\mathrm{3}\right\}= \\ $$$$\left\{\begin{pmatrix}{\mathrm{1}}\\{\mathrm{1}}\\{\mathrm{1}}\end{pmatrix}\:{y}\:+\begin{pmatrix}{\mathrm{3}}\\{\mathrm{0}}\\{\mathrm{1}}\end{pmatrix}\:\::\:\forall{y}\in\mathbb{R}\right\} \\ $$$$\mathrm{el}\:\mathrm{par}\:\left(−\mathrm{2},−\mathrm{5}\right)\:\mathrm{hace}\:\mathrm{que}\:\mathrm{el}\:\mathrm{sistema}\:\mathrm{tenga} \\ $$$$\mathrm{mas}\:\mathrm{de}\:\mathrm{una}\:\mathrm{solucion}.\:\mathrm{El}\:\mathrm{sistema}\:\mathrm{posee} \\ $$$$\mathrm{unica}\:\mathrm{solucion}\:\mathrm{para}\:{a}\in\mathbb{R}\backslash\left\{−\mathrm{2},\mathrm{1}\right\} \\ $$$$ \\ $$
