Question-88021

Question Number 88021 by naka3546 last updated on 07/Apr/20

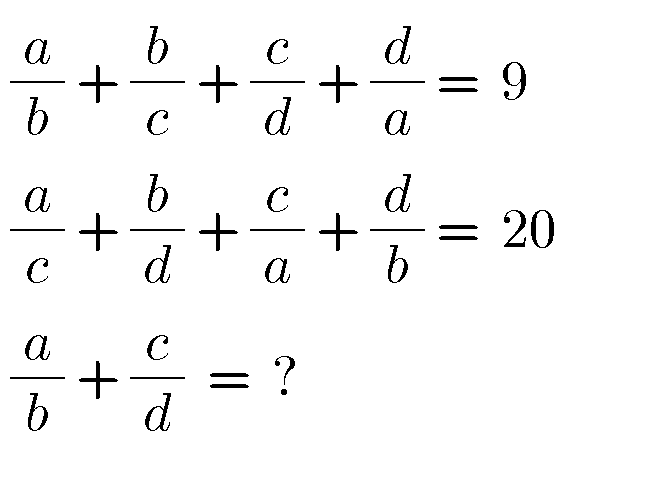
Commented by MJS last updated on 07/Apr/20

I get 4 \lor 5 \dots not sure how I got there \dots

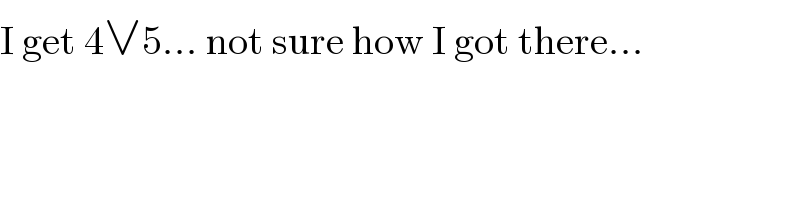
Commented by naka3546 last updated on 08/Apr/20

{y o u}^{'} r e r i g h t, s i r .

Answered by $@ty@m123 last updated on 08/Apr/20

p + q + r + s = 9 {w h e r e p = \frac{a}{b} a n d s o o n

p q + q r + r s + s p = 20

\Rightarrow (p + r) (q + s) = 20

E q u a t i o n w h o s e r o o t s a r e p + r, q + s i s

x^{2} - 9 x + 20 = 0

x = 4, 5

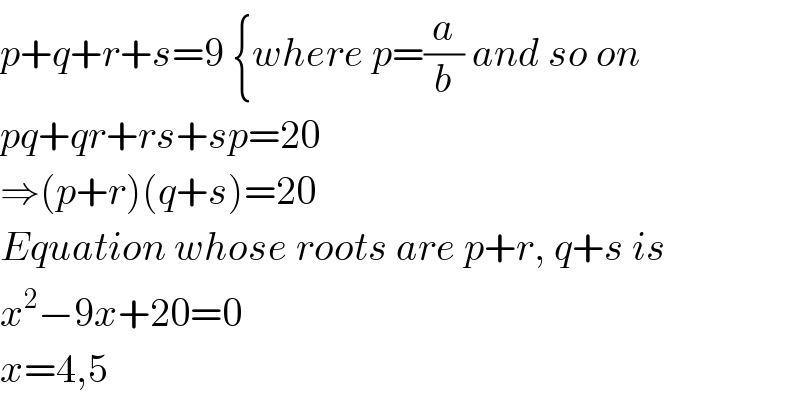
Commented by Prithwish Sen 1 last updated on 08/Apr/20

I think it will be

(p + r) (q + s) = 20

Commented by $@ty@m123 last updated on 08/Apr/20

T h a n k s f o r c o r r e c t i o n .