Question-90069

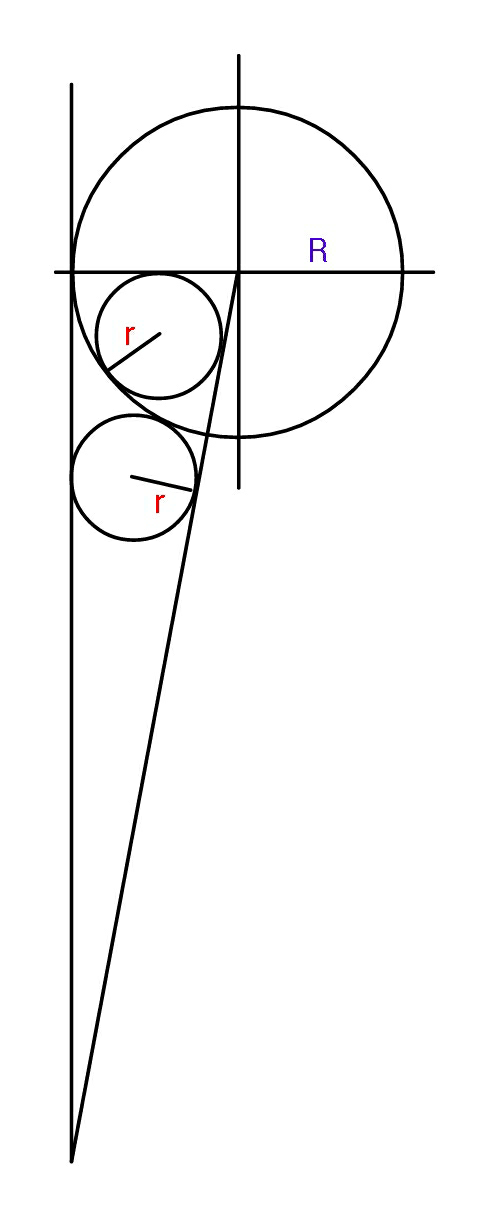
Question Number 90069 by ajfour last updated on 21/Apr/20

Commented by ajfour last updated on 21/Apr/20

dont know why i get two answers; (r/R) ≈ 0.49172, 0.39253

$${dont}\:{know}\:{why}\:{i}\:{get}\:{two}\:{answers}; \\ $$$$\frac{{r}}{{R}}\:\approx\:\mathrm{0}.\mathrm{49172},\:\mathrm{0}.\mathrm{39253} \\ $$

Commented by ajfour last updated on 21/Apr/20

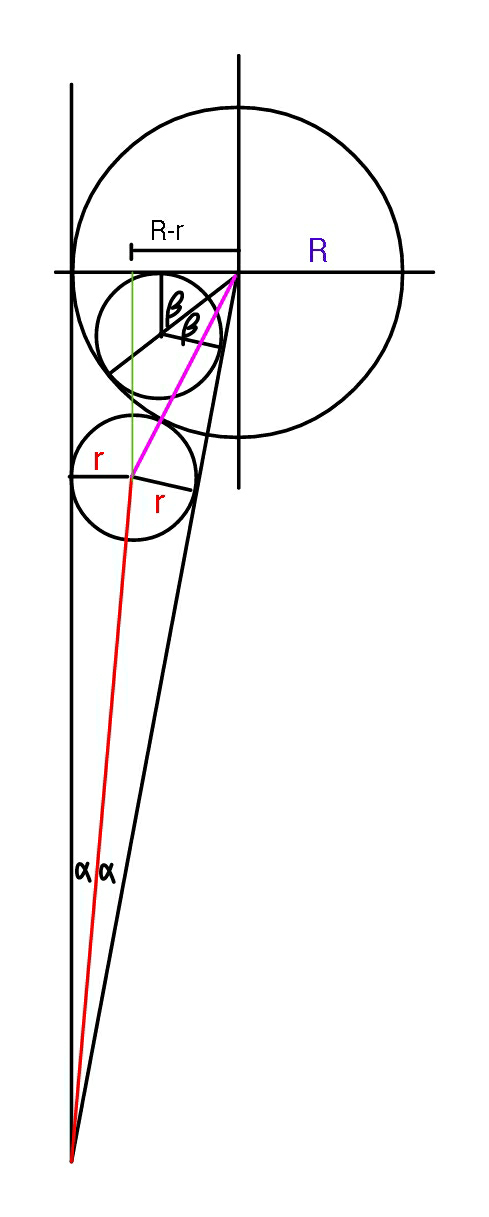
$${Find}\:{r}/{R}. \\ $$

Commented by ajfour last updated on 21/Apr/20

$${mrW}\:{Sir},\:{please}\:{attempt}.. \\ $$

Answered by mr W last updated on 21/Apr/20

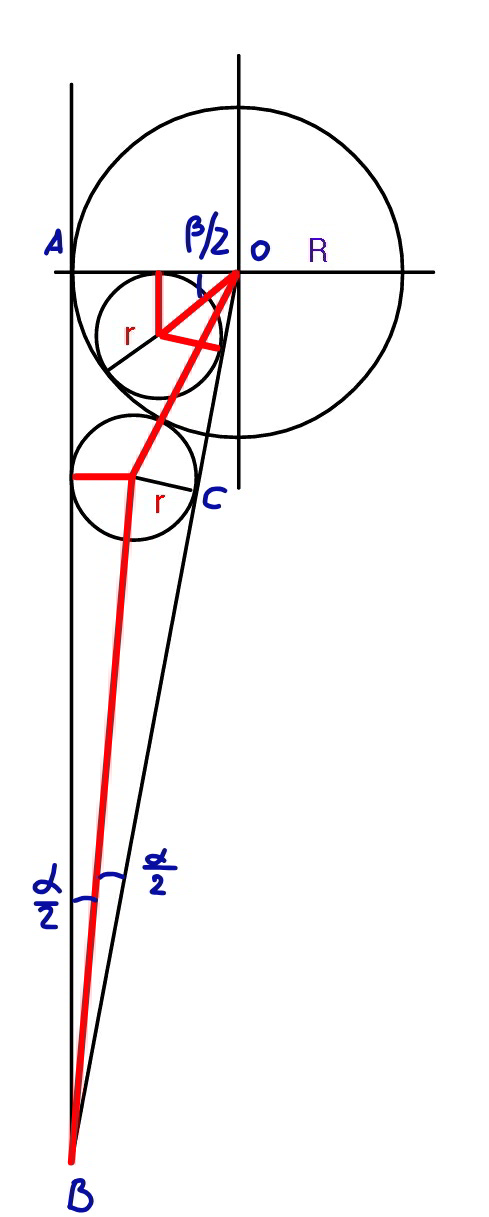
Commented by mr W last updated on 21/Apr/20

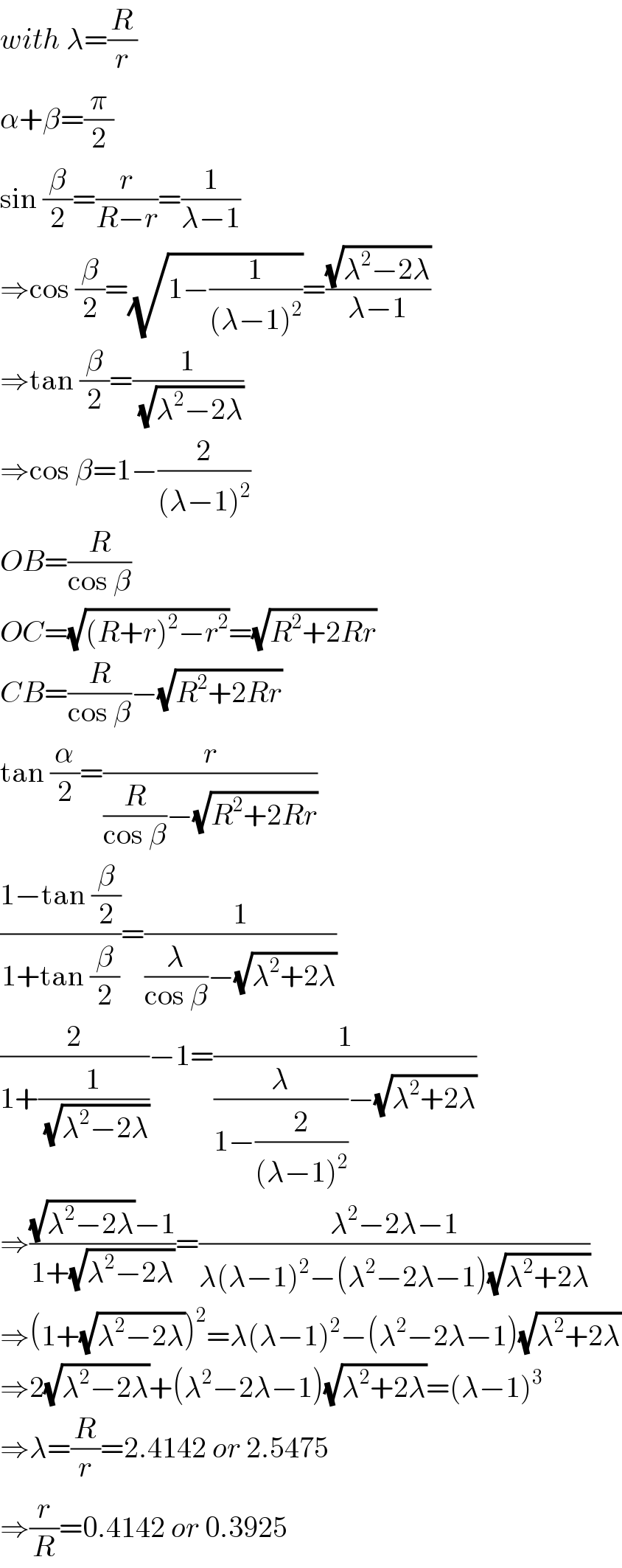
with λ=(R/r) α+β=(π/2) sin (β/2)=(r/(R−r))=(1/(λ−1)) ⇒cos (β/2)=(√(1−(1/((λ−1)^2 ))))=((√(λ^2 −2λ))/(λ−1)) ⇒tan (β/2)=(1/( (√(λ^2 −2λ)))) ⇒cos β=1−(2/((λ−1)^2 )) OB=(R/(cos β)) OC=(√((R+r)^2 −r^2 ))=(√(R^2 +2Rr)) CB=(R/(cos β))−(√(R^2 +2Rr)) tan (α/2)=(r/((R/(cos β))−(√(R^2 +2Rr)))) ((1−tan (β/2))/(1+tan (β/2)))=(1/((λ/(cos β))−(√(λ^2 +2λ)))) (2/(1+(1/( (√(λ^2 −2λ))))))−1=(1/((λ/(1−(2/((λ−1)^2 ))))−(√(λ^2 +2λ)))) ⇒(((√(λ^2 −2λ))−1)/(1+(√(λ^2 −2λ))))=((λ^2 −2λ−1)/(λ(λ−1)^2 −(λ^2 −2λ−1)(√(λ^2 +2λ)))) ⇒(1+(√(λ^2 −2λ)))^2 =λ(λ−1)^2 −(λ^2 −2λ−1)(√(λ^2 +2λ)) ⇒2(√(λ^2 −2λ))+(λ^2 −2λ−1)(√(λ^2 +2λ))=(λ−1)^3 ⇒λ=(R/r)=2.4142 or 2.5475 ⇒(r/R)=0.4142 or 0.3925

$${with}\:\lambda=\frac{{R}}{{r}} \\ $$$$\alpha+\beta=\frac{\pi}{\mathrm{2}} \\ $$$$\mathrm{sin}\:\frac{\beta}{\mathrm{2}}=\frac{{r}}{{R}−{r}}=\frac{\mathrm{1}}{\lambda−\mathrm{1}} \\ $$$$\Rightarrow\mathrm{cos}\:\frac{\beta}{\mathrm{2}}=\sqrt{\mathrm{1}−\frac{\mathrm{1}}{\left(\lambda−\mathrm{1}\right)^{\mathrm{2}} }}=\frac{\sqrt{\lambda^{\mathrm{2}} −\mathrm{2}\lambda}}{\lambda−\mathrm{1}} \\ $$$$\Rightarrow\mathrm{tan}\:\frac{\beta}{\mathrm{2}}=\frac{\mathrm{1}}{\:\sqrt{\lambda^{\mathrm{2}} −\mathrm{2}\lambda}} \\ $$$$\Rightarrow\mathrm{cos}\:\beta=\mathrm{1}−\frac{\mathrm{2}}{\left(\lambda−\mathrm{1}\right)^{\mathrm{2}} } \\ $$$${OB}=\frac{{R}}{\mathrm{cos}\:\beta} \\ $$$${OC}=\sqrt{\left({R}+{r}\right)^{\mathrm{2}} −{r}^{\mathrm{2}} }=\sqrt{{R}^{\mathrm{2}} +\mathrm{2}{Rr}} \\ $$$${CB}=\frac{{R}}{\mathrm{cos}\:\beta}−\sqrt{{R}^{\mathrm{2}} +\mathrm{2}{Rr}} \\ $$$$\mathrm{tan}\:\frac{\alpha}{\mathrm{2}}=\frac{{r}}{\frac{{R}}{\mathrm{cos}\:\beta}−\sqrt{{R}^{\mathrm{2}} +\mathrm{2}{Rr}}} \\ $$$$\frac{\mathrm{1}−\mathrm{tan}\:\frac{\beta}{\mathrm{2}}}{\mathrm{1}+\mathrm{tan}\:\frac{\beta}{\mathrm{2}}}=\frac{\mathrm{1}}{\frac{\lambda}{\mathrm{cos}\:\beta}−\sqrt{\lambda^{\mathrm{2}} +\mathrm{2}\lambda}} \\ $$$$\frac{\mathrm{2}}{\mathrm{1}+\frac{\mathrm{1}}{\:\sqrt{\lambda^{\mathrm{2}} −\mathrm{2}\lambda}}}−\mathrm{1}=\frac{\mathrm{1}}{\frac{\lambda}{\mathrm{1}−\frac{\mathrm{2}}{\left(\lambda−\mathrm{1}\right)^{\mathrm{2}} }}−\sqrt{\lambda^{\mathrm{2}} +\mathrm{2}\lambda}} \\ $$$$\Rightarrow\frac{\sqrt{\lambda^{\mathrm{2}} −\mathrm{2}\lambda}−\mathrm{1}}{\mathrm{1}+\sqrt{\lambda^{\mathrm{2}} −\mathrm{2}\lambda}}=\frac{\lambda^{\mathrm{2}} −\mathrm{2}\lambda−\mathrm{1}}{\lambda\left(\lambda−\mathrm{1}\right)^{\mathrm{2}} −\left(\lambda^{\mathrm{2}} −\mathrm{2}\lambda−\mathrm{1}\right)\sqrt{\lambda^{\mathrm{2}} +\mathrm{2}\lambda}} \\ $$$$\Rightarrow\left(\mathrm{1}+\sqrt{\lambda^{\mathrm{2}} −\mathrm{2}\lambda}\right)^{\mathrm{2}} =\lambda\left(\lambda−\mathrm{1}\right)^{\mathrm{2}} −\left(\lambda^{\mathrm{2}} −\mathrm{2}\lambda−\mathrm{1}\right)\sqrt{\lambda^{\mathrm{2}} +\mathrm{2}\lambda} \\ $$$$\Rightarrow\mathrm{2}\sqrt{\lambda^{\mathrm{2}} −\mathrm{2}\lambda}+\left(\lambda^{\mathrm{2}} −\mathrm{2}\lambda−\mathrm{1}\right)\sqrt{\lambda^{\mathrm{2}} +\mathrm{2}\lambda}=\left(\lambda−\mathrm{1}\right)^{\mathrm{3}} \\ $$$$\Rightarrow\lambda=\frac{{R}}{{r}}=\mathrm{2}.\mathrm{4142}\:{or}\:\mathrm{2}.\mathrm{5475} \\ $$$$\Rightarrow\frac{{r}}{{R}}=\mathrm{0}.\mathrm{4142}\:{or}\:\mathrm{0}.\mathrm{3925} \\ $$

Commented by ajfour last updated on 21/Apr/20

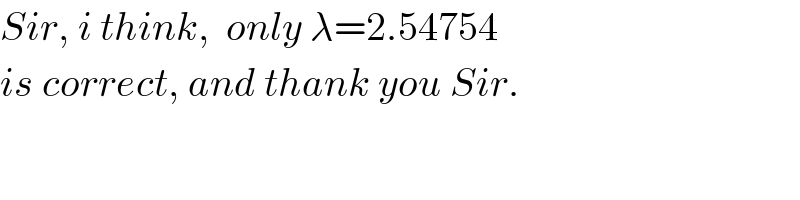
Sir, i think, only λ=2.54754 is correct, and thank you Sir.

$${Sir},\:{i}\:{think},\:\:{only}\:\lambda=\mathrm{2}.\mathrm{54754} \\ $$$${is}\:{correct},\:{and}\:{thank}\:{you}\:{Sir}. \\ $$

Answered by ajfour last updated on 21/Apr/20

Commented by ajfour last updated on 21/Apr/20

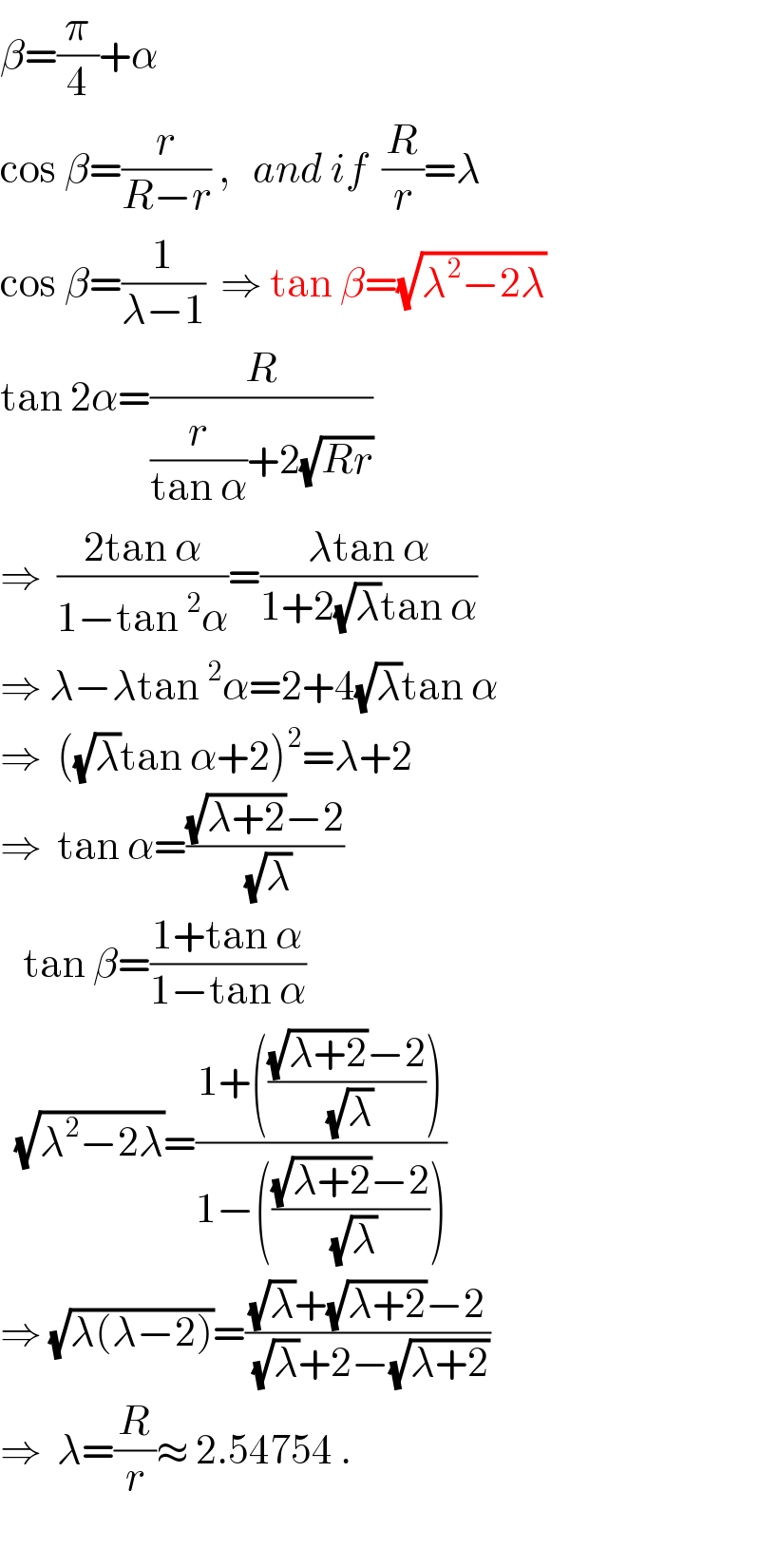
β=(π/4)+α cos β=(r/(R−r)) , and if (R/r)=λ cos β=(1/(λ−1)) ⇒ tan β=(√(λ^2 −2λ)) tan 2α=(R/((r/(tan α))+2(√(Rr)))) ⇒ ((2tan α)/(1−tan^2 α))=((λtan α)/(1+2(√λ)tan α)) ⇒ λ−λtan^2 α=2+4(√λ)tan α ⇒ ((√λ)tan α+2)^2 =λ+2 ⇒ tan α=(((√(λ+2))−2)/( (√λ))) tan β=((1+tan α)/(1−tan α)) (√(λ^2 −2λ))=((1+((((√(λ+2))−2)/( (√λ)))))/(1−((((√(λ+2))−2)/( (√λ)))))) ⇒ (√(λ(λ−2)))=(((√λ)+(√(λ+2))−2)/( (√λ)+2−(√(λ+2)))) ⇒ λ=(R/r)≈ 2.54754 .

$$\beta=\frac{\pi}{\mathrm{4}}+\alpha \\ $$$$\mathrm{cos}\:\beta=\frac{{r}}{{R}−{r}}\:,\:\:\:{and}\:{if}\:\:\frac{{R}}{{r}}=\lambda \\ $$$$\mathrm{cos}\:\beta=\frac{\mathrm{1}}{\lambda−\mathrm{1}}\:\:\Rightarrow\:\mathrm{tan}\:\beta=\sqrt{\lambda^{\mathrm{2}} −\mathrm{2}\lambda} \\ $$$$\mathrm{tan}\:\mathrm{2}\alpha=\frac{{R}}{\frac{{r}}{\mathrm{tan}\:\alpha}+\mathrm{2}\sqrt{{Rr}}} \\ $$$$\Rightarrow\:\:\frac{\mathrm{2tan}\:\alpha}{\mathrm{1}−\mathrm{tan}\:^{\mathrm{2}} \alpha}=\frac{\lambda\mathrm{tan}\:\alpha}{\mathrm{1}+\mathrm{2}\sqrt{\lambda}\mathrm{tan}\:\alpha} \\ $$$$\Rightarrow\:\lambda−\lambda\mathrm{tan}\:^{\mathrm{2}} \alpha=\mathrm{2}+\mathrm{4}\sqrt{\lambda}\mathrm{tan}\:\alpha \\ $$$$\Rightarrow\:\:\left(\sqrt{\lambda}\mathrm{tan}\:\alpha+\mathrm{2}\right)^{\mathrm{2}} =\lambda+\mathrm{2} \\ $$$$\Rightarrow\:\:\mathrm{tan}\:\alpha=\frac{\sqrt{\lambda+\mathrm{2}}−\mathrm{2}}{\:\sqrt{\lambda}} \\ $$$$\:\:\:\mathrm{tan}\:\beta=\frac{\mathrm{1}+\mathrm{tan}\:\alpha}{\mathrm{1}−\mathrm{tan}\:\alpha} \\ $$$$\:\:\sqrt{\lambda^{\mathrm{2}} −\mathrm{2}\lambda}=\frac{\mathrm{1}+\left(\frac{\sqrt{\lambda+\mathrm{2}}−\mathrm{2}}{\:\sqrt{\lambda}}\right)}{\mathrm{1}−\left(\frac{\sqrt{\lambda+\mathrm{2}}−\mathrm{2}}{\:\sqrt{\lambda}}\right)} \\ $$$$\Rightarrow\:\sqrt{\lambda\left(\lambda−\mathrm{2}\right)}=\frac{\sqrt{\lambda}+\sqrt{\lambda+\mathrm{2}}−\mathrm{2}}{\:\sqrt{\lambda}+\mathrm{2}−\sqrt{\lambda+\mathrm{2}}} \\ $$$$\Rightarrow\:\:\lambda=\frac{{R}}{{r}}\approx\:\mathrm{2}.\mathrm{54754}\:. \\ $$$$\:\: \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply