Menu Close

Question-90252

Tinku Tara 0 Comments
Pin




Question Number 90252 by ajfour last updated on 22/Apr/20
Commented by ajfour last updated on 22/Apr/20
If both ellipses have the same shape, find, b/a .Also find circumradius in terms of a.
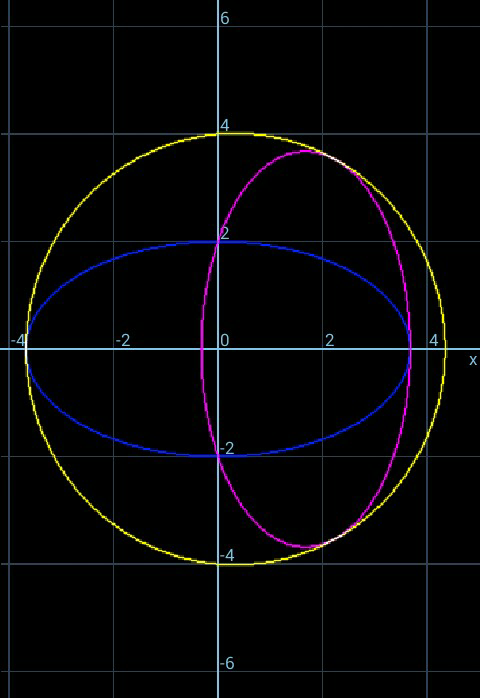
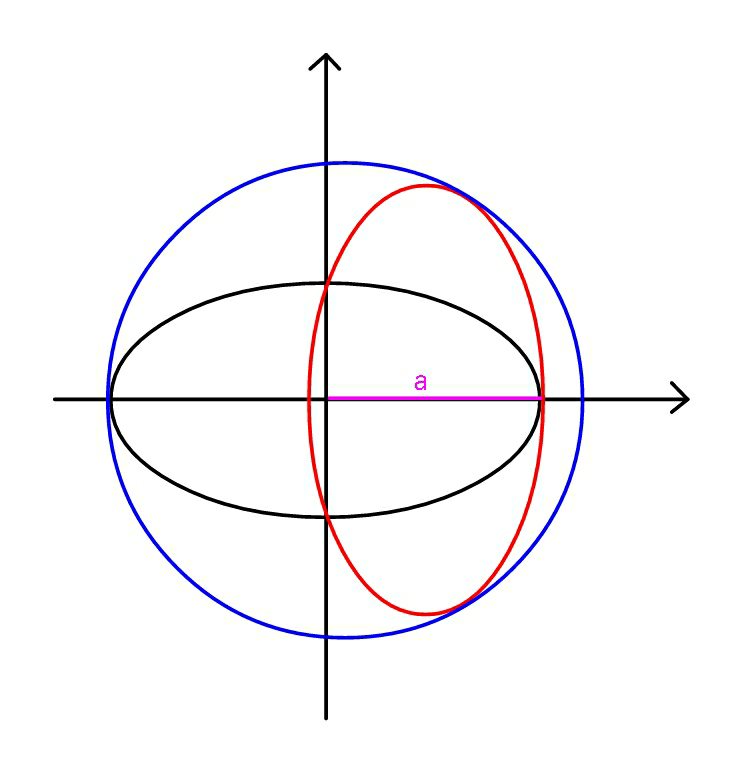
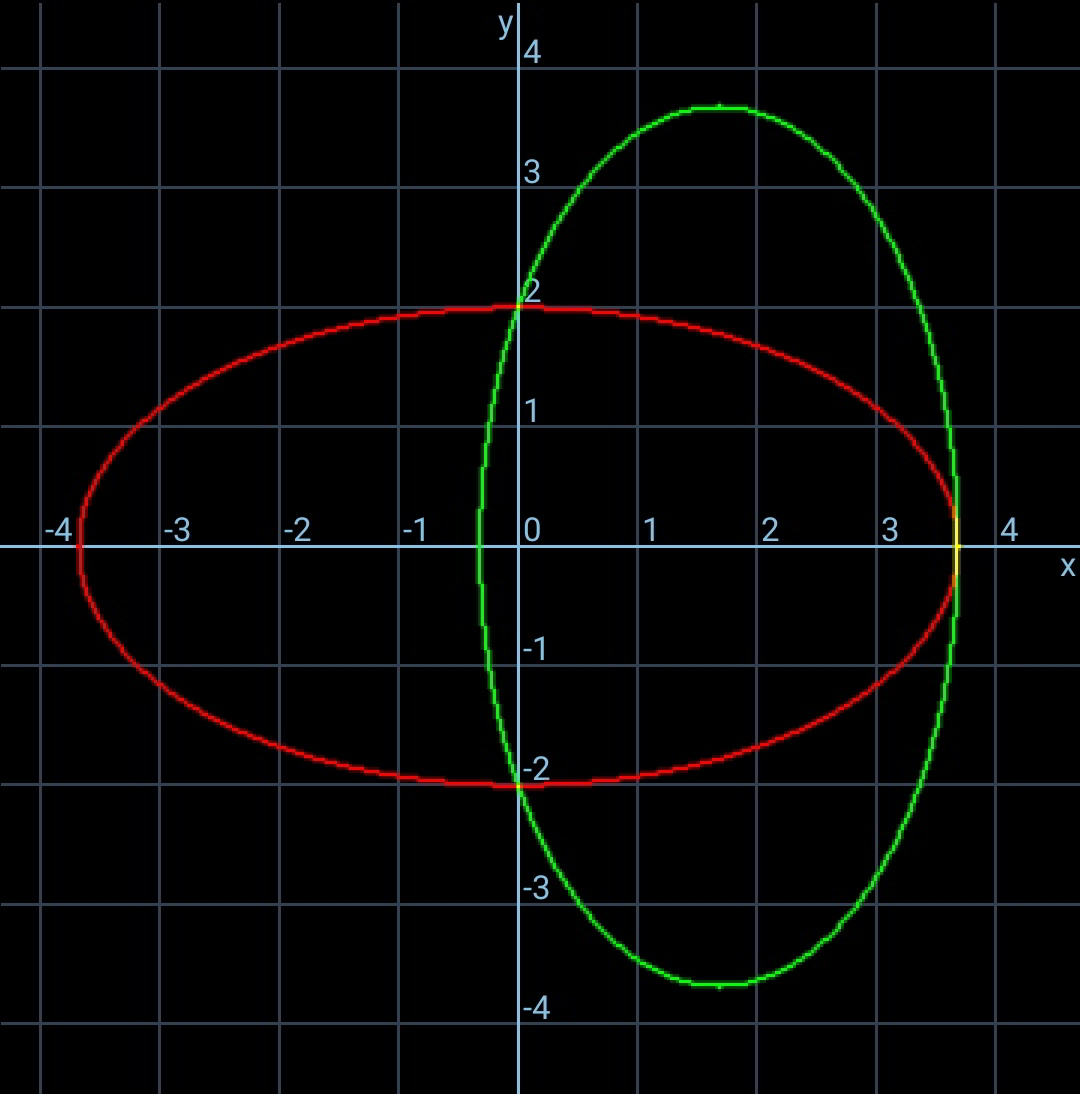
$${If}\:{both}\:{ellipses}\:{have}\:{the}\:{same} \\ $$$${shape},\:{find},\:{b}/{a}\:.{Also}\:{find} \\ $$$${circumradius}\:{in}\:{terms}\:{of}\:{a}. \\ $$
Answered by ajfour last updated on 22/Apr/20
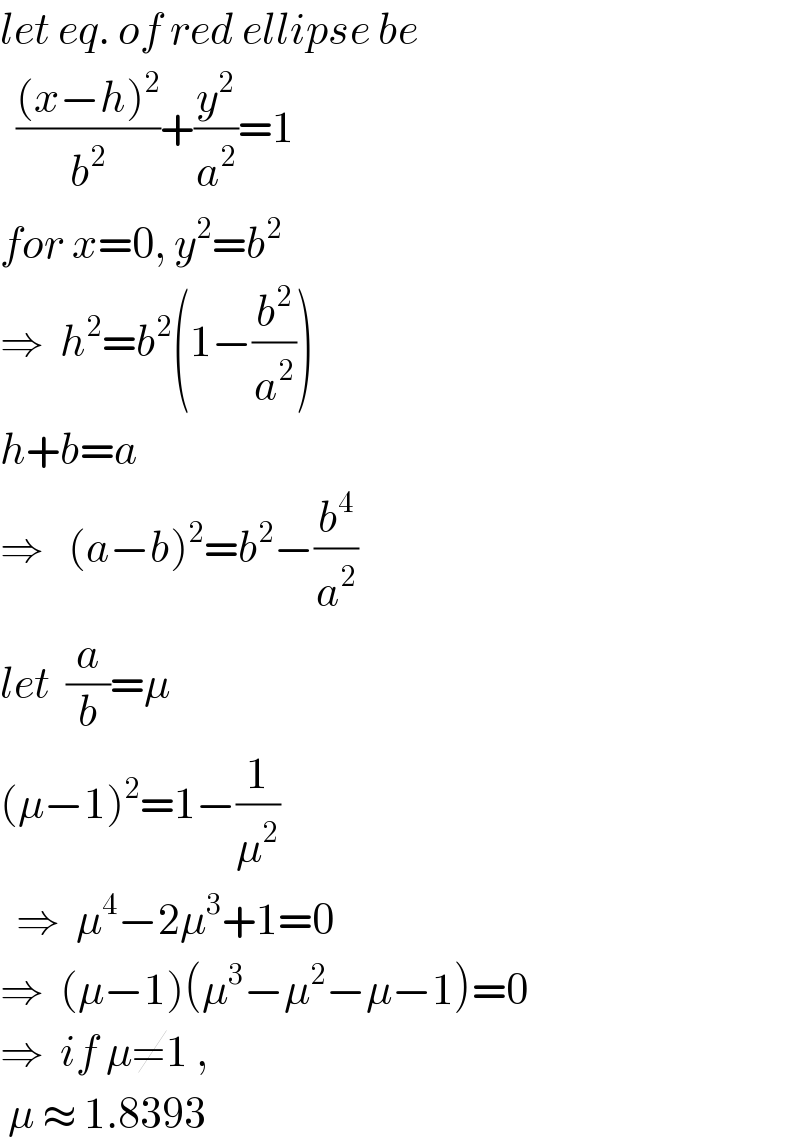
let eq. of red ellipse be (((x−h)^2 )/b^2 )+(y^2 /a^2 )=1 for x=0, y^2 =b^2 ⇒ h^2 =b^2 (1−(b^2 /a^2 )) h+b=a ⇒ (a−b)^2 =b^2 −(b^4 /a^2 ) let (a/b)=μ (μ−1)^2 =1−(1/μ^2 ) ⇒ μ^4 −2μ^3 +1=0 ⇒ (μ−1)(μ^3 −μ^2 −μ−1)=0 ⇒ if μ≠1 , μ ≈ 1.8393
$${let}\:{eq}.\:{of}\:{red}\:{ellipse}\:{be} \\ $$$$\:\:\frac{\left({x}−{h}\right)^{\mathrm{2}} }{{b}^{\mathrm{2}} }+\frac{{y}^{\mathrm{2}} }{{a}^{\mathrm{2}} }=\mathrm{1} \\ $$$${for}\:{x}=\mathrm{0},\:{y}^{\mathrm{2}} ={b}^{\mathrm{2}} \\ $$$$\Rightarrow\:\:{h}^{\mathrm{2}} ={b}^{\mathrm{2}} \left(\mathrm{1}−\frac{{b}^{\mathrm{2}} }{{a}^{\mathrm{2}} }\right) \\ $$$${h}+{b}={a} \\ $$$$\Rightarrow\:\:\:\left({a}−{b}\right)^{\mathrm{2}} ={b}^{\mathrm{2}} −\frac{{b}^{\mathrm{4}} }{{a}^{\mathrm{2}} } \\ $$$${let}\:\:\frac{{a}}{{b}}=\mu \\ $$$$\left(\mu−\mathrm{1}\right)^{\mathrm{2}} =\mathrm{1}−\frac{\mathrm{1}}{\mu^{\mathrm{2}} } \\ $$$$\:\:\Rightarrow\:\:\mu^{\mathrm{4}} −\mathrm{2}\mu^{\mathrm{3}} +\mathrm{1}=\mathrm{0} \\ $$$$\Rightarrow\:\:\left(\mu−\mathrm{1}\right)\left(\mu^{\mathrm{3}} −\mu^{\mathrm{2}} −\mu−\mathrm{1}\right)=\mathrm{0} \\ $$$$\Rightarrow\:\:{if}\:\mu\neq\mathrm{1}\:,\: \\ $$$$\:\mu\:\approx\:\mathrm{1}.\mathrm{8393} \\ $$
Commented by mr W last updated on 22/Apr/20
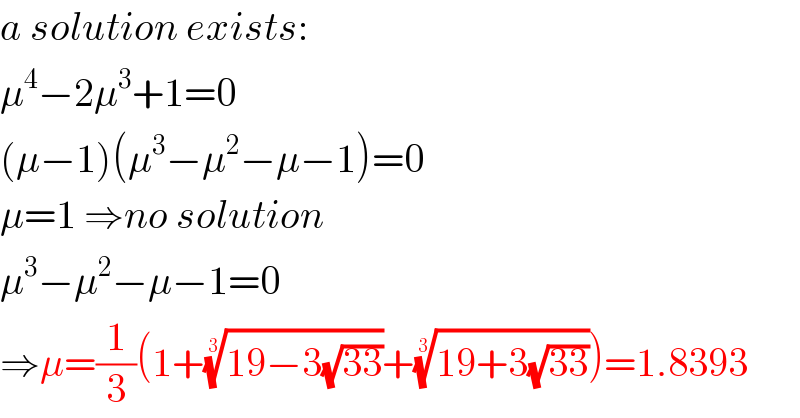
a solution exists: μ^4 −2μ^3 +1=0 (μ−1)(μ^3 −μ^2 −μ−1)=0 μ=1 ⇒no solution μ^3 −μ^2 −μ−1=0 ⇒μ=(1/3)(1+((19−3(√(33))))^(1/3) +((19+3(√(33))))^(1/3) )=1.8393
$${a}\:{solution}\:{exists}: \\ $$$$\mu^{\mathrm{4}} −\mathrm{2}\mu^{\mathrm{3}} +\mathrm{1}=\mathrm{0} \\ $$$$\left(\mu−\mathrm{1}\right)\left(\mu^{\mathrm{3}} −\mu^{\mathrm{2}} −\mu−\mathrm{1}\right)=\mathrm{0} \\ $$$$\mu=\mathrm{1}\:\Rightarrow{no}\:{solution} \\ $$$$\mu^{\mathrm{3}} −\mu^{\mathrm{2}} −\mu−\mathrm{1}=\mathrm{0} \\ $$$$\Rightarrow\mu=\frac{\mathrm{1}}{\mathrm{3}}\left(\mathrm{1}+\sqrt[{\mathrm{3}}]{\mathrm{19}−\mathrm{3}\sqrt{\mathrm{33}}}+\sqrt[{\mathrm{3}}]{\mathrm{19}+\mathrm{3}\sqrt{\mathrm{33}}}\right)=\mathrm{1}.\mathrm{8393} \\ $$
Commented by mr W last updated on 22/Apr/20
Commented by ajfour last updated on 22/Apr/20
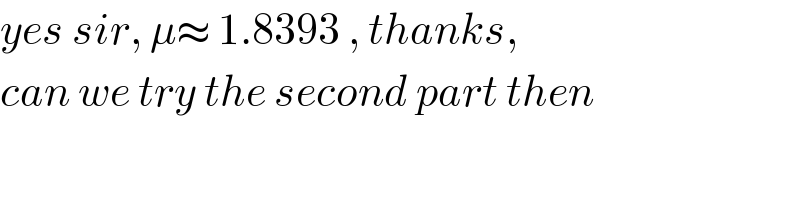
yes sir, μ≈ 1.8393 , thanks, can we try the second part then
$${yes}\:{sir},\:\mu\approx\:\mathrm{1}.\mathrm{8393}\:,\:{thanks}, \\ $$$${can}\:{we}\:{try}\:{the}\:{second}\:{part}\:{then} \\ $$
Answered by ajfour last updated on 22/Apr/20
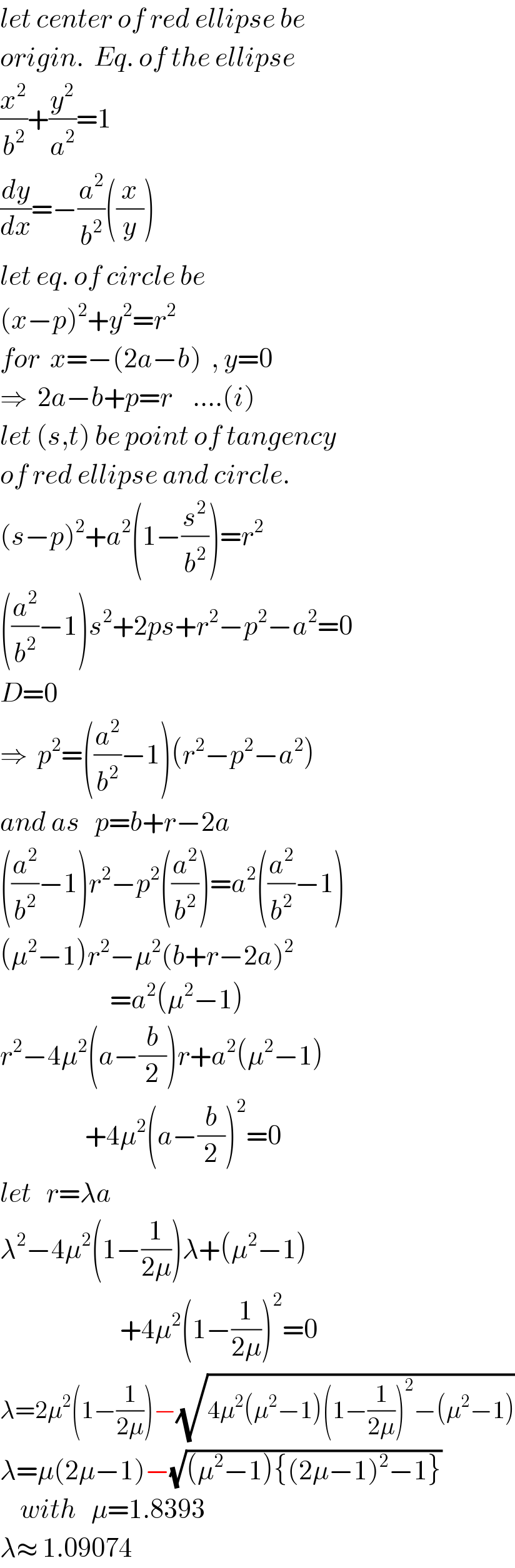
let center of red ellipse be origin. Eq. of the ellipse (x^2 /b^2 )+(y^2 /a^2 )=1 (dy/dx)=−(a^2 /b^2 )((x/y)) let eq. of circle be (x−p)^2 +y^2 =r^2 for x=−(2a−b) , y=0 ⇒ 2a−b+p=r ....(i) let (s,t) be point of tangency of red ellipse and circle. (s−p)^2 +a^2 (1−(s^2 /b^2 ))=r^2 ((a^2 /b^2 )−1)s^2 +2ps+r^2 −p^2 −a^2 =0 D=0 ⇒ p^2 =((a^2 /b^2 )−1)(r^2 −p^2 −a^2 ) and as p=b+r−2a ((a^2 /b^2 )−1)r^2 −p^2 ((a^2 /b^2 ))=a^2 ((a^2 /b^2 )−1) (μ^2 −1)r^2 −μ^2 (b+r−2a)^2 =a^2 (μ^2 −1) r^2 −4μ^2 (a−(b/2))r+a^2 (μ^2 −1) +4μ^2 (a−(b/2))^2 =0 let r=λa λ^2 −4μ^2 (1−(1/(2μ)))λ+(μ^2 −1) +4μ^2 (1−(1/(2μ)))^2 =0 λ=2μ^2 (1−(1/(2μ)))−(√(4μ^2 (μ^2 −1)(1−(1/(2μ)))^2 −(μ^2 −1))) λ=μ(2μ−1)−(√((μ^2 −1){(2μ−1)^2 −1})) with μ=1.8393 λ≈ 1.09074
$${let}\:{center}\:{of}\:{red}\:{ellipse}\:{be}\: \\ $$$${origin}.\:\:{Eq}.\:{of}\:{the}\:{ellipse} \\ $$$$\frac{{x}^{\mathrm{2}} }{{b}^{\mathrm{2}} }+\frac{{y}^{\mathrm{2}} }{{a}^{\mathrm{2}} }=\mathrm{1} \\ $$$$\frac{{dy}}{{dx}}=−\frac{{a}^{\mathrm{2}} }{{b}^{\mathrm{2}} }\left(\frac{{x}}{{y}}\right) \\ $$$${let}\:{eq}.\:{of}\:{circle}\:{be} \\ $$$$\left({x}−{p}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$${for}\:\:{x}=−\left(\mathrm{2}{a}−{b}\right)\:\:,\:{y}=\mathrm{0} \\ $$$$\Rightarrow\:\:\mathrm{2}{a}−{b}+{p}={r}\:\:\:\:….\left({i}\right) \\ $$$${let}\:\left({s},{t}\right)\:{be}\:{point}\:{of}\:{tangency} \\ $$$${of}\:{red}\:{ellipse}\:{and}\:{circle}. \\ $$$$\left({s}−{p}\right)^{\mathrm{2}} +{a}^{\mathrm{2}} \left(\mathrm{1}−\frac{{s}^{\mathrm{2}} }{{b}^{\mathrm{2}} }\right)={r}^{\mathrm{2}} \\ $$$$\left(\frac{{a}^{\mathrm{2}} }{{b}^{\mathrm{2}} }−\mathrm{1}\right){s}^{\mathrm{2}} +\mathrm{2}{ps}+{r}^{\mathrm{2}} −{p}^{\mathrm{2}} −{a}^{\mathrm{2}} =\mathrm{0} \\ $$$${D}=\mathrm{0} \\ $$$$\Rightarrow\:\:{p}^{\mathrm{2}} =\left(\frac{{a}^{\mathrm{2}} }{{b}^{\mathrm{2}} }−\mathrm{1}\right)\left({r}^{\mathrm{2}} −{p}^{\mathrm{2}} −{a}^{\mathrm{2}} \right) \\ $$$${and}\:{as}\:\:\:{p}={b}+{r}−\mathrm{2}{a} \\ $$$$\left(\frac{{a}^{\mathrm{2}} }{{b}^{\mathrm{2}} }−\mathrm{1}\right){r}^{\mathrm{2}} −{p}^{\mathrm{2}} \left(\frac{{a}^{\mathrm{2}} }{{b}^{\mathrm{2}} }\right)={a}^{\mathrm{2}} \left(\frac{{a}^{\mathrm{2}} }{{b}^{\mathrm{2}} }−\mathrm{1}\right) \\ $$$$\left(\mu^{\mathrm{2}} −\mathrm{1}\right){r}^{\mathrm{2}} −\mu^{\mathrm{2}} \left({b}+{r}−\mathrm{2}{a}\right)^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:={a}^{\mathrm{2}} \left(\mu^{\mathrm{2}} −\mathrm{1}\right) \\ $$$${r}^{\mathrm{2}} −\mathrm{4}\mu^{\mathrm{2}} \left({a}−\frac{{b}}{\mathrm{2}}\right){r}+{a}^{\mathrm{2}} \left(\mu^{\mathrm{2}} −\mathrm{1}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+\mathrm{4}\mu^{\mathrm{2}} \left({a}−\frac{{b}}{\mathrm{2}}\right)^{\mathrm{2}} =\mathrm{0} \\ $$$${let}\:\:\:{r}=\lambda{a} \\ $$$$\lambda^{\mathrm{2}} −\mathrm{4}\mu^{\mathrm{2}} \left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}\mu}\right)\lambda+\left(\mu^{\mathrm{2}} −\mathrm{1}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+\mathrm{4}\mu^{\mathrm{2}} \left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}\mu}\right)^{\mathrm{2}} =\mathrm{0} \\ $$$$\lambda=\mathrm{2}\mu^{\mathrm{2}} \left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}\mu}\right)−\sqrt{\mathrm{4}\mu^{\mathrm{2}} \left(\mu^{\mathrm{2}} −\mathrm{1}\right)\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}\mu}\right)^{\mathrm{2}} −\left(\mu^{\mathrm{2}} −\mathrm{1}\right)} \\ $$$$\lambda=\mu\left(\mathrm{2}\mu−\mathrm{1}\right)−\sqrt{\left(\mu^{\mathrm{2}} −\mathrm{1}\right)\left\{\left(\mathrm{2}\mu−\mathrm{1}\right)^{\mathrm{2}} −\mathrm{1}\right\}} \\ $$$$\:\:\:\:{with}\:\:\:\mu=\mathrm{1}.\mathrm{8393} \\ $$$$\lambda\approx\:\mathrm{1}.\mathrm{09074} \\ $$
Commented by mr W last updated on 22/Apr/20
very nice solution sir! exact!
$${very}\:{nice}\:{solution}\:{sir}!\:{exact}! \\ $$
Commented by ajfour last updated on 22/Apr/20

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *